I'm going to conduct an investigation into the dampening of a spring.
Essentially, what specific factors could be investigated? Currently I'm planning to investigate the effect of changing the mass on the end of the spring, the effect of using different spring constants, and the effect of changing the initial extension of the spring on the amount of dampening.
and welcome to the site. I suppose that you have to do this as some kind of homework so I would not try to invent new things and just look what mathematical description(s) of such a system exists. The governing equations will have some constants in it and it would be already a nice thing to look if the given framework can explain the dynamics and if you can determine those constants.
Before we start, you can be assured that the harmonic oscillator is most likely the physical model with the highest impact on life in general - applications from molecule spectra to airplane design.
Wikipedia has a really nice collection of articles for these kind of things. There, we can see that a damped harmonic oscillator can be described by the equation of motion
$$m\ddot{x} + c\dot{x} = -k x$$
where now $x$ is the position of the mass depending on time, dots denote derivative(s) with respect to it, $k$ is the spring "constant" and $c$ is the damping coefficient. All you have to do is to solve this equation by an exponential ansatz of the form $$x(t) = x_0 e^{\lambda t}$$ and discuss the several cases as given in the mentioned article
The motion in the underdamped case will look like an exponentially decaying oscillation $$x(t) = x_0 e^{-\frac{c}{2m}}\cos (\omega t + \phi_0)\,:$$
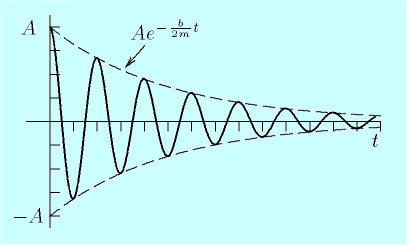
The picture was taken from the very good spanish wikipedia site concerning the topic, $c \equiv b$.
For a damped oscillation, you will have to determine two constants, $k$ and $c$. They are linked to the angular frequency of the system by $$\omega = \sqrt{\frac{k}{m}-\left( \frac{c}{2m}\right) ^2}\, .$$
Now, can you tell me, with respect to the given form of the movement, how to measure the constants just with a stopwatch and a ruler?
After you figured everything out, you can go into a lot of different directions like how the spring constant depends on $m$ (nonlinear motion), aperiodicity, Fourier space (Lorentzian) etc.
Sincerely
Is this a real spring? If it is, then damping is affected by a) The number of active coils (mass that is in motion), b) The cross section of the wire, c) Any rubbing or clashing between the coils, and d) the rate at which loads are applied on the coil (frequency dependency).
I suggest putting strain gauges on each 1/4 turn and hitting it with a hammer. You will see the response of the spring clearly and how the dynamics of the coils really affect the overall behavior.
I suggest find a good engineering book an spring design and analysis and you will be amazed at the complexity that springs exhibit in real life.
