3
$\begingroup$

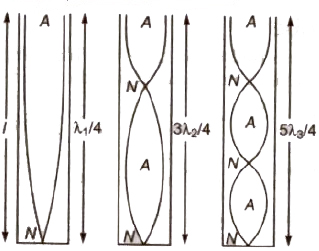
Its seems really clear to me how a guitar string vibrates. I can send a wave down a jump-rope by wiggling it the right way. The two fixed ends of the guitar string reflect this wave back onto the string, forcing it to vibrate at frequencies that involve the whole length of the string or an even division of it (the harmonic series). It makes sense that a guitarist can only find harmonics at the even division points (1/2 the string, 1/3 of the string, 1/4 and so on). This produces a series of ascending pitches that are related by all being natural number multiples of the frequency produced by the whole length of the same string.

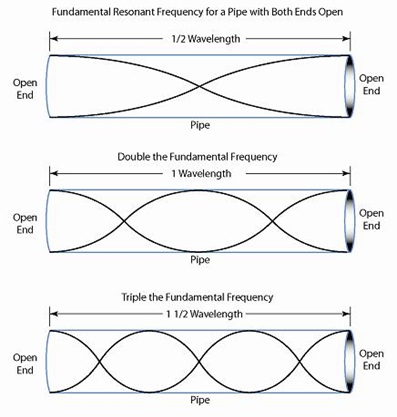
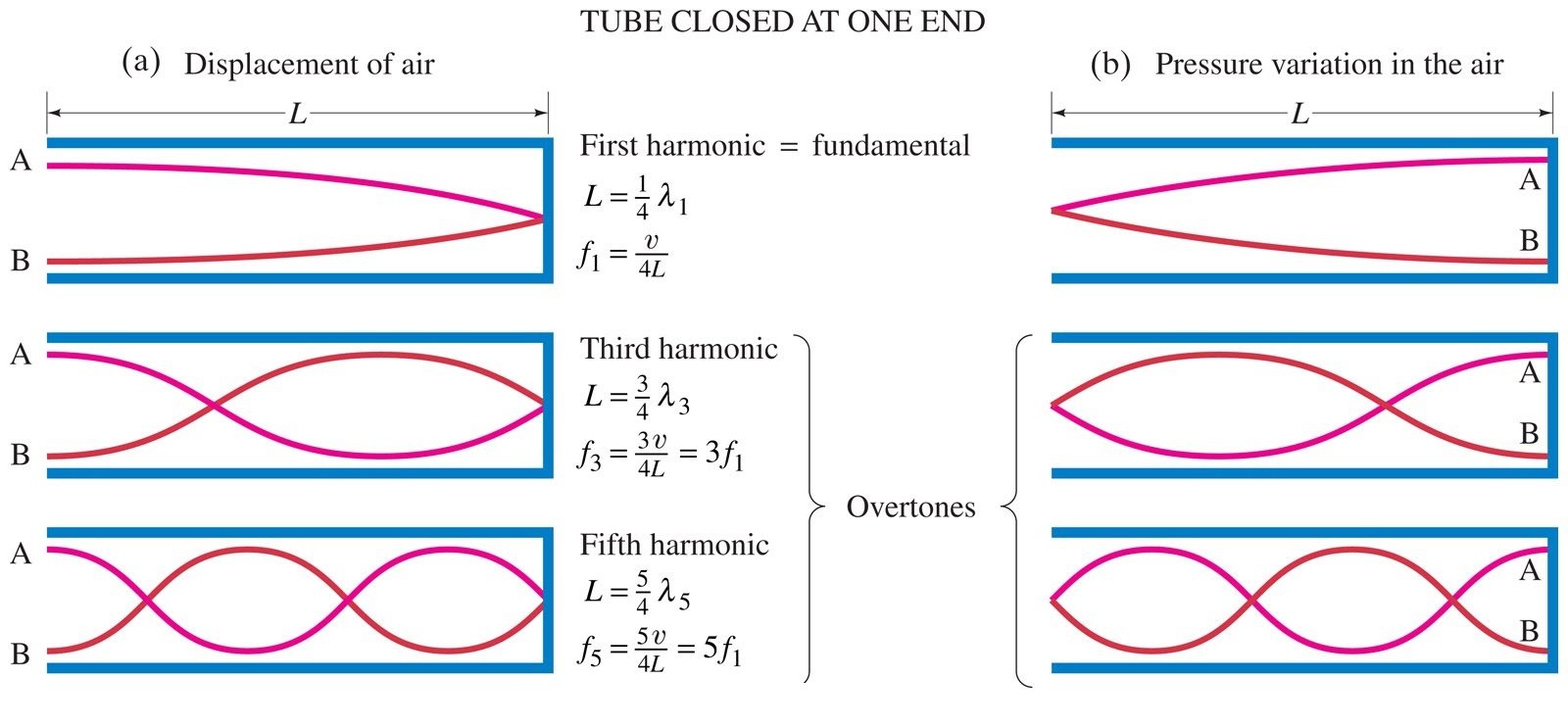
You can hear this same overtone series produced by brass instruments as the choice of frequencies (notes) that are playable for each given length of metal tubing (The seven different positions on a trombone, for example). The open, full length, guitar string is analogous to the brass pedal note, the lowest note possible on a brass instrument for a given length of tubing. Changing only the frequency of the lip buzz, not the tube length, other notes are "playable". Their pitches correspond to the harmonics played on guitar by tapping approximately at the 12th, 5th, 4th, 3rd frets (and more). All these same notes are available to brass players but notes in between can't be sounded at all, or very unclearly at best.

I see this correlation, but I have trouble imagining how things work with a vibrating column of air to produce a similar phenomenon. A vibrating string is so visual. What is the best analogy to help visualize how air vibrates in a brass instrument?

Improve this question
$\endgroup$
3
  • $\begingroup$ It's interesting that I find the string case more confusing to visualize, because the idea of sound waves being longitudinal is what I find intuitive about how they propagate. In that sense I think the brass case is more straightforward to imagine, quite literally... $\endgroup$
    – Amit
    Commented Nov 18 at 23:25
  • $\begingroup$ Try searching YouTube for "Kundt's Tube." $\endgroup$
    – Solomon Slow
    Commented Nov 19 at 4:23
  • $\begingroup$ @Amit just the opposite here: waves on a water surface and in a string/rope are transversal and literally visible... IMHO, what seems to pose the difficulty with a tube is the reflection at the open end. $\endgroup$
    – Roger V.
    Commented Nov 19 at 9:00

2 Answers 2

Reset to default
3
$\begingroup$

A standing wave in a string ca be viewed as a superposition of the wave reflected by the points where the string is clamped: we pluck the string, the waves propagate towards the ends of the spring, get reflected and interfere with the already existing vibration. Those that interfere constructively survive and give us the tone - the length of these surviving waves is an integer fraction of the string length.

Same happens with the pressure wave in a pipe: it is (partially) reflected at the opening of the pipe, and the reflected wave interfering with the pressure oscillations gives the tone.

The figure below is taken from another SE post, which is worth consulting for more details:
enter image description here

Here is another related thread:
enter image description here

Note the difference with a guitar: the reflection does not necessarily correspond to a node: it is different at the closed and the open ends of the pipe (yet another Physics SE thread):
enter image description here

See also this video.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So are the nodes the point of maximum air pressure, the antinodes the minimum pressure, and the antinode is attracted to the open end because it most closely approaches atmospheric pressure there, which is generally less? The varying tube air pressure and atmospheric pressure are able to equalize at the bell? $\endgroup$
    – SteveV
    Commented Nov 19 at 19:19
  • $\begingroup$ Also, could someone explain why closed pipe theory says the frequencies produced are $f$, $3f$, $5f$..., but a brass instrument produces the full harmonic series with even multiples of $f$ included. $\endgroup$
    – SteveV
    Commented Nov 19 at 22:53
2
$\begingroup$

Here's how:

a long trombone tube filled with air is like a squishy, compressible spring. The slug of air contained in the bell of the trombone is like a chunk of mass. that mass can be set into motion so it bounces back and forth on the springy air at the fundamental resonance or harmonics thereof, by feeding lip vibrations into the head end of the tube. This system acts just like a mass hanging from the end of a soft spring and bouncing up and down.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.