Beta particles in Rutherford experiment

Question

What happens if beta particles (electrons) are used in Rutherford gold foil experiment instead of alpha particles? Is there any possibility for the beta particles to be repelled by the existing electron cloud?

Answer 1

We got 4 up votes and comment is the answer with 3 up votes?

There are 2 questions:

1. What happens if you do the Rutherford Experiment with $\beta$ particles:

Answer: What happens if you make a Caesar salad with no anchovies but add chicken? Well, you didn't make a Caesar salad. [You aren't doing The Rutherford Experiment].

2. Do $\beta$'s scatter. Yes, beta particles scatter.

All experimental physicists should be familiar with the Bethe-Bloch equations (https://en.wikipedia.org/wiki/Bethe_formula), though that link is not as good as it used to be.

Use the Particle Data Group, they have complied everything you need to know. When discussing how $\alpha$ and $\beta$ particles go through matter...I mean there is just so much going on vs energy, matter type, radiation type, and so on: https://pdg.lbl.gov/2021/reviews/rpp2020-rev-passage-particles-matter.pdf is a must read.

Now regarding my salad comment, I may have been a but snarky, since wikipedia says:

Rutherford published a landmark paper in 1911 titled "The Scattering of α and β Particles by Matter and the Structure of the Atom"

So a proper answer would be to follow that up.

But: tldr, the point of the alpha particle is its mass. It simply cannot backscatter from a charge of $+79e$ distributed over an area of a square Bohr radius.

Gold is big, 146 nm, so the areal charge density in the plumb pudding model is:

$$ \sigma_{plum} \approx 189{\rm C/m^2} $$

Now google's AI is telling me a gold nucleus is 15 fm, which seems a but large, but lets do that. It's 10,000 times smaller than an atom, which boosts the areal density by 8 orders of magnitude.

Note: I did that charge thing thinking about the electron. Electrons and photons (see the PDG link), they care about the square of the electric field for interacting, and if that $79e$ is distributed over a 30 nm atom, it is much much less than the field near a gold nucleus. Let's work it out:

$$ E \approx \frac 1 {4\pi\epsilon_n} \frac{ Ze}{R^2} \approx 500\,{\rm EC} $$

it's huge, and squared.

Now at beta decay energies, the betas are interacting (long range) with the atomic electrons and not back scattering. At higher energies, you get into bremsstrahlung on the nucleus--just read about electromagnetic showers/calorimeters, they are a key component of every high energy physics experiment.

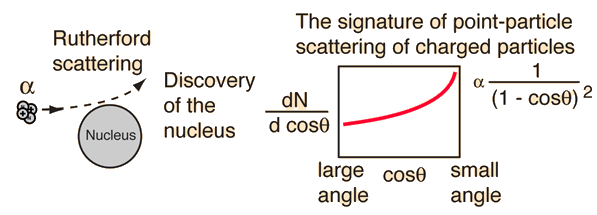
Back to Rutherford: He came up with the formula for non-relativistic point-charge Coulomb scattering:

$$ \frac{d\sigma}{d\cos\theta} = \frac{\pi} 2 Z^2_{\alpha}Z^2_{\rm Au} \Big[ \frac{\hbar c}{E-M} \Big]^2 \frac 1 {(1-\cos\theta)^2} $$

which look likes:

The key here is that is for point particles. If $Z_{\rm Au}$ is distributed according to the plum pudding model, the scattering will be weaker (compare with "Elastic Form factor of the proton").

Note that the length scale is set by the term in square brackets, one over the kinetic energy.

All nuclear physicists have the following memorized:

$$ \hbar c = 197\,{\rm MeV\cdot fm} $$

where $fm$ is a fermi, not a femtometer. No one says femtometer. No one.

If someone says, "Yeah, I'm a bit of nuclear physicist myself." Just ask, "Ok, what is $\hbar c$?", and if the answer is not instant, and "one hundred a ninety seven Fermi", they are sus. (Note: $0.2 {\rm GeV\cdot fm}$ is OK, but it means they might be a particle physicist).

Caveat: I am not sure why we don't need to consider the string force here. I think that kicks in at higher energy, say a kinetic energy on the order of pion mass (130 MeV), which given your new knowledge of $\hbar c$ means the length scale of the interaction is about a fermi, which is ofc, the size of the nucleus (not a coincidence). At the 5 MeV available in typical alpha decays, that alpha is not getting close to the gold nucleus.