3
$\begingroup$

When a roots blower runs, it has some oscillation in the flow rate (and consequently pressure) due to lobe geometry. The pattern occurs $4$ times per rotation, so for a direct drive $1800 \ \text{rpm}$ motor, the oscillation frequency is $120 \ \text{Hz}$.

In my application, I need very constant vacuum pressure ($<1\%$ of the absolute operating pressure), requiring very smooth flow. Adding a very large volume between the pump and the user and dampening would help, but it would be large and expensive to fabricate. The user needs around $1000 \ \text{Pa}$, so the volume required and pressure differential allowed. A mechanical regulator of any sort is impractical. Also, since this is near full vacuum, it makes large vessels very expensive to fabricate.

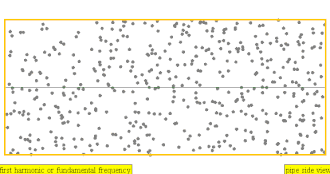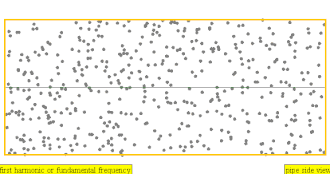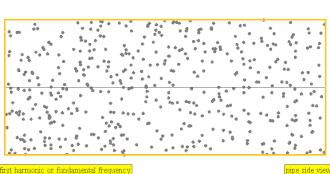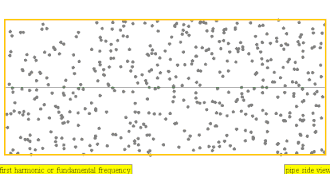
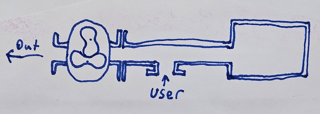
I am considering the following geometry (hand sketch below). The pump feeds directly into a Helmholtz resonating volume, and the user connects at $90^\circ$ at the standing wave pressure node (the center of the image below). Some assumptions here since it would be in a system with flow, but just looking at the oscillating component. When the velocity in the pipe is the highest back and forth, the pressure change should be the lowest (velocities will not be high enough or long enough to consider Bernoulli). Then, in theory, the user sees constant pressure (ignoring geometry and flow considerations of the user inlet).

Image from Acoustic Resonance Wiki:

enter image description here

I did a rough Helmholtz calculation and came to $6 \ \text{litres}$, which is great from a fabrication standpoint but makes me hesitate since it would not be much more volume than the pipe. Alternatively, I could just use a $3$-meter ($350 \ \text{m s}^{-1}/120 \ \text{Hz}$, where $350 \ \text{m/s}$ is the speed of sound) length of pipe capped at the end.

Are my assumptions correct? Would one of these work better than another? Better ideas? Any similar existing applications that you are aware of? All feedback is appreciated. Thanks!

enter image description here

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Is it possible to run TWO identical blowers and connect their drives to be 180 degrees out of phase? There will likely be some oscillations due to the mechanical connection though. $\endgroup$
    – Mariano G
    Commented Oct 7 at 20:19
  • $\begingroup$ @MarianoG, I had thought of this too, but yeah difficult to get the timing correct and there is definitely no guarantee the pressure fluctuation from the pump is sinusoidal so there would probably be harmonics. Right now it doesnt look the the risk is worth the reward, but might visit the idea again in the future. $\endgroup$
    – ericnutsch
    Commented Oct 7 at 21:52

2 Answers 2

Reset to default
4
$\begingroup$

Your assumptions are solid. Using a Helmholtz resonator to match the 120 Hz oscillation frequency should help stabilize the pressure. Connecting the user at the pressure node (where pressure fluctuations are minimized) is correct, and the 6-liter volume seems reasonable. Though not much larger than the pipe, it's the resonance effect that matters, not just volume. The 3-meter capped pipe could also work as a quarter-wave resonator but would be bulkier. The Helmholtz resonator is likely more space-efficient and effective for this application.

Improve this answer
$\endgroup$
1
  • $\begingroup$ The pipe is actually easier in this application because it is not mobile and I can just purchase the pipe without the need to fabricate a vessel. My hesitation with the pipe though is that it may be more susceptible to secondary harmonics. Or does the Helmholtz have the same potential? Thoughts on reducing harmonics? Thanks! $\endgroup$
    – ericnutsch
    Commented Oct 7 at 21:57
2
$\begingroup$

Your concern about secondary harmonics is valid, particularly with the pipe approach. Pipes can resonate at not just the fundamental frequency (120 Hz in this case), but also at higher harmonics (multiples of the fundamental), which could introduce unwanted pressure fluctuations. Helmholtz resonators, in contrast, are primarily designed to resonate at a single frequency, so they tend to suppress higher harmonics more effectively than pipes. Helmholtz resonators are more focused on a single frequency, but they aren't immune to harmonics if not well-tuned. If harmonics are a major concern, the Helmholtz resonator might still be more stable.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Nice answer (+1) How many harmonics will likely exist above the fundamental frequency (120 Hz), is there a maximum theoretical number? Does each higher harmonics decay exponentially in amplitude until the strength becomes unmeasurable in the very high harmonics? $\endgroup$
    – James
    Commented Oct 8 at 0:53
  • 1
    $\begingroup$ There is no theoretical limit to the number of harmonics above the fundamental (120 Hz), but practically, higher harmonics decay in amplitude. Typically, the strength of each harmonic decreases, often exponentially, due to damping and energy loss. At some point, the amplitude becomes so small it's negligible and unmeasurable. The exact rate of decay depends on the system's material, geometry, and damping properties. $\endgroup$
    – user436315
    Commented Oct 8 at 20:42
  • $\begingroup$ thank you very much. If the fundamental frequency is $f_1$, then the frequency of the third harmonic would be $f_3 = 3 f_1$, correct? I have come across a formula like $\frac{1}{n^2}$ being the decay of amplitude for odd harmonics (there supposedly exists no even harmonics), so the third harmonic will be $\frac{1}{9}$ the amplitude of the fundamental frequency, does that formula look plausible in your view? $\endgroup$
    – James
    Commented Oct 8 at 23:56
  • 1
    $\begingroup$ Yes, the third harmonic would be 3 times the fundamental frequency. The formula you mentioned for amplitude decay looks plausible for certain systems where only odd harmonics exist. It suggests that higher harmonics get weaker as their number increases, with the third harmonic being much smaller than the fundamental. This general pattern is common in many resonance systems. $\endgroup$
    – user436315
    Commented Oct 10 at 1:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.