Why do we say the extraordinary ray does not follow Snell's law, even though we write the refractive index of extraordinary ray as $\dfrac{\sin i}{\sin r}$? The only thing that happens is the refractive index of the extraordinary ray is variable according to the direction in which it is propagating, but if we can write the refractive index of the extraordinary ray as $\dfrac{\sin i}{\sin r}$, it means it is following Snell's law. When did Snell say that refractive index is needed to be constant for a given material?
-
1$\begingroup$ In a birefringent crystal the extraordinary ray can experience spatial walk-off at normal incidence, and separate from the ordinary ray. This is not predicted by Snell's law. $\endgroup$– fulisCommented Oct 7 at 14:08
-
1$\begingroup$ what do you mean by "spatial walk off at normal incidence" ? Is there any pictorial representation for that? $\endgroup$– Assassins HunterCommented Oct 7 at 14:56
-
1$\begingroup$ look into: Poyinting vector in birefringent materials $\endgroup$– José AndradeCommented Oct 20 at 13:03
1 Answer
In an anisotropic medium, you've learned that the light ray propagates in the direction of the wave vector. In an anisotropic medium, you have to be careful about the notions of light ray and wave vector. In general, the Snell-Descartes's law do not apply to light rays but to wave vectors in an anisotropic meidum.
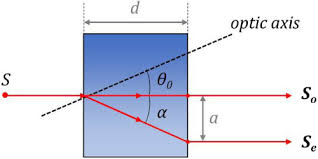
But for an anistropic medium, the permittivity $\epsilon$ isn't a scalar anymore, so there's a difference between the direction of the phase $\mathbf{k}$ (wave vector) and the direction of the energy $\mathbf{S}$ (Poyting's vector). This concerns only the extraordinary ray, its name comes from the fact that it does not follow the Snell-Descarte's law under certain circumstances.
If the medium was isotropic, the ray would have passed through it without any deviation following the Snell-Descartes's law. Just like the ordinary ray (because it is ordinary to follow this law). The angle between the two ray is called the walk-off angle. This angle isn't explained by the laws of refraction formulated by Snell and Descartes. $\textbf{Note that this ray splitting is due to the fact that the optic axis is not parallel or perpendicular to the face of the plate.}$
-
1$\begingroup$ The statement "In general, the Snell-Descartes's law do not apply to light rays but to wave vectors in an anisotropic meidum" what do you mean by this statement i did not understand.@MauvaiseFoi $\endgroup$ Commented Nov 9 at 18:39
-
$\begingroup$ Do you have any recommendation from where i can study the topic of Birefringence in detail and good manner? Any books, resources or videos. Please tell @MauvaiseFoi $\endgroup$ Commented Nov 9 at 19:43
-
$\begingroup$ Optics by E. Hecht (chapter 8), Polarization of light by S. Huard, Polarized light : production and use by W. Shurcliff. Look at the first one to begin with $\endgroup$ Commented Nov 9 at 19:50
-
$\begingroup$ k and E(electric field ) should be always perpendicular how they can be collinear and when you say "Light rays follow the Poynting's vector and Snell's laws apply to the wave vector". Is direction of wave vector is not the direction of the light ray propagation?@MauvaiseFoi $\endgroup$ Commented Nov 9 at 19:55
-
$\begingroup$ Oh, yes exactly.. my bad. In a anisotropic medium $\mathbf{k}$ and $\mathbf{E}$ aren't perpendicular anymore except in certain cases (like for $\theta_0=\pi/2$ or $0$ on the above figure). The direction of the light ray is the Poynting's vector. Of course, for an isotropic medium, $\mathbf{k}$ and $\mathbf{S}$ are colinear so the direction of the ray is the same as the direction of the wave vector. Everything is much better explained by Hecht in his book ! $\endgroup$ Commented Nov 9 at 20:00