7
$\begingroup$

A textbook I'm reading says that in a cylindrical beaker, the center of a meniscus must be completely flat (having infinite radius of curvature) because otherwise "there is no way the surface tension on the molecules near the center can be balanced vertically". Can't a pressure difference balance it?

The text is Wang and Ricardo's Competitive Physics. Here is the excerpt:

"In fact, the center of the meniscus must be completely flat — else there is no chance for the surface tension on the molecules near the center to be balanced in the vertical direction. This flatness implies that the pressure in the liquid, directly below the meniscus, is the atmospheric pressure $p_0$ by the Young-Laplace equation (with infinite radii of curvature)."

The explanation seems circular to me.

The reason I ask this question is because the author is able to derive this result $$\Large{ H = \sqrt{\frac{2\gamma(1-\sin\theta)}{\rho g}}}$$ Where $H$ is the meniscus height and $\theta$ is the contact angle.

He does this by balancing forces on the meniscus, and taking the pressure at the bottom of the meniscus as $p_0$ from the above assumption.

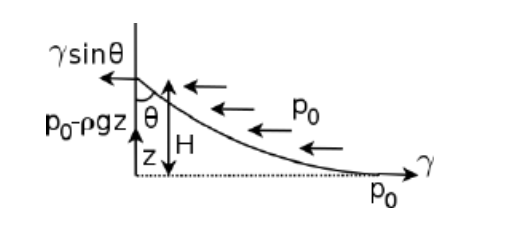
Here's the free-body diagram and the force equation (only horizontal forces are represented): enter image description here

$$\Large{p_0H-\frac{\rho gH^2}{2}-p_0H+\gamma-\gamma\sin\theta}=0$$

Improve this question
$\endgroup$
9
  • 1
    $\begingroup$ I'm not sure that is correct. Consider the beaker to have a small diameter. What is the text you are reading? $\endgroup$
    – Math Keeps Me Busy
    Commented Sep 15 at 14:29
  • $\begingroup$ I would buy “approximately flat” but not “completely flat.” What is the exact wording and source? $\endgroup$
    – Chemomechanics
    Commented Sep 15 at 15:42
  • $\begingroup$ Added it to the question $\endgroup$
    – Marc Carlsan
    Commented Sep 15 at 16:46
  • 2
    $\begingroup$ not flat at all in general. The smaller the diameter, the less flat. Maybe the right word is "horizontal" $\endgroup$
    – basics
    Commented Sep 15 at 16:47
  • $\begingroup$ This is wrong if meant in general. To have the surface flat (infinite radius of curvature), the pressure difference has to be zero. But it often isn't zero - e.g. water column in glass capillary rises above the water level in the container, and in equilibrium state, where it rises to height $h$, pressure of water at that higher height has to be lower than atmospheric pressure by $\rho gh$ , thus the water surface in the capillary is curved, with finite radius of curvature, linked to this difference of pressure. $\endgroup$
    – Ján Lalinský
    Commented Sep 16 at 12:11

3 Answers 3

Reset to default
6
$\begingroup$

I am not sure that explanation really is right.

If the pressure at the surface was higher at one spot than another in the middle of a cylinder, the high pressure spot would push the surface up more than the low pressure spot. The surface would bulge up there. The bulge would grow until the combination of extra weight in the bulge and force from surface tension pulling along the tilted surface pulling down balanced the upward force from the extra pressure.

If you did have such a bulge, water would flow until it flattened out.

You do have a curve at the edge of the cylinder. Water is sticky. Molecules of water attract each other. Many substances like glass also attract water. If they attract water more than they attract air, water will climb the sides until the extra weight pulls it down as hard as the attractive force pulls it up.

Improve this answer
$\endgroup$
3
$\begingroup$

EDIT. After the edit in the question, I'll add an edit here to my previous answer. This sentence

and taking the pressure at the bottom of the meniscus as p0 from the above assumption.

is wrong! if you think at the fluid in a small capillary pipe, since you're not considering the height in the capillarity pipe and the related pressure jump.

This condition is the condition you find in an open vessel, when you're only focusing on the region close to the wall. In the limit of large vessels, there is no capillarity effect far from the wall, no pressure jump across the surface and thus no surface curvature.

Original answer. The "center of the meniscus" is not flat at all in general. The smaller the diameter, the less flat. The explanation is wrong, being the Young-Laplace equation a relation between the pressure jump and the curvature of the surface.

As an example, in the experiment of water in a thin capillary, pressure jump across the free surface is proportional to the height of the water column, $\Delta P = \rho g \Delta h$, approximately constant (except for small height difference across the section of the capillary) and thus the curvature of the free surface is approximately constant, $k \propto \Delta P / \gamma$

Maybe the right word is "horizontal".

Please, do not confuse first-order (horizontal) and second-order (flat) derivative. Words matter!

Improve this answer
$\endgroup$
7
  • $\begingroup$ No, the author says that its flat (infinite radius of curvature). The author is able to derive a result with this assumption which (I suppose) is correct (the meniscus height is sqrt(2γ(1 − sin θ)/ρg) $\endgroup$
    – Marc Carlsan
    Commented Sep 15 at 16:52
  • $\begingroup$ IMHO, the author is wrong. But I'll be glad to learn something new if you share the derivation of this result by the author $\endgroup$
    – basics
    Commented Sep 15 at 16:56
  • $\begingroup$ the height of the meniscus only depends on $\gamma$, $\theta$ (angle of contact), $\rho g$, but this doesn't prove anything about the curvature (local quantity) of the surface. The height of the column can be related to the pressure jump across the surface using Stevino's law. You need Young-Laplace equation to say something about curvature, and this equation exactly says that curvature is proportional to the pressure jump across the free surface (not zero, and thus the surface can't be flat) $\endgroup$
    – basics
    Commented Sep 15 at 17:02
  • $\begingroup$ It seems like this textbook’s approach would yield only the maximum possible meniscus height in this context. $\endgroup$
    – Chemomechanics
    Commented Sep 15 at 17:07
  • $\begingroup$ from what I read here, it looks like a naive approach, with logical jumps and mistakes. Maybe a high school textbook? $\endgroup$
    – basics
    Commented Sep 15 at 17:12
3
$\begingroup$

This is why good editors for texts are important. The phrase, "center of the meniscus must be completely flat — else there is no chance for the surface tension on the molecules near the center to be balanced," misplaces "flat" for "horizontal" and includes a superfluous clause "else there is no chance" and loses no useful information if "on the molecules" is omitted.

This is the more accurate and shorter version, "the center of the meniscus must be completely horizontal for the surface tension near the center to be balanced in the vertical direction."

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.