Forces accelerating a tire

Question

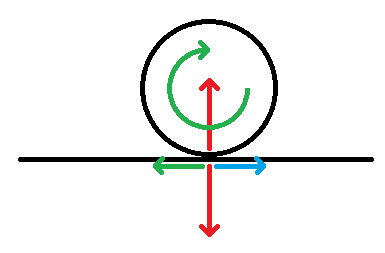
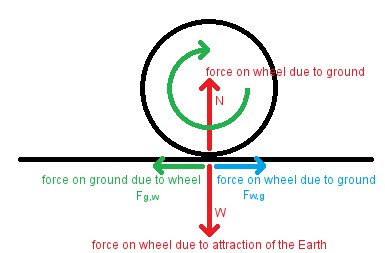
I'm attempting to write a little vehicle simulator and I'm working on calculating vehicle linear acceleration and tire angular acceleration. I'm trying to add in tire slipping and I'm having trouble deriving the solution. I realized this is because I don't have a complete understanding of how all the forces involved work. Consider this simplified image:

The tire is receiving torque from the engine and brakes (the green rotating arrow). This can be expressed as a linear force by dividing by the tire radius (the green horizontal arrow). Combine this force with the force of the tire and vehicle from gravity (the red arrow pointing down). Intuitively, this combined force is "applied against the ground". What does this mean exactly? I think you break it up into two components, vertical and horizontal, which happen to just correspond to the force from gravity and the linear force calculated from torque, since we're on flat ground. The vertical force is fully opposed (the red arrow pointing up) and the horizontal force "stays as torque" (maybe? I'm not sure how to correctly think about this). In a frictionless situation, the horizontal force gets no opposing force and causes only angular acceleration. When you add in friction, you get an opposing linear force at the point of contact (I think?), equal in magnitude to the horizontal force in the case of static friction and of a fixed magnitude in the case of kinetic friction (the blue horizontal arrow).

Here's where I'm confused: intuitively, the green line "stays as torque" and causes angular acceleration and the blue arrow "stays as linear force" and causes linear acceleration. But I'm pretty sure that intuition is meaningless/wrong and I just don't understand the concepts well. When are we allowed to go back and forth between torque and force? Why am I allowed to treat the green torque arrow as a force arrow for the purposes of friction (or: am I even allowed to do this?)? Why don't the green and blue arrows simply cancel out, resulting in no acceleration/motion?

Answer 1

I suggest a simple model to cover the no slipping and the slipping situation.

Why don't the green and blue arrows simply cancel out, resulting in no acceleration/motion?
Because the forces act on different objects.

The two red forces are equal in magnitude and opposite in direction and both act on the wheel.
The blue and green forces are also equal in magnitude and opposite in direction but act on different object and are a Newton third law pair of forces (action/reaction).
The green and blue forces are frictional forces and the friction can either be static, ie no slipping, or dynamic, ie slipping.
The static frictional force can be any value as given by the equation $0\le F_{\rm w,g,static}\le \mu_{\rm static}\,N$, where $\mu_{\rm static}$ is the coefficient of static friction.
The torque applied to the wheel would then be $F_{\rm w,g,static}\,r$ where $r$ is the radius of the wheel with the force $F_{\rm w,g,static}$ providing the acceleration of the wheel.
So the maximum torque which can be applied to the wheel with no slipping occurring is $\mu_{\rm static}\,N\,r$.

If a larger torque is applied then slipping will occur and the frictional force, $\mu_{\rm dynamic}\,N$ drops because the coefficient of dynamic friction is less than the coefficient of static friction and also note that it has a constant value.

Answer 2

Just consider the forces on the wheel - ignore the forces exerted by the wheel on the ground. The four forces on the wheel are:

(a) its weight - your downwards red arrow

(b) the normal force from the ground - your upwards red arrow

(c) torque from the engine - your circular green arrow

(d) friction from the ground - your blue arrow

So you do not need your horizontal green arrow - this is just confusing you.

(a) and (b) are equal and opposite forces so they net to zero.

If the wheel is not accelerating then (c) and (d) must represent equal and opposite torques on the wheel. In other words, if the torque from the engine is $T$, friction is $F$ and the radius of the wheel is $r$ then for a non-accelerating wheel we have

$T = Fr$