1
$\begingroup$

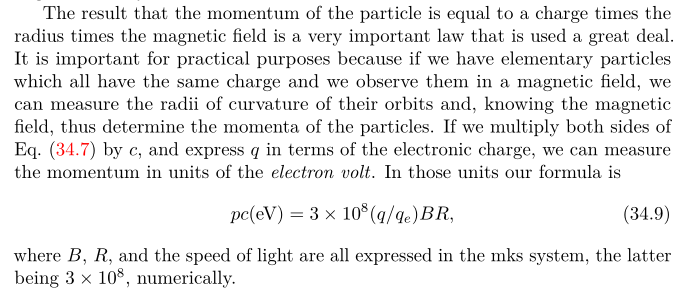
Pictures below are from 34-3 of Feynman's Lectures on physics. I can't understand the red line.

The $p$ is momentum, $c$ is light speed. I can't understand the red line. I feel the author think $pc$ is the kinetic energy, since the total energy should be rest energy adding kinetic energy.

But if it is kinetic energy, it should be $\frac{1}{2}mv^2$, which is not equal to $pc$, evenly $v\approx c$. So, what the $pc$ is ?

enter image description here

enter image description here

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ This is just an approximation . $$\sqrt {x+y} - \sqrt x$$ becomes smaller and smaller as $x$ becomes larger and larger compared to $y$. Thus practically $$\sqrt {x+y} ≈ \sqrt x$$ . In those cases also $p^2c^2>>m^2c^4$ so the approximation is quite acurate . Thus $W≈\sqrt {p^2c^2}=pc$ $\endgroup$
    – Users
    Commented May 23 at 7:43
  • $\begingroup$ @Users I know it is approximation. But why $pc$ is energy? The kinetic energy is $\frac{1}{2}mv^2$ which is not like $pc$. $\endgroup$
    – Enhao Lan
    Commented May 23 at 7:50
  • 2
    $\begingroup$ $\frac{1}{2}mv^2$ in not the kinetic energy in special relativity . $\endgroup$
    – Users
    Commented May 23 at 8:04
  • $\begingroup$ You may find my answer helpful: physics.stackexchange.com/a/595175/123208 especially the first part. $\endgroup$
    – PM 2Ring
    Commented May 23 at 9:08

2 Answers 2

Reset to default
2
$\begingroup$

$pc$ is the total energy of a massless relativistic particle, like the photon. Electrons do have mass, so what's the catch?

Well, if the momentum of the electron is very big, then we can neglect the rest energy, so that $E=\sqrt{(pc)^2+(mc^2)^2}\approx pc$. A massive particle for which this approximation is valid is called an ultrarelativistic particle.

Finally, with respect to your confusion with kinetic energy, in SR, $KE = \sqrt{(pc)^2+(mc^2)^2} - mc^2$, which in the non-relativistic limit ($v/c \ll 1$) is the classical $m v^2/2$ (Taylor expand, while remembering that $p = \gamma mv$).

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Thanks, could you detail talk about "the non-relativistic limit is the classical $p^2/2m$" ? It seemly be not obvious. $\endgroup$
    – Enhao Lan
    Commented May 23 at 8:16
  • $\begingroup$ Yeah, you have to do a taylor expansion considering $v/c \ll 1$, and remembering that in SR $p = \gamma mv$. I added it to the text now. $\endgroup$
    – Gabriel Ybarra Marcaida
    Commented May 23 at 8:17
  • $\begingroup$ Thanks. I forgot all about it. In fact, there is the process of calculability in 16-4 and 16-5. $\endgroup$
    – Enhao Lan
    Commented May 23 at 8:22
2
$\begingroup$

$$W=\sqrt{p^2c^2+m^2c^4}$$ approaches $W=pc$ for $p\gg mc$ and $W=mc^2+{p^2\over 2m} = mc^2+{1\over 2}mv^2$ for $p\ll mc$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.