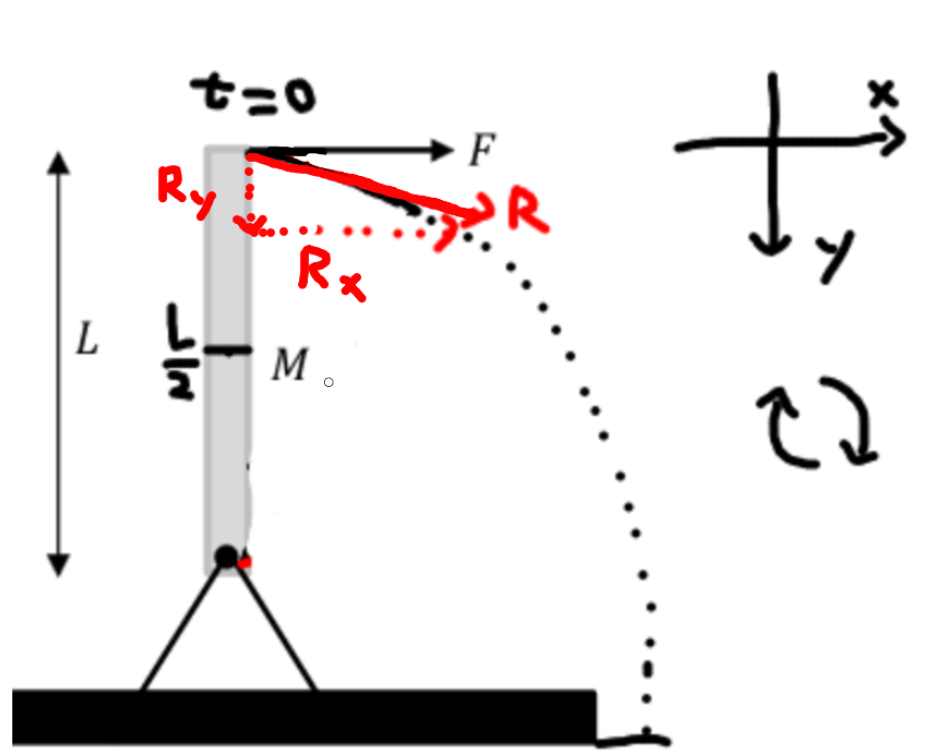
You need to consider $R_x$, that the reaction force along the x-axis, is applied on the pivot.
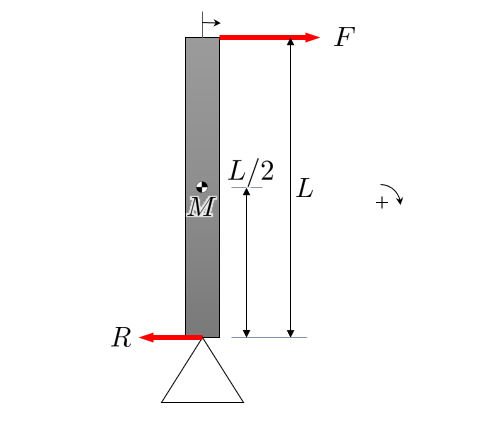
and then look at the equations of motion along the x axis and the rotational sense
$$\begin{aligned}
F - R_x & = M \ddot{x} \\
\tfrac{L}{2} F + \tfrac{L}{2} R_x & = I \ddot{\phi}
\end{aligned}$$
where $I = \tfrac{1}{12} M L^2$ is the mass moment of inertia of the rod about the center of mass.
In addition, consider the kinematics where $\ddot{x} = \tfrac{L}{2} \ddot{\phi}$ when starting for a resting position.
The equations above are solved for the two unknowns, $\alpha$ the rotational acceleration, and $R$ the requested reaction force.
$$ \begin{aligned}
\ddot{\phi} & = \frac{2 F \tfrac{L}{2}}{I +M \left( \tfrac{L}{2} \right)^2} \\
R_x & = \left( \frac{I - M \left( \tfrac{L}{2} \right)^2}{I+M \left( \tfrac{L}{2} \right)^2} \right) F
\end{aligned}$$
Using the MMOI for a rod the above is
$$ \begin{aligned}
\ddot{\phi} & = \frac{3 F} { M L} \\
R_x & = -\tfrac{1}{2} F \\
\end{aligned}$$
The direction of $R_x$ is not in the way I drew in the diagram thus.
In total, the force on the pivot is the vector sum of the side reaction and the weight
$$ R = \sqrt{ \left( M g \right)^2 + \left( \mbox{-}\tfrac{1}{2} F \right)^2 } $$
If you investigate the value and direction of $R_x$ in relation to where the force $F$ is applied, then you will discover where the axis of percussion is.
Call $b$ the distance from the center of mass where the force $F$ is applied and solve as above to get
$$ \begin{aligned}
R_x & = \left( \frac{\tfrac{L}{2}-3b}{2L} \right) F \\
\ddot{\phi} & = \frac{3 F \left(b+\tfrac{L}{2}\right) }{4 M \left( \tfrac{L}{2} \right)}
\end{aligned}$$
This means that the reaction force has the following cases
$$ \begin{cases}
R_x < 0 & b > \tfrac{1}{6} L \\
R_x = 0 & b = \tfrac{1}{6} L \\
R_x > 0 & b < \tfrac{1}{6} L \\
\end{cases} $$
The middle case where the force $F$ is applied at $1/6$ the length of the rod from the center of mass is the axis of percussion where the rotation is induced with no reaction force.