In dispersive readout of a qubit coupled to a resonator, how is the measured phase shift used to determine the resonant frequency of the resonator?

Question

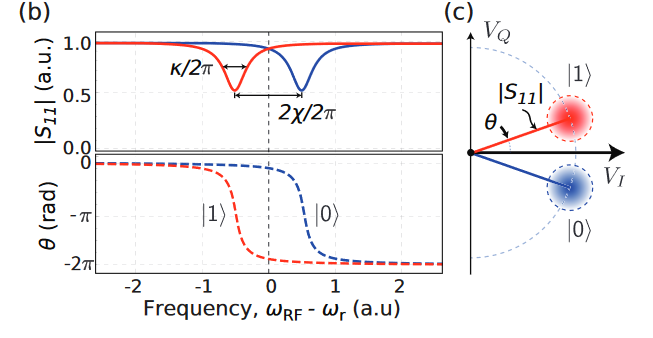
In the dispersive readout of a qubit coupled to a resonator, a microwave pulse is applied to the resonator and the phase shift of the reflected pulse is used to determine the resonant frequency of the resonator, which depends on the state of the qubit. I understand how the state of the qubit affects the resonant frequency of the resonator, but I do not understand how to relate the measured phase shift to this shifted resonant frequency.

Specifically, I ask if there is an equation that relates the phase shift of a reflected microwave pulse to the resonant frequency of the resonator. I imagine the answer has to do with how transmission line resonators work, but I am not too familiar with these. Is there a way to relate the answer to the classic RLC circuit equations?

Answer 1

In dispersive readout, there are two ways to readout the state of your qubit: amplitude and phase readout, see Chapter V in this great guide on superconducting qubits.

In the first case, the probe frequency of your microwave pulse is at one of the pulled frequencies $w_r' \pm \chi$, so depending on the magnitude of your reflected signal you know what state your qubit is in.

In phase readout, you probe at the resonator frequency $w_r'$ (dashed line), so the reflected magnitude is the same for $|0>$ and $|1>$. Therefore, all the information about the state of the qubit is encoded in the phase $\theta$. The phase shift of your readout signal therefore is simply $\pm \arctan{(2\chi / \kappa)}$.

You are not measuring the shifted resonance frequency, you just measure what phase shift is imposed on your signal. If you're interested what the shifted frequencies of your resonator look like depending in which state you're in, you can prepare in the desired state and then do a frequency sweep of $w_r'$. This way you can find the resonances corresponding to your qubit being in $|0>$ or $|1>$.

Note: $w_r'$ is not your actual bare resonator frequency but what you measure in the dispersive regime, it depends on the bare $w_r$ by \begin{equation} w_r' = \omega_r - \frac{g^2}{\Delta - E_C/\hbar} \end{equation}