3
$\begingroup$

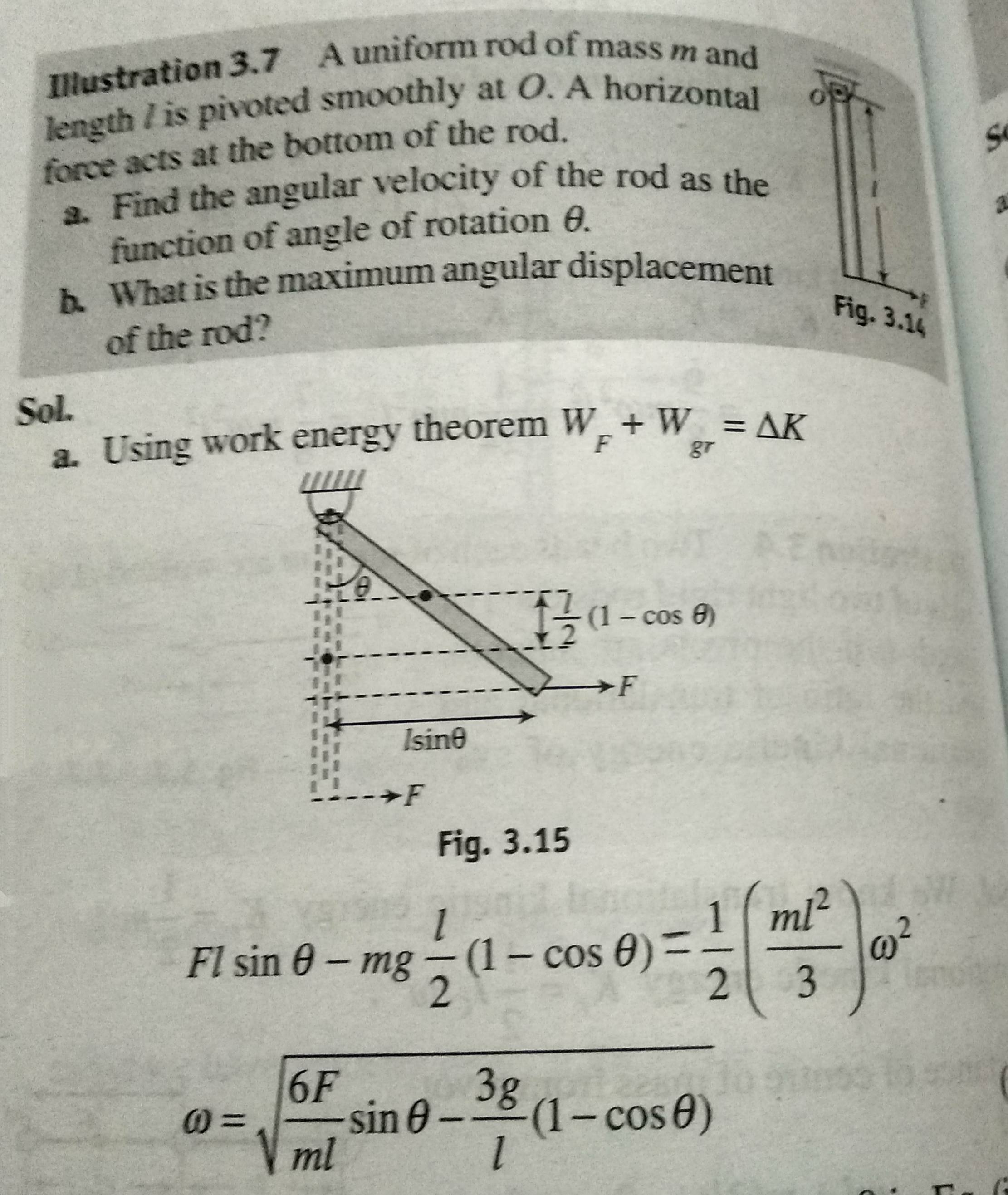
enter image description here

This is a question on work-energy theorem in rotational motion with its answer for the first part of the question. Here, I have a doubt about why is the book taking the translational work done by the gravity and not the rotational work done by the gravity? I can not understand this question, please help

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ The book takes into account just the forces that do work and enter into the energy balance. $\endgroup$
    – Jon
    Commented Mar 28 at 13:17
  • 1
    $\begingroup$ So does the gravity not to any rotational work? I find its value -mgLsin(x)/2 $\endgroup$
    – yyzr
    Commented Mar 28 at 13:23
  • $\begingroup$ The only component doing work by gravity is the one the book accounts for. $\endgroup$
    – Jon
    Commented Mar 28 at 13:42
  • $\begingroup$ Does the gravity not impart a torque on the system? I find the torque -mgLcos(x)/2, hence upon integrating I find its rotational work. Why is it wrong to do so? $\endgroup$
    – yyzr
    Commented Mar 28 at 14:00
  • $\begingroup$ @yyzr It is not wrong, it is just a different route to the same answer. The torque exerted by the rod's weight at an angle $\phi$ to the vertical is $-\frac {mgl} 2 \sin \phi$ and if you integrate this from $\phi=0$ to $\phi = \theta$ you get $\frac {mgl} 2 (\cos \theta - 1)$, so the work done against gravity comes to the same value either way. $\endgroup$
    – gandalf61
    Commented Mar 28 at 16:55

1 Answer 1

Reset to default
6
$\begingroup$

There is no such thing as "rotational work" or "translational work". Work is work and energy is energy. In fact, we can go further - "work" and "energy" are just two names for the same thing.

The horizontal force $F$ moves through a horizontal distance $l \sin \theta$ so the work done by the force $F$ is $Fl \sin \theta$. This is not "rotational work" or "translational work" - it is just work.

The centre of mass of the rod is raised by a distance $\frac l 2 (1-\sin \theta)$ so the work done against gravity is $ \frac {mgl} 2 (1-\sin \theta)$. Once again, this is not "rotational work" or "translational work" - it is just work. In fact, you could put this term on the other side of the equation and call it "potential energy gained by the rod" instead of "work done against gravity" - it comes out to the same thing.

The kinetic energy gained by the rod is $\frac {ml^2 \omega^2} 6$. You can think of this as being $\frac 1 2 I_1 \omega^2$ where $I_1$ is the rod's moment of inertia about the stationary pivot. Or you can think of it as being $\frac 1 2 mv^2 + \frac 1 2 I_2 \omega^2$ where $v$ is the velocity of the rod's centre of mass and $I_2$ is the moment of inertia about its centre of mass. You get the same answer either way, because energy is energy.

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Thank you, but I have one doubt. We can also calculate the work by integrating torque with dx, where x is the angular displacement what is that and when to use it? $\endgroup$
    – yyzr
    Commented Mar 28 at 16:26
  • $\begingroup$ Technically you are just finding the work done by the force in that case too, it is just that in those cases the force is always perpendicular to the object (it would trace out an arc). In the above question the force is always acting in the horizontal direction. When the force is always perpendicular the work would be $\int F\left(rd\theta \right)\:$. When you take $F$ and $r$ outside it seems to be as if some new formula for work done by torque. Over here I have taken $\theta $ as the angular displacement. $\endgroup$
    – Anonymousstriker38596
    Commented Mar 28 at 16:34
  • $\begingroup$ @Anonymousstriker38596 when I use this formula for force "F" I get the same answer but I get different answer if I use it for gravity. Why so? Please help $\endgroup$
    – yyzr
    Commented Mar 28 at 16:38
  • $\begingroup$ In this question you cannot use the formula I have mentioned in my previous comment for this to hold true you need the force to always act perpendicularly to the object which is not true here. Think about what $rd\theta \:$ represents, it refers to the arc length subtended angle $d\theta \:$. Here for gravitational work you could either go by change in potential energy or use $mg.x$ where $x$ is the displacement, both will give you the same result. Refer to the answer by gandalf61. There is no such thing as rotational or translation work by gravity. $\endgroup$
    – Anonymousstriker38596
    Commented Mar 28 at 16:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.