Refraction of light through a slab of variable refractive index

Question

Here I am posting a question of Jee Adv. 2023 based on refraction.

A monochromatic light wave is incident normally on a glass slab of thickness 𝑑, as shown in the figure. The refractive index of the slab increases linearly from 𝑛1 to 𝑛2 over the height ℎ. Which of the following statement(s) is(are) true about the light wave emerging out of the slab?

(A) It will deflect up by an angle tan^-1[(n₂²-n₁²)d/2h]

(B) It will deflect up by an angle tan^-1[(n₂-n₁)d/h]

(C) It will not deflect.

(D) The deflection angle depends only on (𝑛₂ − 𝑛₁ ) and not on the individual values of 𝑛₁ and 𝑛₂.

As per the official answer key correct answer is 2 and 4, but I am unable to understand that why light will bend if angle of incidence is zero then as per snell's law angle of refraction must be zero?

Answer 1

You can easily argue that if you do a variable transformation from a linearly varying refractive index with a fixed distance, to a fixed refractive index with a variable distance it is easier to see that you are essentially looking at a prism.

To validate the argument, consider that for the ray picture you can state that the time it takes for a ray to travel through the slab will be:

$t_{1,2} = dn_{1,2}/c$

and if we look at a prism, the equation will then be:

$t'_{1,2} = d'_{1,2}n'/c$

Since the refractive index changes linearly, you can state that the time it takes from the top of the slab and the top of the prism is the same, and the same relation applies to the bottom of the slab.

$t_{1,2} \stackrel{\text{!}}{=} t'_{1,2} $

From here we need to derive the angle of refraction in this transformed system then you can easily find it through the tangent of the angle:

$\tan \theta = opp / adj = \Delta n / h = \Delta d / h$

Since we know the refractive index contrast, we can easily get to answer B), and again, it is proven through the variable transformation that the deflection angle does not depend on individual values, rather the difference of the refractive indices.

There are more contrived ways to look into it in the wave image but I think this is sufficiently sound of an answer given the provided information.

Answer 2

The wavefront will emerge from the slab tilted at an angle. The question asks about deflection of the wave, and the wavefront is the locus of all points in phase. In the emergent wave, clearly the ray leaving from the top will be out of phase with the ray leaving from the bottom - note that the optical path length travelled near the top of the slab (AE) would be more than that of a ray near the bottom (BC), if we just consider how much they travelled within the slab.

By finding the extra path travelled (CD) outside the slab (to make up for the lost phase), the question can be solved.

Note that due to the variable refractive index (vertically), the ray will be deflected. However, we can assume an infinitesimally thin horizontal strip (and here, ray optics breaks down) of the slab to have uniform refractive index. The wave nature of light can be exploited here, by considering point sources at A and B according to Huygens' principle, and oscillations of vectors in this strip. By analysing the optical path travelled by the wave in that thin horizontal strip of medium (along AE and BC) and further ahead, the situation becomes clearer. Ultimately, the change in phase is what decides that the ray has deflected, when we look at it in air.

Notes:

1. As a previous user explained, the behaviour is like that of a prism.
2. The extra path is denoted by CD in the diagram.
3. Focus on treating light as a wave rather than a ray here.

I would love to hear from more experienced users about polarization in this case.

Answer 3

When using Snell's Law it's easy to forget that the index of refraction is determined by the speed of light through that material. As indicated in the image, the normal wavefront will move faster at the bottom of the slab than the top and this is why the wavefront changes angle. The speeds at the top and bottom of slab are:

$$v_2 = \frac{c}{n_2}$$ and $$ v_1 = \frac{c}{n_1}$$

Let x be the extra path traveled then the angle of refraction is:

$$\tan{\theta} = \frac x h$$

Now let $$ d = v_2t$$ and $$d = v_1t_1$$

These are the respective times and velocities to cover the distance d in the slab

Now $v_1$ passes through the slab first and then travels the extra distance x. Assuming that the medium outside the slab has n = 1 then: $$ x = ct_2$$

In order for the entire wavefront to pass through then $ t= t_1+t_2$ and $$x = c(t-t_1)$$

Substituting for $t$ and $t_1$ gives:

$$ x = c(\frac d v_2 - \frac d v_1)$$

$$ x = c(\frac{d n_2} c - \frac{d n_1} c)$$

Finally, $$ x = d(n_2 -n_1)$$ and $$\tan{\theta} = \frac{(n_2-n_1)d} h$$

Answer 4

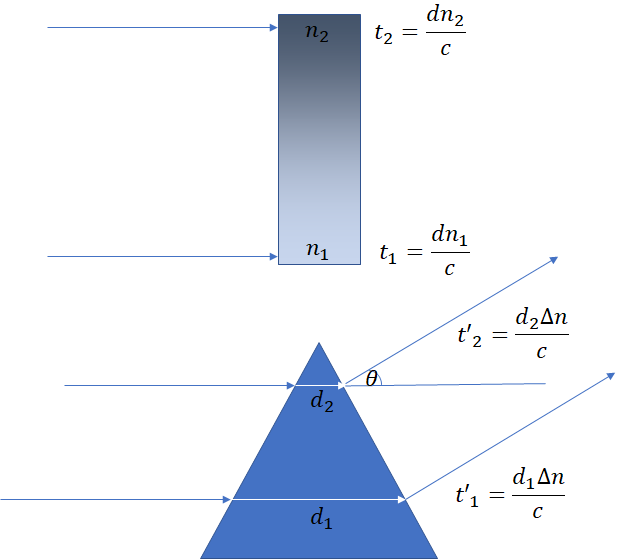
«as per snell's law angle of refraction must be zero»

Snell's law assumes a homogeneous medium

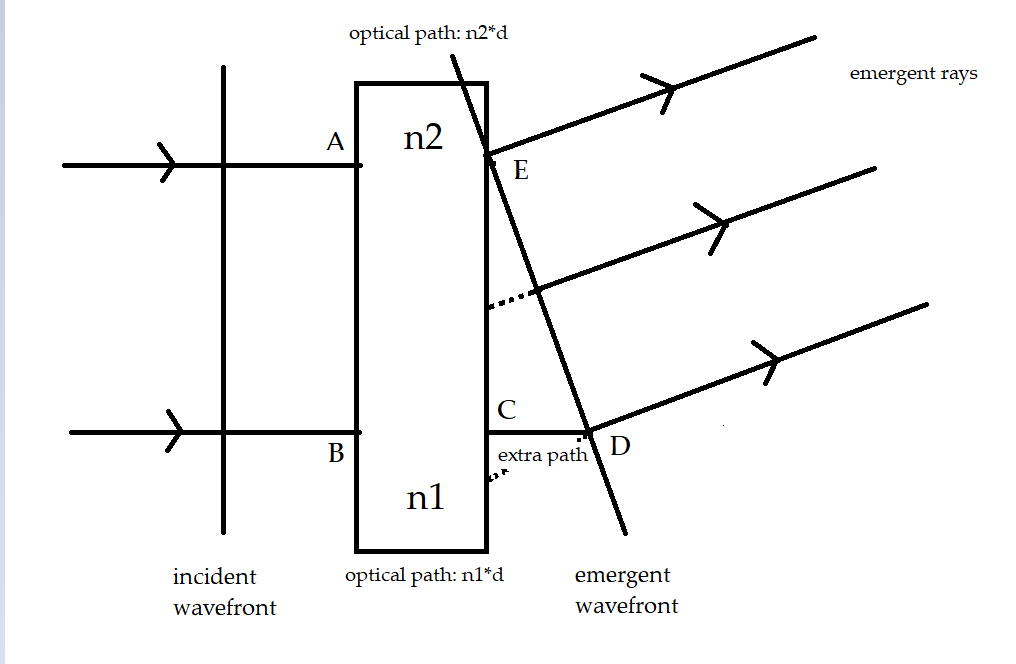
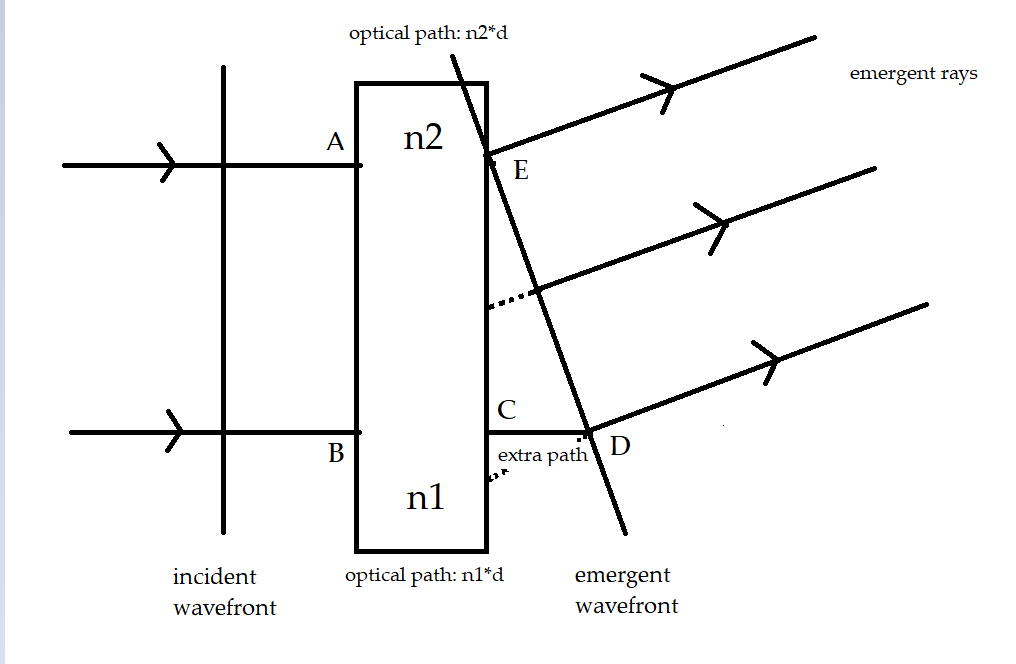
Snell's law assumes a homogeneous medium (in which the refractive index is the same everywhere). Because of this homogeneity, all points of the medium on the surface become identical point sources of spherical wave fronts (Huygens' principle). In the figure, the flat front on the left reaches points $O_1$ and $O_2$ on the surface of the plate.

All point sources on the surface of a homogeneous medium have the same speed of light propagation (this is the speed of light $C$ in vacuum divided by the refractive index $n$ of the medium). Therefore, a flat front parallel to a flat homogeneous surface remains the same after passing through it (there is no refraction). If the medium is inhomogeneous (in the figure, the refractive index at the top is large $n_2$, and at the bottom is small $n_1$), spherical fronts from point sources will run into the medium at different speeds. Therefore, the plane touching them (a flat wave front) will no longer be parallel to the surface. It will bend towards it in those areas where the speed of light is less (the refractive index is greater), i.e. at the top of the picture.

Over some time $T$, the spherical front from the upper point $O_2$ will pass with a small speed $C_2$ (due to the large refractive index $n_2$) a small distance $O_2B_2= TC_2= TC/n_2$, and from the lower point $O_1$ - a large distance $O_1B_1= TC_1= TC/n_1$. The plane tangent to these spherical fronts (in the figure this is straight line $A_1A_2$, tangent to the circles with centers $O_1$ and $O_2$), forms an angle with the surface $O_1O_2$ approximately equal to angle $α$ in the right triangle $B_1B_2D_1$. This approximation is more accurate the smaller $α$, i.e. the smaller the difference between $n_2$ and $n_1$. In this approximation, we replace the tangent section $A_1A_2$ with the secant section $B_1B_2$ and obtain from the triangle $B_1B_2D_1$

$tg(α)= (D_1B_1/O_1O_2)= (|O_1B_1-O_2B_2|/h)= (TC/n_1-TC/n_2)/h= TC(1/n_1-1/n_2)/h$.

This plane gradually moves inside the plate and rotates relative to its surface.

Due to the linearity of the refractive index gradient, the radii of all spherical fronts with sources between points $O_1$ and $O_2$ will vary linearly on this segment. Therefore, the plane $A_1A_2$ will touch all these spherical fronts and thereby turn out to be the desired plane wave front inside the medium. If the gradient of the refractive index between points $O_1$ and $O_2$ is not linear, but convex, then the wave front will be distorted accordingly (it will be similar to focusing light in lenses).

But let's return to the case of a linear gradient. The approximate flat front $B_1B_2$ moves inside the plate and simultaneously rotates relative to its surface until its slowest upper point $B_2$ at speed $C_2$ passes the entire thickness $d$ of the plate to point $R_2$ in time $T_2= d/C_2= dn_2/C$. A spherical front will begin to emit from this point on the surface at this moment. But by this moment, the fastest bottom point of the front $B_1$ will not only have time to reach point $P_1$ on the surface, but also emit a spherical front, which will have time to reach some point $R_1$. The lower point $B_1$ will pass the entire thickness of the plate $d$ to point $P_1$ on the surface in time $T_1= d/C_1= dn_1/C$. At this moment, a spherical front will appear from point $P_1$, which in the remaining time $T_2-T_1$ will cover the distance

$P_1R_1= (T_2-T_1)C= (dn_2/C-dn_1/C)C= d(n_2-n_1)$.

As a result, a flat front will emerge from the surface, approximately coinciding with $R_1R_2$ (similar to what was for $A_1A_2$). From the right triangle $R_2R_1P_1$ we obtain

$tg(φ)= P_1R_1/h= d(n_2-n_1)/h$

Q.E.D.

Answer 5

This simply can by answered by remembering a very useful concept. Remember the case in YDSE (Young's Double...) where we put 2 different slabs on the slits so that they are fully cover. So. $\Delta x= h\sin\theta$ where $\Delta x$ would be $t_1(n_1-1)-t_2(n_2-1)$

in the above case $t_1=t_2$ (thickness is same) So, simply $t(n_1-n_2)/h = \sin\theta$