0
$\begingroup$

Can anyone guide me how can I find $C_P$ and $C_V$ ? There are two equations in Heat and Thermodynamics: An Intermediate Textbook Textbook by Mark Zemansky and Richard Dittman, which I think can help but I'm not sure if I can use them to find $C_P$ and $C_V$ of a Van der Waals gas. First equation:

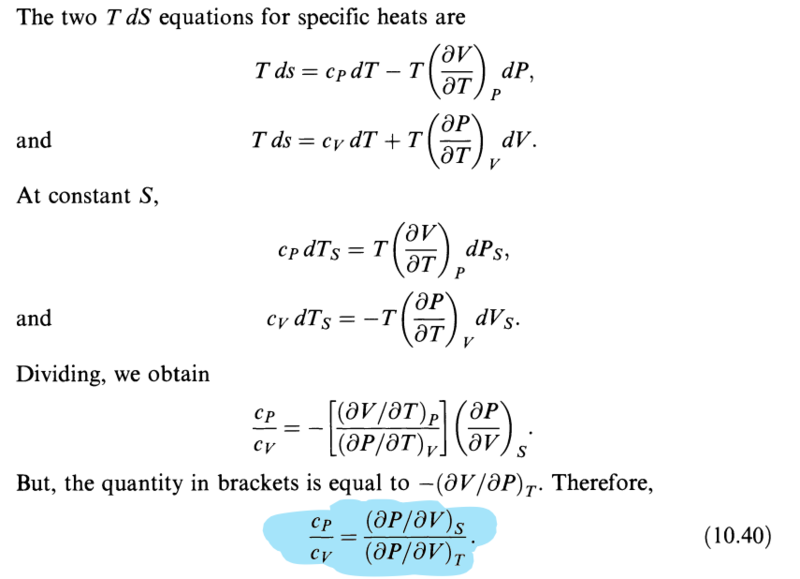
enter image description here

Second equation: enter image description here

Is there any book or source which discuss this thing???

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Please consider writing the equations and keeping just the important part. Images are large and can break easily. $\endgroup$
    – Mauricio
    Commented Jan 31 at 17:18
  • $\begingroup$ @Mauricio Thanks. I've thought if I only write 2 final equations then no one will understand them. $\endgroup$
    – seymatinsar
    Commented Jan 31 at 17:23
  • $\begingroup$ The first equation is called Mayer's relation and the second is the heat capacity ratio. Both are well known you can just cite Wikipedia. $\endgroup$
    – Mauricio
    Commented Jan 31 at 19:32
  • $\begingroup$ Ok, I didn't know that. Thanks for your guidance ... The book is: Heat and Thermodynamics: An Intermediate Textbook Textbook by Mark Zemansky and Richard Dittman $\endgroup$
    – seymatinsar
    Commented Jan 31 at 19:49

1 Answer 1

Reset to default
3
$\begingroup$

The two equations you cite: $$ C_P-C_V=-T\left( \frac{\partial V}{\partial T} \right)^2_P \left( \frac{\partial P}{\partial V} \right)_T $$ and $$ \frac{C_P}{C_V}= \frac{\left( \frac{\partial P}{\partial V} \right)_S}{\left( \frac{\partial P}{\partial V} \right)_T} $$ can only provide ine $C_P$ and $C_V$ in terms of the thermal expansion coefficient $$ \alpha = \frac{1}{V}\left( \frac{\partial V}{\partial T} \right)_P $$ and the adiabatic and isothermal compressibilities $\chi_S$ and $\chi_T$ defined as $$ \chi_S = -\frac{1}{V}\left( \frac{\partial V}{\partial P} \right)_S; ~~~~~~~~~~~~~~ \chi_T = -\frac{1}{V}\left( \frac{\partial V}{\partial P} \right)_T. $$

The problem is how we can get the adiabatic compressibility if by van der Waals gas we intend a system of atoms such that the equation of state $p=p(N,T,V)$ coincides with van der Waals' equation of state. It should be clear that the equation of state is not enough to reconstruct any thermodynamic potential. Therefore, some information is missing and should be added to the equation of state.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I think $\alpha$ and $\chi_T$ can be calculated from the equation of state see theory.physics.manchester.ac.uk/~judith/stat_therm/node50.html the problem seems to be $\chi_S$ $\endgroup$
    – Mauricio
    Commented Jan 31 at 19:31
  • 1
    $\begingroup$ @Mauricio, that's precisely my point. From $p=p(N,V,T)$ alone, there is no way to recover the entropy or the heat capacity. A simple proof is that, in the case of the ideal gas, the same equation of state is valid for monoatomic and diatomic gases that have different specific heats. $\endgroup$
    – GiorgioP-DoomsdayClockIsAt-90
    Commented Jan 31 at 19:40
  • $\begingroup$ Thanks for your reply. So the way I've tried does not work. Do you know a better way? Or Can you suggust a source about this topic ... $\endgroup$
    – seymatinsar
    Commented Jan 31 at 19:52
  • $\begingroup$ @seymatinsar The problem is due to some information missing in the equation of state. This implies that one has to add such information in order to evaluate specific heats and other thermal quantities. Une possibility is shown in Callen's book, where the van der Waals equation of state is complemented with a second relation $U=cNkT$ ($c$ being a constant). However, such a choice is not unique and not well justified. $\endgroup$
    – GiorgioP-DoomsdayClockIsAt-90
    Commented Feb 1 at 7:01
  • $\begingroup$ Thanks for your help and valuable time @GiorgioP-DoomsdayClockIsAt-90 $\endgroup$
    – seymatinsar
    Commented Feb 1 at 12:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.