How is a resonant bandpass filter similar/different from a damped mass-spring oscillator? They seem to behave both similar and different in testing

Question

Background

I am using resonant bandpass filters as musical oscillators. One can excite an array of them at harmonic frequencies and given Q values for a note by, for example, running a burst of noise through them.

I thought intuitively that an array of damped mass-spring oscillators tuned to the same Q and frequencies should perform the same as the resonant bandpass array.

The result is they behave similarly but also differently in some ways.

Damped Mass-Spring Oscillator

I set up some with the following code, where instead of running the audio input through the bandpasses directly as input samples, I converted the input exciter audio into force and then used that to drive the mass-spring oscillators.

I thought this would be the Newtonian way to handle this in theory. (Correct?)

double processNextSample(double sampleInput) {

    //SOLVE DRIVING FORCE BEING PUT INTO IT FROM EXCITER SIGNAL
    in_1 = in_0;
    in_0 = sampleInput;

    inVel_1 = inVel_0;
    inVel_0 = (in_0 - in_1) * sampleRate;

    inAcc_0 = (inVel_0 - inVel_1) * sampleRate;
    F_input = inAcc_0 * oscMass; //use imaginary mass as 1 kg to keep amplitude the same

    //PROTECT AGAINST HIGH FREQUENCIES DUE TO INSTABILITY
    if (springFreq > 21000 || springFreq > 0.08 * sampleRate) {
        return 0;
    }

    //SOLVE MOTION OF DAMPED MASS-SPRING OSCILLATOR
    double F_dampedSpring = (springK * currentPos) + (dampCoeff * currentVel);
    currentForce = F_input - F_dampedSpring;
    currentVel += currentForce * deltaTime;
    currentPos += currentVel * deltaTime;
        
    return currentPos;

Similarities/Differences

This creates a similar effect in that I can get the expected resonances of frequencies and the musical note comes through the same at the same amplitude. There are two main differences:

1) Stability

It is far less stable. I have to limit the frequencies relative to the sample rate as at higher frequencies it is failing. I believe it is going into NaN and inf territory easily. I am not sure why.

Perhaps the input force or stepwise position/velocity solution is too crude and discontinuities are resulting in massive forces randomly? Whereas the filter (using this one) handles this with better math somehow?

Or perhaps it is because as in point (2) below, it is letting high freqs through, and being forced into very rapid motion obviously then, the damping term is getting too big and becoming problematic at the sample rate with these high freqs as it is not parametized for this purpose, and pushing it into error.

2) Frequency Response

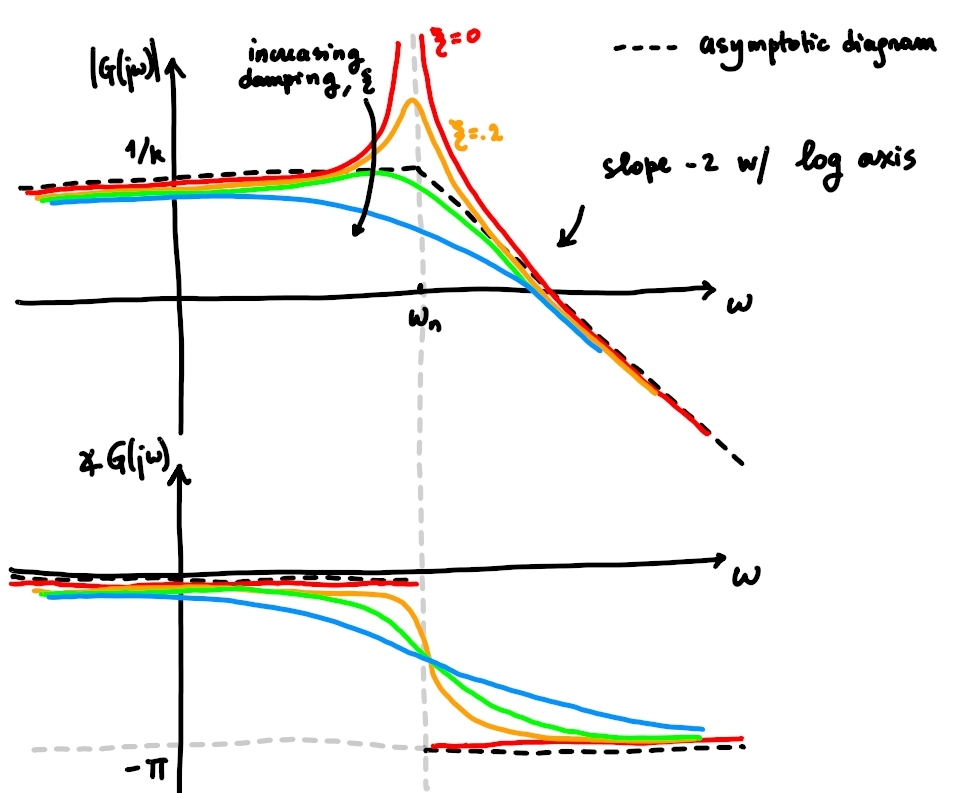
It sounds like it lets all the high frequencies from my exciter noise bursts through it completely, whereas the resonant bandpass filters these out. ie. If I have a single mode (bandpass or oscillator) at 80 hz, with the bandpass, I only hear ever sound around 80 hz (it filters above and below). With the oscillator, I hear the full high frequency spectrum of the burst of sound as it goes through. Not sure about the lows, but the highs are obviously passing through.

Questions

Based on this experiment, it seems the damped harmonic oscillator is not equivalent to the resonant bandpass.

What is the harmonic oscillator equivalent to then? Is it a resonant high pass filter?

What would be the mechanical/Newtonian equivalent to the resonant bandpass if one exists?

Why also (in layman's terms) is the harmonic oscillator so unstable compared to the filter?

Thanks for any thoughts or ideas.

EDIT

Based on replies and comments so far that the harmonic oscillator should certainly be identical to the bandpass, then I must presume the burst of noise I am getting out of the harmonic oscillator on excitation is not the exciter noise passing through (not a high pass filter), but rather some sample rate related quantization error in my force conversion code which is creating a new noise burst.

I didn't think of that possibility. Thanks for the feedback.

Answer 1

Fast look into your code. It looks like you're using a time-explicit numerical method (Explicit Euler, $1^{st}$ order explicit method), that is very likely to make your simulation diverge. My suggestion: use some routine from some numerical library to perform the numerical integration with the numerical scheme you need/like the most: maybe some Runge-Kutta method is ok for you (high order could help in avoiding extra numerical damping, common of low order schemes), I don't know if you need some stiff integrator; but if you use some numerical library you can change the numerical method by changing only one or few lines of the code.

Damped spring-mass system. I won't go into lines of code here, but I'll answer that a damped spring-mass system \begin{equation} m \ddot{x}(t) + c \dot{x}(t) + k x(t) = f(t) \ , \end{equation} or in its "non-dimensional" form (with $\frac{k}{m} = \omega_n^2$, $\frac{c}{m} = 2 \xi \omega_n$), \begin{equation} \ddot{x}(t) + 2 \xi \omega_n \dot{x}(t) + \omega_n^2 x(t) = \dfrac{1}{m} f(t) \ , \end{equation} is a low pass filter, with a range of frequency of amplification approximately around the natural frequency $\omega_n$, if not damped enough.

You can easily realize that, transforming in Laplace or Fourier domain, \begin{equation} \begin{aligned} x(s) & = \dfrac{1}{m} \dfrac{1}{s^2 + s \, 2\xi\omega_n + \omega_n^2 } f(s) = G(s) f(s) \qquad \qquad \text{(Laplace)} \\ x(j\omega) & = \dfrac{1}{m} \dfrac{1}{\omega_n^2 - \omega^2 + j 2\xi\omega_n \omega} f(j\omega) = G(j\omega) f(j\omega) \qquad \text{(Fourier)} \ , \end{aligned} \end{equation} and plotting Bode diagram for a graphical description of the harmonic response of the system.