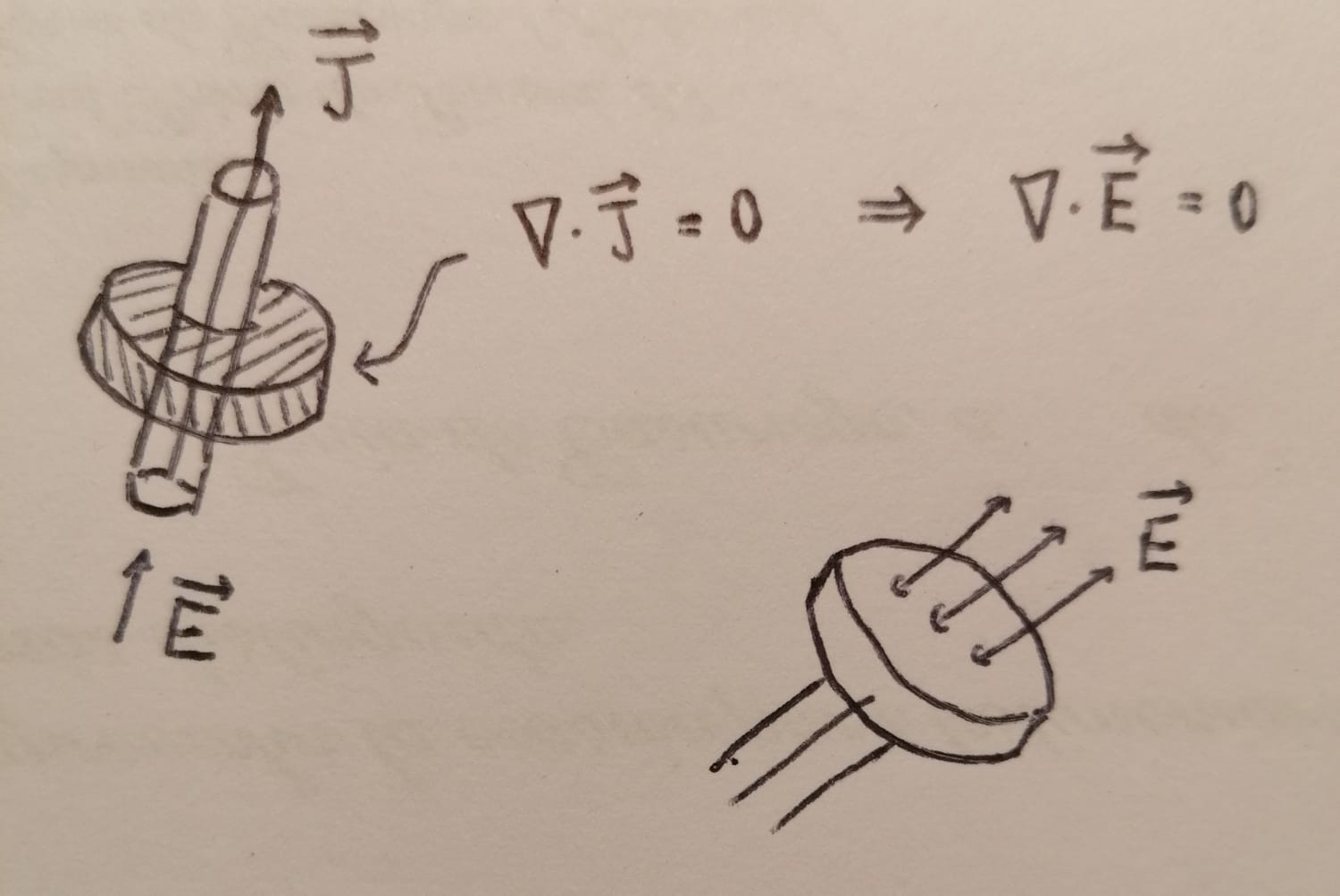For steady current, why volume charge density is zero?

Question

The divergenc of steady current density is zero

$\nabla \bullet \vec{J}=0 $

And, by microscopic Ohm's law $ \vec{J}=\sigma \vec{E} $

If the conductivity is uniform, we can get $\nabla \bullet \vec{J}=\sigma \nabla \bullet \vec{E}=0$.

That is, $\nabla \bullet \vec{E}$

And, by gauss's law ( $\nabla \bullet \vec{E}=\frac{\rho_v}{\epsilon_0}$), $\nabla \bullet \vec{E}$ implies that the volume charge density $\rho_v$ is zero.

But, i can't understand why the charge density is 0. Of course, when current flows in a wire, the positive and negative charges are balanced, so the charge density is 0.

However, if the free charges in the vacuum constitute a steady current (a), then it makes no sense for the charge density to be 0.

What am I misunderstanding? Or does a situation like (a) make no sense?

Answer 1

In a metal your argument leading to $\vec \nabla.\vec E=0$ is valid. So, according to Gauss's law, the NET charge per unit volume is zero. In a metal, that's the algebraic sum of the charge density of free electrons (negative) and of ion cores (positive). The charge density, $\nu_e$, of free electrons is not zero. Indeed we use it in the equation $I=\nu_e Ave$.

"However, if the free charges in the vacuum constitute a steady current (a), then it makes no sense for the charge density to be 0." In a vacuum, $\vec J = \sigma \vec E$ doesn't apply; it requires resistive forces to act on the charge carriers, for example from their collisions with vibrating ions. Therefore the argument leading to $\vec \nabla .\vec E = 0$ fails and the charge density doesn't have to be zero – which it clearly isn't in a beam of charged particles passing through a vacuum!

Answer 2

Keep in mind that $\rho$ is the net charge density. A neutral uniform conductor has equal positive charge in the nuclei and negative charge in the electrons. If the electrons are moving in one direction with a certain average velocity, the conductor is still neutral, but now has current through it. There is no contradiction here.

If we had a positively charged region on the interior of a uniform conductor, this would put an outward force on nearby positive charges, causing a transient flow of charges that quickly neutralizes the region. This is what your equations are saying: since we are not allowing transient currents, the conductor must be neutral on the interior. As Sagar K. Biswal has mentioned in a comment, these equations do not preclude the existence of charges on the surfaces of conductors, just like in electrostatics. Surface charges do exist and are partially responsible for shaping the E-field such that current flows along wires in a circuit.

Finally, note that your key assumption of uniform $\sigma$ is violated in all sorts of cases and in interesting devices. This includes the ubiquitous case of charge flow across the interface of two dissimilar conductors (with different conductivities). Since $\sigma$ has a discontinuity at the interface, there is surface charge at this interface whenever there is current flow. See this question for a simple example of this.

Answer 3

Divergence measures the difference between the outflow and inflow of a vector field on the surface surrounding a volume.

If you Have a constant current, both inside a conductor or in outer space, as you mention, the divergence of the current density is zero.

Now if you suppose that this current is created by an electric field (as you assume when you use the Ohm's law) you get that the divergence of the electric field is zero. Here's the issue. That divergence corresponds to the field that's moving those charges, not to the one created by the current. That divergence ins´t telling you anything about those charges, only about the field that is moving them (more precisely, is telling you that in that specific volume you took there are no sources aka charges creating that field).

It's clear that the divergence equals zero if we consider the surface shown in the figure below, since the electric field lines enter from one tap of the cilinder and exit from the other tap, while there is a non zero charge density.

If we take de divergence of the electric field created by the current in that specific surface, it wouldn´t be zero.

Hope this answers your question !!. I think this is where that little contradiction comes from.