Consider a two-body system where $m_1$ and $m_2$ are attached to each other by a string when m1 is pulled with an additional string then it pulls m2 along with it. The acceleration is constant since the system demonstrates a single body behavior. Then if m2 is lesser than m1 it means that the force transmitted to m2 has been reduced because if it was the same then it would produce a greater acceleration and vice versa. What causes this change in force? Why isn't the original force transmitted to m2
$\begingroup$
$\endgroup$
0
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
1
Even when $m_2 > m_1$ the force of the string is less than the pull force.
The reason? Some of the pull force goes to accelerate $m_1$ and only the remaining goes through the string.
answered Oct 25, 2023 at 22:06
-
$\begingroup$ That's what my professor said when I asked him,he said it's because m1 has it's own inertia and hence m2 isn't pulled with the same force but rather decreased in magnitude. The idea is still vague and I don't know how it happens $\endgroup$ Commented Oct 26, 2023 at 4:30
$\begingroup$
$\endgroup$
6
Why isn't the original force transmitted to m2
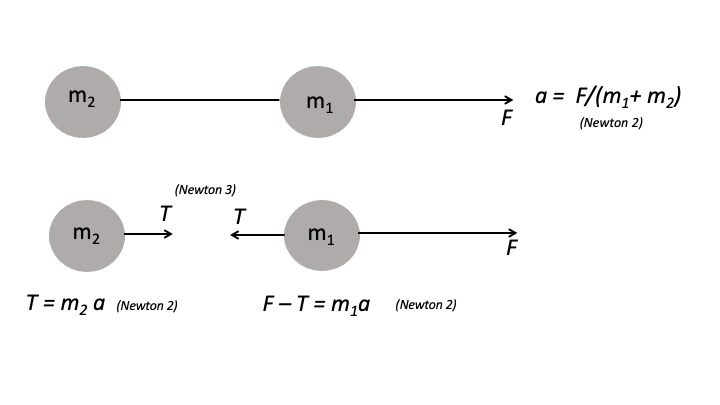
In order for the two masses to accelerate together due to the pulling force $F$ on $m_1$, the pulling force on $m_2$ must be less than $F$, regardless of the magnitudes of the two masses.
To see this, refer to the free body diagrams (FBD) below, which assumes the only net external force on the system of masses is $F$, and that the strings are massless and inextensible.
From the FBD on $m_1$, for $m_1$ to accelerate to the right the tension force $T$ to the left must be less than $F$. From the FBD on $m_2$, the only external force acting on $m_2$ is the tension force $T$ to the right. Therefore the force acting on $m_2$ must be less than $F$.
Hope this helps.
-
$\begingroup$ "Then since the force the connecting string applies to 𝑚2 has to be equal and opposite to the force the connecting string exerts on 𝑚1 per Newton's 3rd law..." Per Newton's 3rd law it has to be equal to the force m2 exerts on the string, no? $\endgroup$ Commented Oct 27, 2023 at 12:34
-
$\begingroup$ @Not_Einstein Not sure what you are trying to say. In the diagram, the force that m2 exerts on the string is F21 and the force m1 exerts on the string is F12. The two forces are equal and opposite per N3. $\endgroup$– Bob DCommented Oct 27, 2023 at 13:05
-
$\begingroup$ In your diagram let's assume F12 and F21 are positive numbers. F12 is the force the string exerts on m2, so by Newton's 3rd law -F12 (to the left) is the force m2 exerts on the string. In you diagram, F21 is the force the string exerts on m1, so by Newton's 3rd law F21 (to the right) is the force m1 exerts on the string. Adding the forces on the string, F21-F12 = ma where m is the mass of the string. Assuming a massless string, F12 = F21. It appears you skipped some of these steps by not applying Newton's 3rd law correctly. $\endgroup$ Commented Oct 27, 2023 at 20:09
-
$\begingroup$ @Not_Einstein "let's assume F12 and F21 are positive numbers." I don't understand what you mean by this statement. F12 and F21 are force vectors having the same magnitude but opposite direction. Please elaborate $\endgroup$– Bob DCommented Oct 28, 2023 at 0:08
-
$\begingroup$ Maybe I could have worded it better i.e. that F12 and F21 are the magnitudes of the forces (hence positive numbers). $\endgroup$ Commented Oct 28, 2023 at 15:04
$\begingroup$
$\endgroup$
3
The idea of force being "transmitted" has no base or usefulness. The force is a quantity associated with the interaction between two objects. It does not get transmitted to a third object. The force on the third object depends on this body's interactions with whatever it comes on contact (or with the gravitational field). It's only a coincidence that in some special setup the force for some interaction is equal with the force from another. Like when the normal force is equal to the weight for a body at rest on a horizontal plane. This does not mean that the weight is "transmitted" to the plane. If the plane is inclined, the normal force is not equal to the weight of the body.
-
$\begingroup$ So why does the force that acts on m2 is less than that on m1 when one force was exerted in the first place? $\endgroup$ Commented Oct 25, 2023 at 18:29
-
$\begingroup$ There is no reason not to be less. Your assumption that it has to be same has no basis. There is no special status of the force exerted on the first object. All forces are exerted by one object on another. $\endgroup$– nasuCommented Oct 25, 2023 at 19:00
-
$\begingroup$ And if it's incorrect to say that force is transmitted i guess you need to call Halliday Resnick and Krane because the same word is used there in the same topic $\endgroup$ Commented Oct 26, 2023 at 4:53
$\begingroup$
$\endgroup$
2
Expanding somewhat on what @nasu has said, the key point is that each force comes about because of an interaction between two objects. There is no reason to expect that one force will equal another, unless you can show via Newton's Laws that they should be equal. The two strings in your example cannot talk to each other to agree on how big the forces they exert should be, and so we have no reason to expect them to exert the same force. So, how do they "decide" on what force to exert?
I'm going to say the string being used to pull the whole thing along (the string just attached to $m_1$) is string A. The string connecting $m_1$ to $m_2$ is B. Presumably the other end of A is connected to something (a person's hand, perhaps).
Strings exert a force that is "exactly what is needed" to achieve the effect we are seeing? How do they "know" what is needed? They are secretly springs, and they deform, with a varying force until the force is "just right". So, when you hang something on a string the string will stretch, exerting bigger and bigger forces, until the thing is stationary (actually, it will oscillate, but the oscillations damp out quickly...). We don't notice this because the string is a very stiff spring, and so the amount of stretch is not easily seen by us.
So, when this setup of masses and blocks first started to be pulled by the hand (or whatever) the strings went through a brief time of slightly changing length until the system settled into a dynamic equilibrium with both strings maintaining constant lengths. String B is needing to apply just enough force on $m_2$ for $m_2$ to accelerate at the same rate as $m_1$. If it exerts too small a force then $m_2$ falls behind and string B will stretch, increasing the force it exerts. So, it settles (quickly!) into exerting a force that is just equal to $m_2 a$.
String A somehow has to "know" that it is pulling $m_1$ and $m_2$ and so it is exerting a force of $(m_1 + m_2) a$. Of course it doesn't "know" this at all! For $m_1$ to accelerate at a rate of $a$, despite the fact that string B is pulling back on it with a force of $m_2 a$, string A must exert a force of $(m_1 + m_2) a$. Again, if string A fails to do this, then string A's length will change, changing the force it is exerting, until equilibrium is achieved, which in practice would occur on timescales of milliseconds or faster, which is why we don't notice this process.
The key point is that the strings can't talk to each other. In fact this is all governed by interatomic interactions in the strings, and each atom only "knows" about its immediate environment. So all forces have to be governed by "local" interactions. String A only interacts with the hand and with $m_1$. String B only interacts with $m_1$ and $m_2$. The forces that result are set by local conditions. No force is "transmitted". There is no meaning to saying that a force is "transmitted". Forces are local.
-
$\begingroup$ I'll put it this way: m1 is accelerated by a force m1a then it SHOULD pull along with it m2 with the exact same force shouldn't it? There's an obvious reason for it to know that it should do it and that is m2 is attached to m1 which is in contact with the original force when m1 is pulled along with m1a the m2a is attached to it and it pulls m2 with the same acceleration but not the same Force!! I asked my professor and he said it's because m1 has it's own inertia and that's why force drops $\endgroup$ Commented Oct 26, 2023 at 4:24
-
$\begingroup$ The acceleration of m1 is the result of the net force on m1. This is the resultant of the applied force and the tension in the string. None of these alone is equal to m1a by itself and is not the force accelerating m1.there is no individual force equal to m1a to be "transmitted" or shared. The m1 have different interactions than m2 so it is natural to be acted by different forces. $\endgroup$– nasuCommented Oct 26, 2023 at 11:27
$\begingroup$
$\endgroup$
The situation is more clear if we use the equivalence principle, and imagine $m_1$ and $m_2$ linked vertically by a spring. And another spring links $m_1$ to the roof. The system is at rest, and subject to the gravity acceleration.
That later spring will deflect more because supports both masses. The spring between $m_1$ and $m_2$ will deflect less because it is supporting only the mass of $m_2$.
As the force is proportional to the deflection of the springs, it follows that the force on the spring joining the system to the roof is greater than the other one.
answered Oct 25, 2023 at 21:38