Derivation of relativistic uniformly accelerated motion

Question

I'm trying to understand solution of the following problem from Landau, Lifshitz, Classical Theory Of Fields:

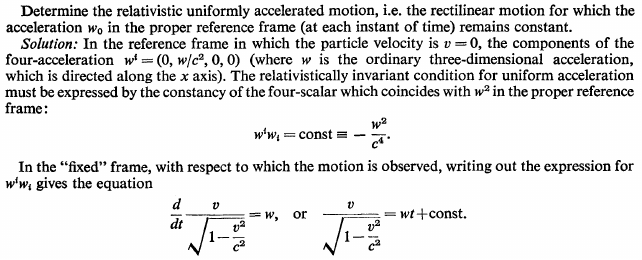
(ending skipped).

What I see when I "write out the expression for $w^iw_i$", using definition $w^i=\frac{du^i}{ds}=\frac{du^i}{cdt\sqrt{1-v^2/c^2}}$: $$\frac1{c^2-v^2}\left(\left(\frac{d}{dt}\frac1{\sqrt{1-v^2/c^2}}\right)^2-\left(\frac{d}{dt}\frac{v}{c\sqrt{1-v^2/c^2}}\right)^2\right)=-\frac{w^2}{c^4}$$

I then take the derivatives and arrive at the following result: $$-\frac{c^2\dot v^2}{(c^2-v^2)^3}=-\frac{w^2}{c^4}$$

I can get rid of the squares, use some guessing to determine correct signs, and then solve the resulting equation, getting correct results (according to the book). But the original solution in the book looks way simpler and must be better justified than mine.

So, the question: how did LL get their bottom-left equation? Did they "write out the expression for $w^iw_i$" in some other way than I did? How should I do here instead?

Answer 1

I work in units $c=1$, I use the notation $\gamma = \frac{1}{\sqrt{1-v^2}}$.

The relation between the proper time $\tau$ and the time $t$ is :

$$dt = \gamma ~ d \tau\tag{1}$$

In the particle-comoving inertial frame $F$, the 4-velocity of the particle is :

$$U^\mu = (1,0,0,0)\tag{2}$$

We define the acceleration by $A^\mu = \large \frac{dU^\mu}{d\tau}$, we note that, because $U^\mu U_\mu = 1$, then $A^\mu U_\mu = 0$, so the acceleration has only spatial component that we choose on the axis $x$ :

$$A^\mu = (0,w,0,0)\tag{3}$$

Now, in the stationnary inertial frame $F'$, the $4$ velocity is :

$$U'^\mu = \gamma (1,v,0,0)\tag{4}$$ You may check this by noticing that $U^\mu$ transforms like a vector in a Lorentz transformation, so we have simply :

$$\begin{pmatrix} U'^0\\U'^1\end{pmatrix} = \gamma \begin{pmatrix} 1&v\\v&1\end{pmatrix}\begin{pmatrix} U^0\\U^1\end{pmatrix}= \gamma \begin{pmatrix} 1&v\\v&1\end{pmatrix}\begin{pmatrix} 1\\0\end{pmatrix} \tag{5}$$

Now, looking at the acceleration $A'^\mu = \large \frac{dU'^\mu}{d\tau}$, in the stationary frame $F'$, we may notice that $A$ transforms also as a vector in a Lorentz transformation, so we have :

$$\begin{pmatrix} A'^0\\A'^1\end{pmatrix} = \gamma \begin{pmatrix} 1&v\\v&1\end{pmatrix}\begin{pmatrix} A^0\\A^1\end{pmatrix}= \gamma \begin{pmatrix} 1&v\\v&1\end{pmatrix}\begin{pmatrix} 0\\w\end{pmatrix} \tag{6}$$

So, we have : $$A'^1 = \frac{dU'^1}{d\tau}= \frac{d(\gamma v)}{d \tau} = \gamma w \tag{7}$$

That is :

$$\frac{d(\gamma v)}{dt} = w \tag{8}$$

See also this lecture of Susskind

Answer 2

Landau's hint goes as follows:

Four-velocity is $$ u^{i} = \frac{dx^{i}}{ds} = \gamma_{v}(1,\frac{1}{c}\mathbf{v}), $$ where $$ \gamma_{v} = \frac{1}{\sqrt{1-\frac{v^2}{c^2}}}. $$ We have used $$ ds = \frac{cdt}{\gamma_{v}}. $$ To proceed, we note that $$ \dot{\gamma}_{v} = \frac{\gamma^{3}_{v}}{c^2}\mathbf{v}\cdot\dot{\mathbf{v}}, $$ therefore $$ w^{i} = \frac{dw^{i}}{ds} = \frac{\gamma_{v}}{c}\left\{\dot{\gamma}_{v}(1,\frac{1}{c}\mathbf{v})+\gamma_{v}(0,\frac{1}{c}\dot{\mathbf{v}})\right\} = \frac{\gamma^{2}_{v}}{c^2}(\frac{\gamma^{2}_{v}}{c}(\mathbf{v}\cdot\dot{\mathbf{v}}),\frac{\gamma^{2}_{v}}{c^2}(\mathbf{v}\cdot\dot{\mathbf{v}})\mathbf{v}+\dot{\mathbf{v}}). $$ Considering only one-dimensional motion (along $x$), using a initial reference system in which the particle with $v=0$ (but $\dot{v}=w\ne0$) at this moment, because $\gamma_{v}=1$, we have $$ w^{i} = (0,\frac{w}{c^2},0,0). $$ If the current velocity is not vanishing, we have $$ w^{i} = \frac{\gamma^{2}_{v}}{c^2}(\frac{\gamma^{2}_{v}}{c}v\dot{v},\frac{\gamma^{2}_{v}}{c^2}v^2\dot{v}+\dot{v},0,0) = \frac{\gamma^{4}_{v}}{c^2}(\frac{v}{c}\dot{v},\dot{v},0,0). $$ The scalar $w^{i}w_{i}$ is $$ w^{i}w_{i} = -\frac{\gamma^{6}_{v}}{c^4}\dot{v}^{2}. $$ Landau hints that it should be a constant under Lorentz transformation, i.e., $$ -\frac{\gamma^{6}_{v}}{c^4}\dot{v}^{2} = -\frac{w^2}{c^4}, $$ therefore $$ \gamma^{3}_{v}\dot{v} = w, $$ which is equivalent to $$ \frac{d}{dt}(\gamma_{v}v) = w. $$ Solving this differential equation with initial condition, we reach Landau's solution.