How to interpret a Green's function containing a branch cut from $-\infty$ to $+\infty$ at negative imaginary frequency?

Question

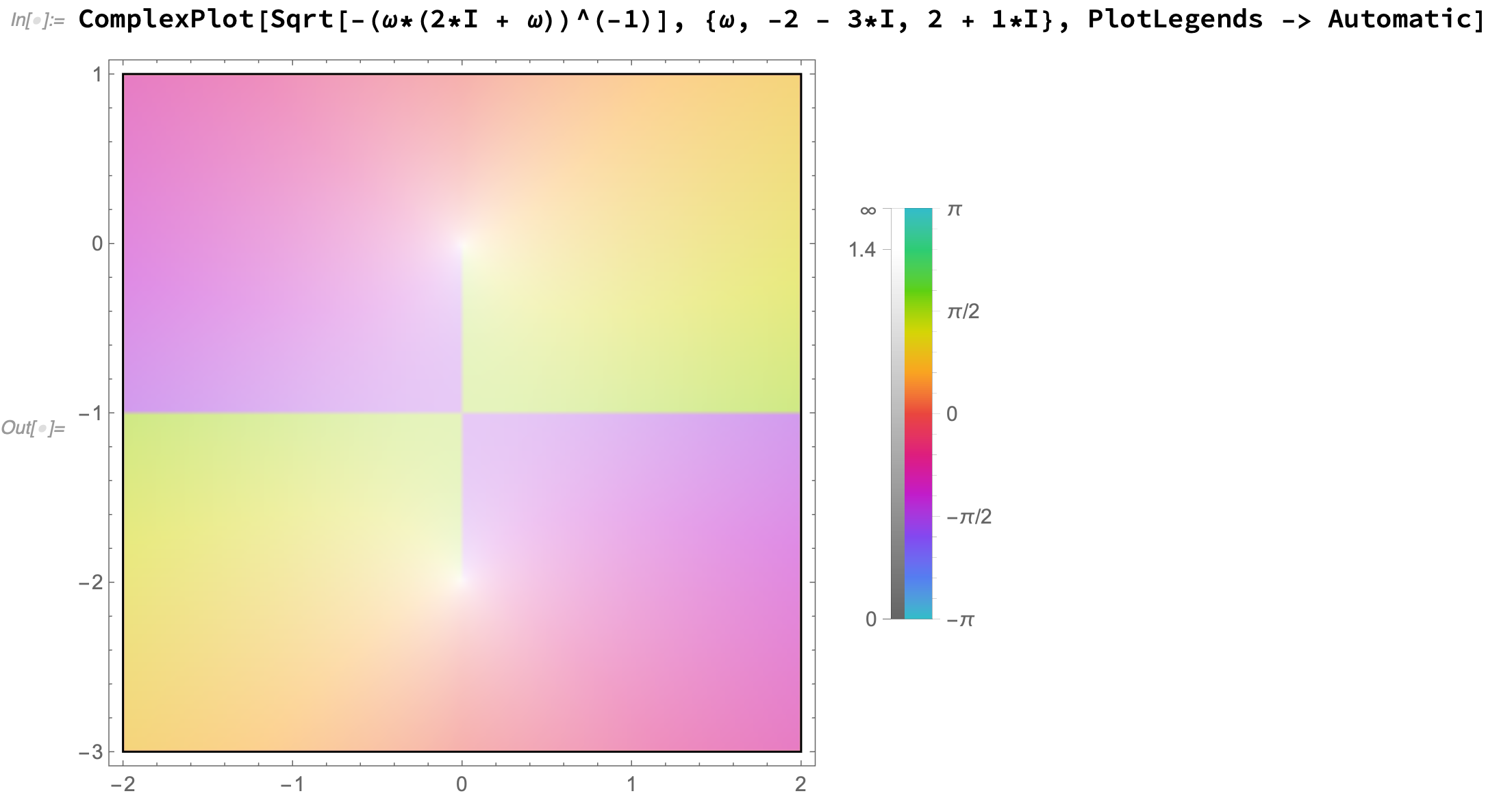
From some loop calculation in an EFT, I have found the Green's function containing this square root: $$G(\omega, k)\sim \sqrt{\frac{D k^2 \tau- (i+\tau \omega)^2}{(i+\tau \omega)^2(-D k^2 \tau + \omega \tau (2 i + \tau \omega))}}$$ I need to find $G(t, k)$. However, Mathematica command "InverseFourierTransform" cannot do this. Therefore, I restrict myself to the special case $k=0$ $$G(\omega, 0)\sim \sqrt{\frac{-1}{\omega \tau (2 i + \tau \omega)}}$$ Even in this case, "InverseFourierTransform" doesn't work. By $3D$-plotting of the Re and Im parts of $𝐺(\omega,0)$, I found that its analytical structure is a bit strange: there are two branch cuts, one starting from $0$ to $-2i/\tau$ and the other from $-\infty-i/\tau$ to $+\infty-i/\tau$ (see below). I want to understand what is the meaning of a branch cut from -infinity to +infinity at a negative imaginary frequency? In particular, this becomes important when one wants to find $G(t,0)$ by closing the integral contour in $G(t,0)=\int_{-\infty}^{+\infty} G(\omega,0) e^{- i \omega t}$ on the lower half of the complex frequency plane.

If anyone could comment on this issue I would be grateful.

Answer 1

Up to rescaling, you are studying the function: $$ \hat G(\omega) = \frac{1}{\sqrt{\omega(\omega+i)}} $$ The natural cut to choose is the segment $[0,i]$. It's what the program tried to do, but due to automatic choice of the branch for the square root, there are the artificial branches that appeared. The Fourier transform: $$ G(t) =\int \frac{d\omega}{2\pi}\hat G(\omega)e^{-i\omega t} $$ can be calculated using contour integration.

For $t<0$, you need to close the contour on the upper half complex plane, so you get $$ G(t) = 0 $$

For $t>0$, you need to close the contour on the lower half complex plane. You can deform it to "snugly" encompass the branch cut to get: $$ \begin{align} G(t) &= \frac{1}{\pi i}\int_0^1 \frac{e^{-xt}dx}{\sqrt{x(1-x)}} \\ &= -ie^{-t/2}I_0\left(\frac{t}{2}\right) \end{align} $$ with $I_0$ the modified Bessel function of the first kind (courtesy of Wolfram Alpha...).

Hope this helps.