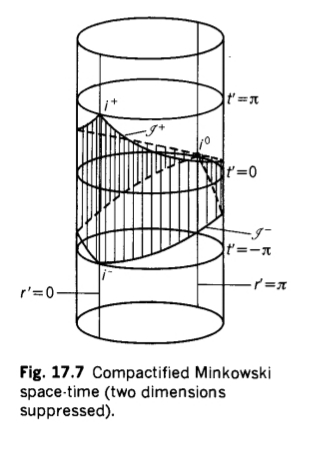
I'm studying Ray D'Inverno's book "Introducing Einstein's relativity". I'm having trouble understanding Fig. 17.7 (pag. 236), which is an illustration of compactified Minkowski spacetime. Especially, I don't understand how $r'$ coordinate is measured on the cylinder: there are two vertical straight lines on the cylinder labelled $r' = 0$ and $r' = \pi$, but if I want to individuate, say, $r' = \frac{\pi}{3}$, how should I proceed? Thank you for your answers.
$\begingroup$
$\endgroup$
6
-
$\begingroup$ I've included it just now. $\endgroup$– Al01Commented Sep 21, 2023 at 19:56
-
$\begingroup$ Are you wondering which way around you should go? It wouldn’t matter as far as I can tell. $\endgroup$– GhosterCommented Sep 21, 2023 at 20:02
-
$\begingroup$ I believe $r'$ is considered as an angular coordinate. In the book, on the page preceding this figure, he states that constant $t$ coordinate slices have the topology of a three sphere, so in that case $r'$ would be treated as an angular coordinate. $\endgroup$– Daniel WatersCommented Sep 21, 2023 at 20:04
-
$\begingroup$ So do the points on the cylinder with $r' = c$, where $c$ is a constant and $0 \leq c \leq \pi$, lie on the vertical straight line making an angle with $r'=0$ equal to $c$ when measured from the axis of the cylinder, no matter the direction (clockwise or counterclockwise)? $\endgroup$– Al01Commented Sep 21, 2023 at 20:10
-
$\begingroup$ Yes, except the direction (clockwise or counterclockwise) does matter. Just pick one of these directions for angles between $0$ and $\pi$. Then the other direction is for angles between $\pi$ and $2\pi$. $\endgroup$– GhosterCommented Sep 21, 2023 at 20:50
|
Show 1 more comment
2 Answers
$\begingroup$
$\endgroup$
Although in the cylinderical diagram the time coordiante $t'$ has the range $$ -\infty < t' < \infty$$
the relavent range is only from $$ -\pi< t' < \pi $$
As such, the conformally compactified region of the Minkowski spacetime is only the diamond region of the cylinder with boundaries ($\mathscr{I}^{+}, \mathscr{I}^{-}$).
In addition we pick any one side of this diamond with $ 0 \leq r' \leq\pi$. As two dimensions are suppressed, on its own the compactified region would appear like shown in the image below.
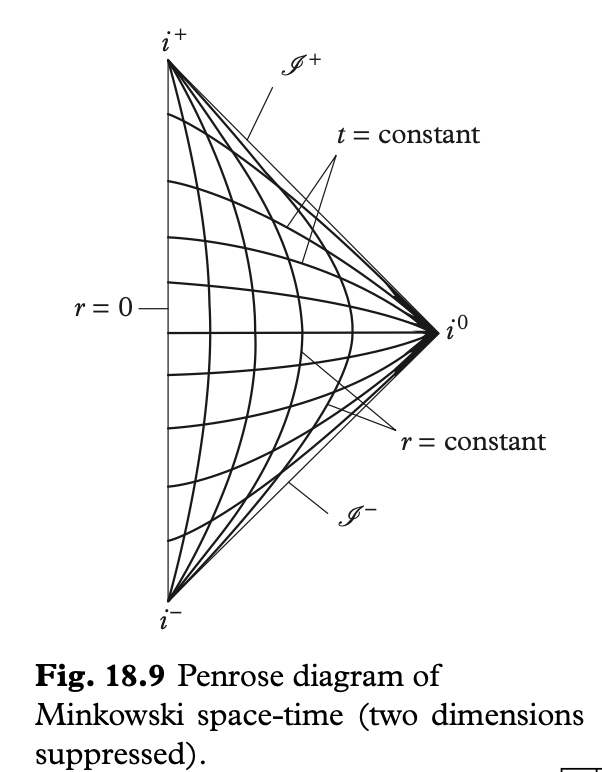
In this image $r' \in (0, \pi)$ and $r' = \pi/3$ would lie at a distance 1/3 from the LHS along the lines $t = $ const.
$\begingroup$
$\endgroup$
1
$r'$ is just the angular position on the cylinder's surface. In the Penrose diagram (Fig. 17.9), it's the horizontal coordinate.
From comments, I think you're confused because there is a second copy of the Penrose diagram running from $0$ to $-π$ (or $π$ to $2π$). That's a peculiarity of the 1+1 dimensional case. In $d+1$ dimensions, the compactification lives on the surface of $S^d\times \mathbb R$, and the points at a fixed $r'$ ($0<r'<π$) form a $(d-1)$-sphere of radius $\sin r'$. If $d>1$, that's a continuous surface, and you can cover it with the $θ,φ$ of the original Minkowski space. If $d=1$, it's two points, and it's easiest to allow $r'$ to take negative values—the same thing that happens if you try to put polar coordinates on 1D Euclidean space.
-
$\begingroup$ When you say "the horizontal coordinate" do you mean simply that I have to draw on Fig. 17.9 (that one showing the Penrose diagram) a vertical straight line at a distance from the left vertical edge equal to the value of the coordinate? As if I had a cartesian (x,y) 2D coordinate system? $\endgroup$– Al01Commented Sep 22, 2023 at 6:57