Measurement Error Analysis in Gaussian distribution

Question

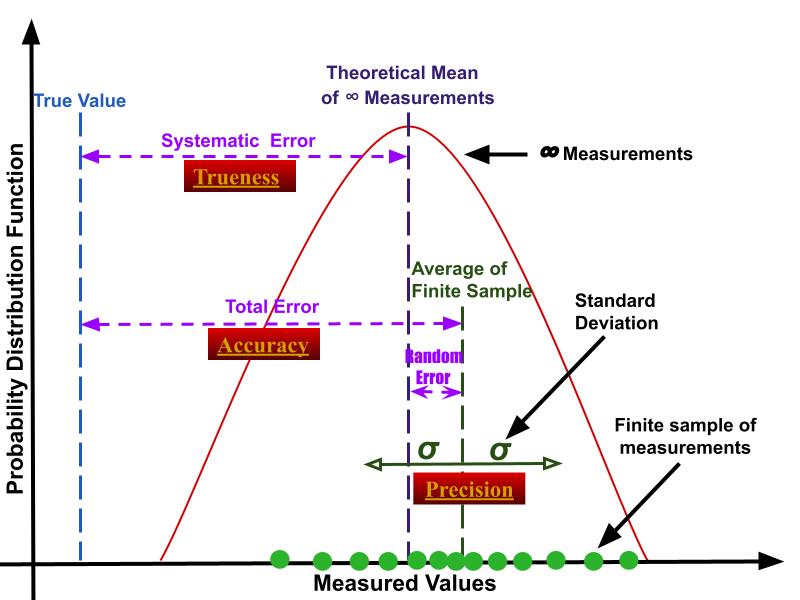
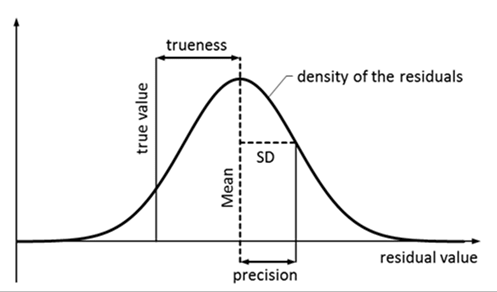
I am new to statistics and recently learned about ISO guidelines for Accuracy & Precision and Uncertainty & Error. But there are some graphs of probability distribution I found on internet which I am not able to grasp.

image Source

Q. In this graph(above) if systematic error is zero then average value will be the true value! How's that possible? i.e. if we take measurements under a condition of zero systematic error , average of whatever we measured will be equal to true value but aren't there some random error in average of measurement? And why random error here is described with respect to measured value not to mean of measured value?

Image Source

In first graph total and random errors are described wrt measured value not to mean of measurements and here in 2nd graph accuracy and precision are related to mean of measurement, no concept of measured value.
Could someone post a single graph ( adding some more details in first graph about accuracy precision and Trueness) of probability distribution in which all the parameters of measurement like Random error, Systematic error, Total error, Uncertainity, Accuracy, Precision and Trueness are described? It would also be fine if you upload a photo a hand-drawn graph in copy rather than a printed one.

Edit:- I have made a graph could someone check it that it is correct or not according to ISO definations of the terms used in graph? (Link of the definations are provided above in the question)

Answer 1

The standard model is $$ y_{meas} = y_{true} + \epsilon $$ where $\epsilon \sim N(\mu_{bias}, \sigma_\epsilon)$ is normally distributed. In this model $\mu_{bias}$ is the systematic error. If it is zero, the expected value $E[y_{meas}] = \bar Y$ is equal to the true value $y_{true}$ in the limit $N\to \infty$. This can be shown by the central limit theorem, which states that the standard deviation of the average value scales as $\sigma_{\epsilon}/\sqrt{N}$ -- for $N\to \infty$ this tends to zero. Instead, if we have a finite $N$, the measurement error of the average is of cause finite as well -- as you correctly stated in your question.

The accuarcy is (most often) the difference between the average value and the true value. Thus, it is nowadays often called bias, because "accuracy" was formerly used to describe several different things. Also, the terms total error, systematic error, and trueness are not uniquely defined -- at least this is stated in some ISO norms and therefore it is discouraged to used these terms.

In short:

• accuracy is (most often) used to mean bias
• precision is used to mean standard deviation of "some statistics" $T$.
• A statistics $T$ is any procedure, which uses the data. E.g. if you define a function using the measured data $T=f(y_{1}, y_{2}, \ldots)$ you get a statistics. Note that it would formally be fine to choose a constant as the function output.
• uncertainty is usually used to mean standard deviation. However, there is also the term extended uncertainty, which uses some constant factor $k$ and multiplies the standard deviation. Often (not always) $k=2$.

Personally, I prefer to be very explicit. Often I read something like "the diameter is 1mm, and the uncertainty of the measurement is 0.01mm". This is (at least) ambitious, because we did not measure the average value, but calculated it from our measurements. Thus, I would prefer to read "the average diameter is $\bar Y = 1mm$, and it's uncertainty $Sd[\bar Y]=0.01mm$". In this sentence it is clear that we state the uncertainty of the average value $\bar y$, and not the uncertainty of a single measurement $y_i$.