Understanding enthalpy and gibbs energy changes in a reaction

Question

I was looking at the enthalpy change for water-splitting reaction:

$$ \Delta H^o_R = [\Delta H^0_{H_2(g)} +\frac{1}{2}\Delta H^0_{O_2(g)}]-\Delta H^0_{H_2O(l)} = 285.83 kJ/mol$$

According to the book "Thermal physics"by Schroeder; at constant T and P; if there are no other forms of work on the system besides compression/expansion, then $\Delta H^o_R = Q$. However, when there are other forms of work being done we then have $\Delta Gº_R \leq W_{other}$ and $\Delta H^o_R = Q + W_{other}$. The value for the Gibbs free energy in this case is $ \Delta Gº_R = 237 kJ/mol $. We can relate $\Delta G$ and $\Delta H$ by $\Delta G =\Delta H -T\Delta S$ .

My confusion arises first from reading that the gibbs free energy is the work we need to drive the reaction, say electrical work. However, the enthalpy change shows that the energy required could be done via heat and/or another form of work is higher than that of the Gibbs free energy? My guess so far is that we can take some energy from the environment for "free", but what happens when we drive this reaction only via heat, such that $\Delta H = Q$, would $\Delta G = 0 $ ?

Answer 1

We know that Nature tends to drive forward any process that would maximize entropy. The formation of reaction products that have a lower internal energy level than the reactants ($\Delta U<0$) seems promising, as the energy released could heat the surroundings, thus increasing the entropy of the rest of the universe.

Oh, but also, we should consider the fact that denser reaction products would also be favored, because then the rest of the universe could have a larger volume, which also provides greater entropy. The greater the surrounding pressure, the more this is important (or, alternatively, we could recognize that pressure is the conjugate variable to volume), so we have an additional relevant term $P\Delta V$. This is a work term (specifically, mechanical compression work).

And let's also consider that reaction products with a larger entropy would also fulfill the primary goal. The corresponding term, noting that temperature is the conjugate variable to entropy, is $-T\Delta S$. This is a heat term.

An infinite number of similar arguments could be made for other forms of work such as surface energy work, electrical work, magnetic work, etc., but let's ignore those for now (we could always incorporate them if we wished) and consider the total $\Delta U+P\Delta V-T\Delta S$. This is the change in the Gibbs free energy $G$ that we use so often: Reactions at near-constant pressure and temperature are spontaneous when $\Delta G<0$.

This review provides a basis for understanding why dissociation reactions can generally be driven by heating, for instance: The greater entropy provided by the products (especially gases) outweighs the energy drawn from the surroundings to break the required bonds.

Further intuition can be gained from the graphical Ellingham diagram, which shows what temperatures and partial pressures are needed to drive formation and dissociation reactions:

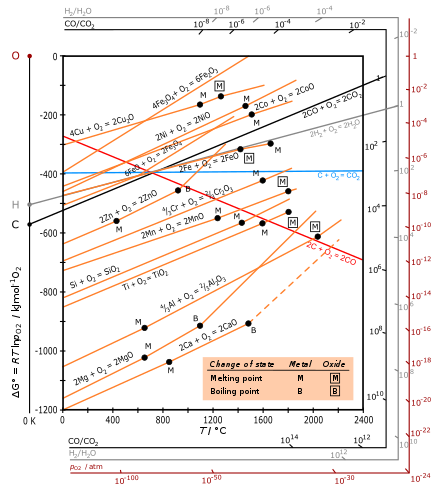
All those upward curves show the increase in $\Delta G$ as elements combine with oxygen to form compounds; the slope is generally positive because a gas is disappearing ($\Delta S<0$). (Note especially the horizontal line for carbon dioxide, whose formation leaves the number of moles of gas unchanged, and the downward line for carbon monoxide, which provides two moles of gas for each one mole consumed.) When $\Delta G$ changes sign from negative to positive, dissociation—rather than formation—is predicted.