0
$\begingroup$

I am currently confused about things I read about special relativity (I'm a CS major and not as well-versed in physics as I would like to be).

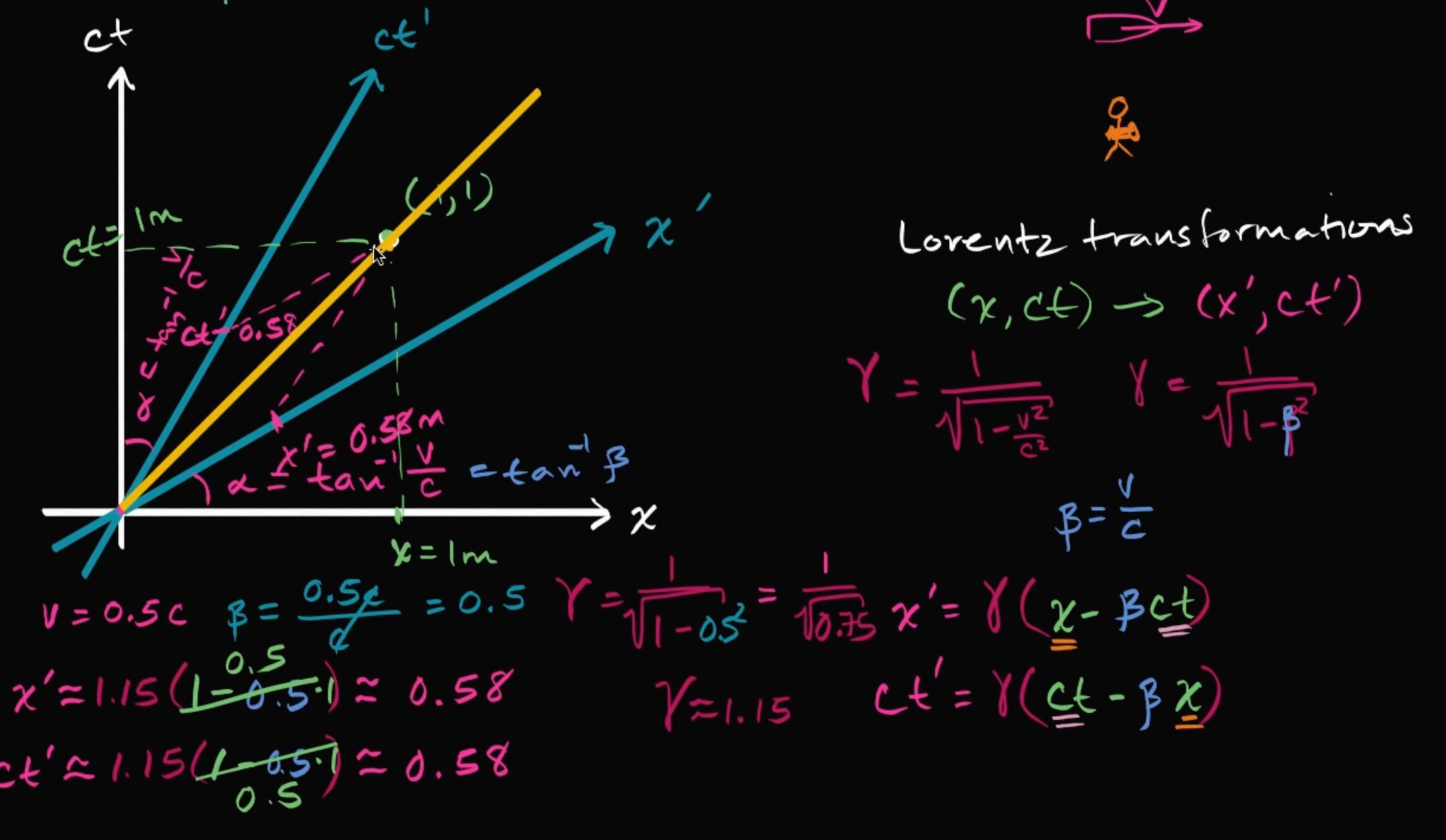
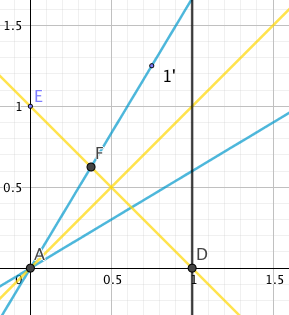
I just watched a video on evaluating a Lorentz transformation of Khan Academy (link below), here's a screenshot I took of the final few minutes. It essentially says that if a stationary person would shine a light on an asteroid that is x=1 away, the stationary person would observe that asteroid to light up at ct=1, whereas the person going at 0.5c in a spaceship sees this asteroid light up at 0.58ct and 0.58x on the x-axis.

The way I interpreted this is that after 0.58ct proper time (on the spaceship), this person sees that asteroid light up whereas this seemed to happen after 1ct in stationary time. So far so good.

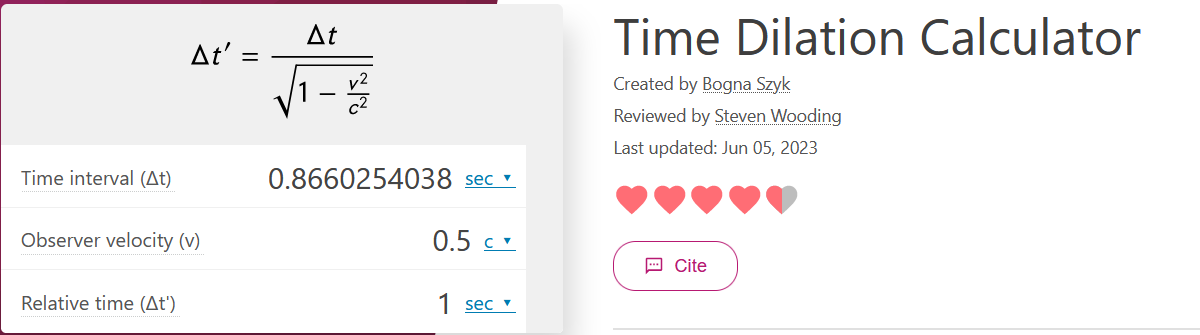
enter image description here

Now I also found a tool that lets me calculate Time Dilation given the velocity of a spaceship on OmniCalculator. It tells me that for 1ct in stationary time, we would have only 0.86ct pass in proper time on the spaceship going at 0.5c. This is where I got confused. Am I mixing things up? Why are the numbers 0.86 and 0.58 different? I would have expected them to be the same. Shouldn't they be? I'm almost certain that I am mixing things up.

Also, what does it mean for ct' in the Khan Academy example to be at 0.58 as well? Does that mean the person in the spaceship going at 0.5c thinks that the asteroid that lights up is closer than that the stationary person does?

Any clarification would be appreciated, thank you.

enter image description here

Khan Academy video: https://www.youtube.com/watch?v=G-aTU0RXJww

Improve this question
$\endgroup$
0

2 Answers 2

Reset to default
0
$\begingroup$

If want to be successful in solving SR problems, clarity is paramount.

So first: define reference frames. $S$ is "stationary", and $S'$ is moving, and their origins are of course the same.

Then you have specific events, labeled by $(t, x)_{frame}$, which are points in space-time.

The 1st event is the emission of the light:

$$E_1 = (0, 0)_S = (0, 0)_{S'}$$

The second event is not clear in the question. Is it when/where $S$ sees the asteroid illuminated, or is it when/where the light strikes the asteroid? I'll assume the latter:

$$ E_2 = (1, 1)_S $$

Now you can Lorentz transform that:

$$E_2 = (\gamma(t-vx), \gamma(x-vt))_{S'} = (\gamma(1-v), \gamma(1-v))_{S'} = (\frac 1 {\sqrt 3}, \frac 1 {\sqrt 3})_{S'}$$

Rather then applying Lorentz factors, which can lead to confusion thanks to the relativity of simultaneity, just look at the coordinate time difference in the primed frame:

$$ \Delta t' = t'_2-t'_1 = \frac 1 {\sqrt 3} $$

It's clear from $E_2$ in $S'$, that the Lorentz transform account for both time dilation ($\gamma$), and the motion of the asteroid $(1-v)$.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you for your answer, this is already very helpful. Probably a stupid question, but where does 1/sqrt(3) come from? Edit: it's probably just short for 1.15 * (1-0.5*1) isn't it? Could you tell me what the 'x' stands for in the event frame? That part is still unclear, does that mean the ship (S') traveled x units before it saw the asteroid being struck? $\endgroup$
    – Frans
    Commented Aug 2, 2023 at 14:48
  • $\begingroup$ $[\gamma(1-v)]^2 = \frac{(1-v)^2}{(1-v)(1+v)} = (1-v)/(1+v) = \frac 1 2 / \frac 2 3 = 1/3$ $\endgroup$
    – JEB
    Commented Aug 2, 2023 at 22:32
0
$\begingroup$

you have to complete the picture, a ray coming from x=1 meets x=0 at ct=1 (E in picture) and x'=0 at F x'=ct'=0.58cMinkowsky diagram the point t'=0,86 is on the horiontal line from E

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.