I hope my question does not break the rules. I can't understand how this statement can be true in the book which I've mentioned:
I cannot undestand why it's true because if I have two point charges with same sign and same magnitude then the electric field should be zero at point P:
But according to gauss's law it's not zero and it equal to electric field due to one of these point charges. So this electric field is only caused by the charge inside the closed surface, not the charges outside it!
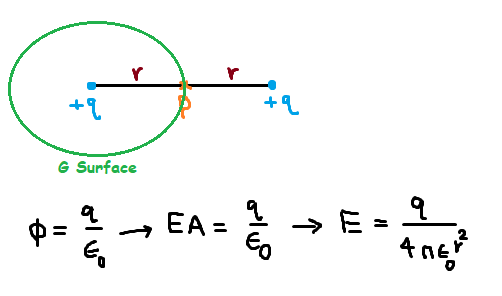
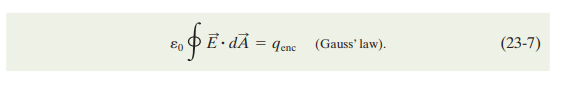You wrote as a comment that: "I know that electric field due to charges outside of the closed surface doesn't contribute to net flux but I don't see how it answers my question."
OK, first take a single unit point charge a draw and sphere around it. Its flux is the integral $\Phi_0 = \oint \frac{1}{4\pi} \frac{\mathbf r^0}{r^2}\cdot d\mathbf A $ but from the radial symmetry you have since $d\mathbf A = \mathbf r^0 dA$ and $A=4\pi r^2$ therefore $\Phi_0 = 1$, one unit charge.
Now take an arbitrary closed surface $\mathcal A$ containing a single unit charge and draw a small enough sphere $\mathcal S$ that is completely within that surface $\mathcal A$ and also contains the same unit charge. Also let $\mathcal B $ denote the surface of the rest of the volume, $\mathcal B = \mathcal A - \mathcal S$. The flux through $\mathcal S$ is $1$ while through $\mathcal B$, as you already know, is $0$, hence the flux through $\mathcal A$ is $1$.
Now if you have an arbitrary charge distribution break that up into small almost point like infinitesimal pieces and add up their individual infinitesimal fluxes associated with the closed surface that contains all of them. The result it is Gauss's law.
Yes, the net electric field at point P is 0 and Gauss law is not violating it. What Gauss Law means is that if you choose a 3 dimensional closed shape and the net electric field incident on a small elemental of area $ dA$ on the surface of this volume is E , the flux through this closed shape is defined as the sum of $\vec E \cdot \vec{dA} $ at all points on the boundary surface of this shape. In the scenario which you have taken , the field at point P is most certainly equal to 0 , however at other points on the boundary of this sphere this field is not 0. Overall if you sum $\vec E \cdot \vec{dA} $ , you are bound to get this equal to $ \frac{q_{enclosed}} { e_0 }$ according to Gauss law.
Also if you want to find the field at point P using Gauss law , I think it is not possible to do so because the field at each point on a spherical Gaussian surface like you have taken in this scenario is different and it would be tremendously difficult to calculate it without using Coulomb’s law. Instead using Coulomb’s law in such scenarios is very easy comparatively to calculate the field at all points in space.
Hope this helps!
