Apparant contradiction in magnetic vector potential of current loop

Question

I'm studying electromagnetism and I came upon an apparant contradiction in the calculation of the magnetic field above the center of a current loop.

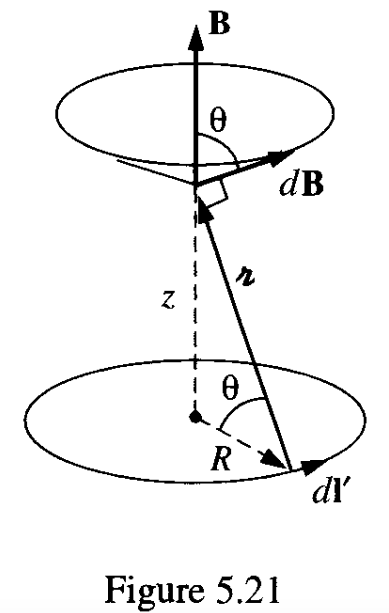
The setup is given in the image, I want to calculate the magnetic field in a point above the center of the loop, at height $z$. When this is done with Biot-Savart, you get a non zero value for $B(z)\hat{z}$

I want to calculate it by first calculating the vector potential $A$ and then taking the curl of it. However when it is done in this way:

$$ A = \frac{\mu_0 I}{4 \pi} \oint \frac{d\vec{r}}{|\vec{r}-\vec{r}'|}$$

But this distance in the denominator is a constant, in the case of the loop with constant radius $R$. And thus the whole integral evaluates to $0$, so the resulting vector potential $A$ is also zero, and we get no magnetic field $B$.

How is this possible?

Answer 1

Your calculation gives A at the point of interest, as well as directly below and above it, A as a function of z. How A varies in the z-direction does not relate to the z-component of its curl. A will vary in the horizontal plane, so it can have a non-zero vertical curl.

Answer 2

The $\vec {dr}$ should be $\vec{dr'}$. Then the curl acts only on the $\vec r$ in the denominator.