Generalization of Bargmann's theorem

Question

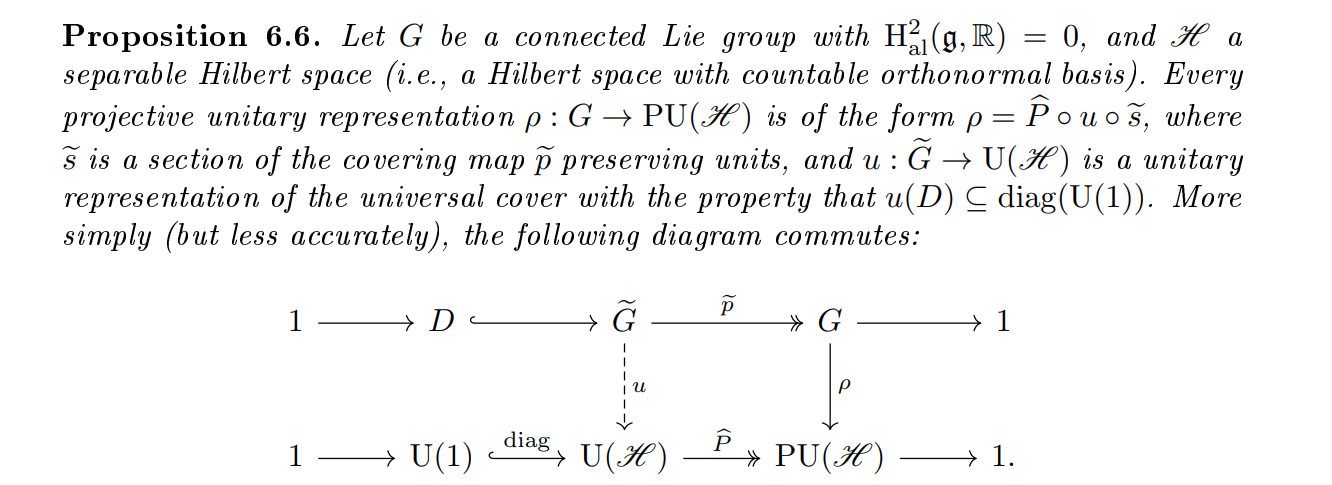
Bargmann's theorem is usually stated for a simply connected Lie group with vanishing second Lie algebra cohomology $H^2(\mathfrak{g},\mathbb{R})$. I found a generalization of this result in a thesis https://www.math.ru.nl/~landsman/Nesta.pdf, which accounts for $G$ being not simply connected. The theorem reads:

This is strongly related to @ACuriousMind's comment on the question Why exactly do sometimes universal covers, and sometimes central extensions feature in the application of a symmetry group to quantum physics?. It should be precisely point 2 of his answer. My question is: does this theorem actually really reduce to Bargmann's theorem for $G$ simply connected? In that case $D=\{e\}$ and $p=\text{id}$ as $\tilde{G}=G$. But in Bargmann's theorem, there's a $U(1)$-extension, not an extension by ${e}$. Can one obtain Bargmann's theorem from this result directly, or they are not a-priori related?

EDIT: Maybe a more well-posed question is the following: Is the case 2 a generalization of case 1 in @ACuriousMind 's answer, or they are unrelated? Can one obtain case 1 as a special case of case 2?

Answer 1

Yes, of course this reduces to Bargmann's theorem: Bargmann's theorem says that when $H^2(\mathfrak{g},0) = 0$, then every projective representation of a simply-connected $G$ can be lifted a unitary representation of $G$. While the proof of Bargmann's theorem involves talking about central extensions by $\mathrm{U}(1)$, the statement of the theorem does not.

The theorem you quote here says the same: When $D = 1$ and $\tilde{G} = G$, i.e. $G$ is simply-connected, it says that the projective representation $\rho$ of $G$ can be lifted to the unitary representation $u$ of $G$.