Why are we interested in the dimensional analysis/power counting in string theory?

Question

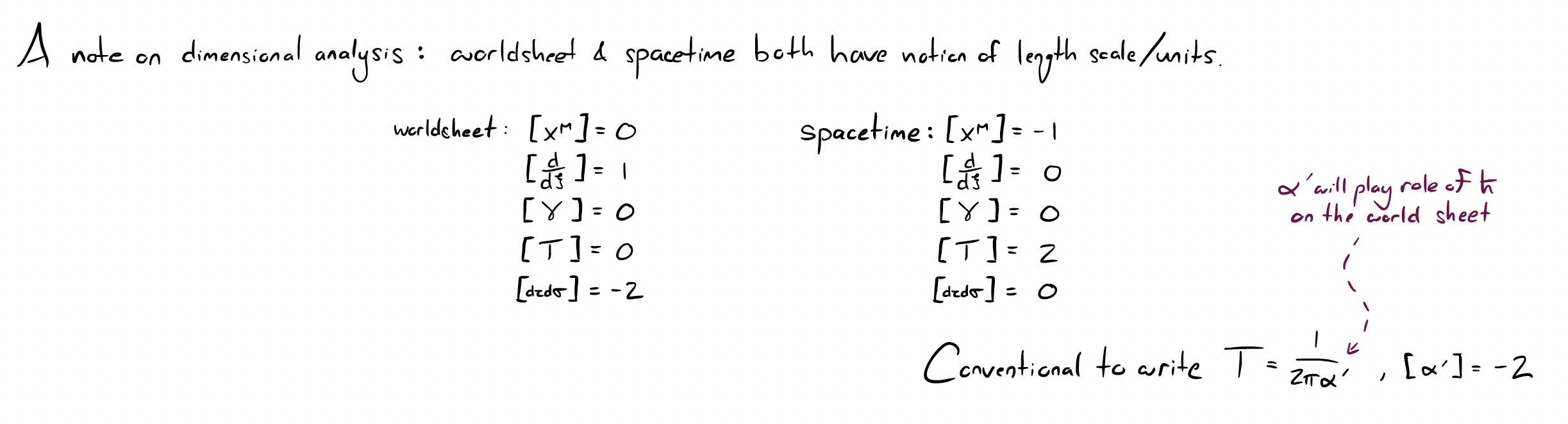
I'm learning bosonic strings on my string theory course; here is part of my notes about the dimensional analysis on the world sheet $\Sigma$ and the spacetime manifold $\mathcal{M}$:

I learned this in the context of figuring out which terms we could add to the Polyakov action:

$$ S_p[\gamma_{ab},X^\mu] = -\frac{T}{2}\int_\Sigma d\tau d\sigma\sqrt{-\gamma}\gamma^{ab}\frac{\partial x^\mu}{\partial\xi^a}\frac{\partial x_\mu}{\partial\xi^b} $$

to maintain Weyl invariance. I remember the consequence is the 'cosmological constant term': $$ S_C = \lambda\int_{\Sigma} \sqrt{-\gamma}d\tau d\sigma $$ cannot be added, but the Einstein-Hilbert term: $$ S_{EH} = \int_{\Sigma}R(\gamma) \sqrt{-\gamma}d\tau d\sigma $$ can be added.

I don't quite understand if this has anything to do with power counting, and if so, how do we interpret the dimensions for $\Sigma$ and $\mathcal{M}$? I remember in QFT dimensional analysis/power counting is used to determine whether a given Lagrangian is renormalizable. Is the idea of power counting similar here in string theory (like doing renormalization on the world sheet / embedded spacetime)?

Answer 1

The worldsheet action should be conformally invariant. A simple-to-check necessary condition for conformal invariance is global scale invariance. Dimensional analysis provides a way to check for global scale invariance. If the action is invariant after rescaling the fields, coordinates, derivatives, etc, by their scaling dimension, then the action has global scale invariance. To state the same thing in a different way, the action has global scale invariance if it does not have any dimensionful coupling constants.

You can see using the worldsheet dimensional analysis rules you've written down that the Polyakov action is scale invariant after accounting for the scaling of $d/d\xi$ and of the measure $d\sigma d\tau$. Alternatively, you can see that the coupling constant $T$ associated with this term is dimensionless.

A similar analysis shows the cosmological constant term breaks scale invariance, while the Einstein-Hilbert term does not. Since the cosmological constant term does not have global scale invariance, it cannot possibly have full conformal invariance, so we conclude the cosmological constant term cannot be in the worldsheet action in a consistent conformally-invariant string theory.