Why in Stern-Gerlach experiment atoms emitted in the beam have kinetic energy $2kT$?

Question

The book I am following to study Stern-Gerlach experiment has written -

... from kinetic theory, the velocity $v_x$ of an atom of mass $M$ is evaluated by setting \begin{equation} \frac{1}{2}Mv_x^2=2k_BT. \end{equation}

The reason for putting $2k_BT$ at RHS is described as:

... atoms in the oven have kinetic energy $\frac{3}{2}k_BT$; but the atoms emitted in the beam have kinetic energy $2k_BT$. The reason is that the more energetic atoms hit the walls of the oven more frequently and hence have a higher probability of falling on the hole in the wall through which the beam is emitted.

But that doesn't make any better sense to me. Is there any reason for multiplying $\frac{1}{2}k_BT$ by $4$? Or the logic is entirely different?
I also saw something called EFFUSION in this context. But never heard of it before.

Answer 1

You are referring to rms (root mean square) velocity.

In any beam of particles there are two velocity distributions that may be of interest. Let's call them $f_1$ and $f_2$.

1. If we propose a region R of space containing some long section of the beam then:

(number of atoms in R at some instant of time with velocity between $v$ and $v+dv$) = $f_1(v) dv$

2. If we propose a plane P which the beam passes through and some interval of time $\Delta t$ then:

(number of atoms passing through P during $\Delta t$ with velocity between $v$ and $v+dv$) = $f_2(v) dv$

Many textbooks simply go straight to $f_2(v)$ here and don't really make it clear that both distributions are well-defined and each has its uses. Anyway one can show from some simple kinetic theory that $$ f_2(v) \propto v f_1(v) . $$

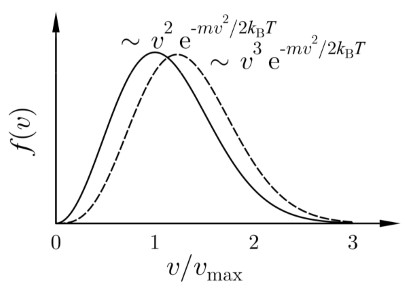
In a Stern Gerlach experiment you typically have a beam of atoms produced by free emission from a small hole at low density (this is called effusion) from a chamber in thermal equilibrium at some temperature $T$. Basic kinetic theory then gives $$ f_1(v) \propto v^2 e^{-m v^2/2kT} $$ and $$ f_2(v) \propto v^3 e^{-m v^2/2kT}. $$ In either case the proportionality constant can be obtained by normalizing the probability distribution.

Now we can calculate the mean square velocity.

For distribution 1, $$ \frac{1}{2} m \langle v^2 \rangle = \frac{1}{2} m \frac{ \int_0^\infty v^4 \exp(-mv^2/2kT) dv } {\int_0^\infty v^2 \exp(mv^2/2kT) dv} = \frac{3}{2} k T $$

For distribution 2, $$ \frac{1}{2} m \langle v^2 \rangle = \frac{1}{2} m \frac{ \int_0^\infty v^5 \exp(-mv^2/2kT) dv } {\int_0^\infty v^3 \exp(mv^2/2kT) dv} = 2 k T . $$

Which distribution to use depends on what kind of experimental observation one is making. In Stern Gerlach case one usually places a screen and measures how many atoms arrive on that screen during some interval of time, so the second distribution is the right one to use. If instead one detected the atoms by amount of fluorescence at any given time owing to illumination of a given length of the beam, then distribution 1 would be appropriate.

(As I say, many discussions of atomic beams omit case 1 here altogether, but it is better to be aware of both.)

Answer 2

Thet follow the Maxwellian Velocity distribution. So you can get an average from there.

Answer 3

You need to consider a hole which is small so that the equilibrium of gas in the container is not disturbed.

In a given time $\delta t$ all molecules in the volume shown in grey at travelling at a speed $v$ with $AX = v \,\delta t$ have a chance of escaping through hole $X$.
However, there are more molecules with a higher speed $V$ which can escape from the hole in the same time.
So the distribution of the speeds of molecules escaping from the hole is different from the distribution of speeds of molecules in the container as shown below.

Note that $v_{\rm max}$ is the speed for the Maxwell-Boltzmann distribution at which the distribution function for molecular speeds, $f(v)4, is a maximum.

The solid line is the Maxwell-Boltzmann distribution and the dashed line is that for molecules which effuse from a small hole. Note the factor $v^2$ changing to $v^3$.

Standard texts then show, via somewhat difficult integration, that whereas the average kinetic energy of molecules in a container is $\frac 32 k_{\rm B}T$ the average kinetic energy of the molecules escaping from the container is $2 k_{\rm B}T$.