Projective representations and central extensions

Question

I have read this Q&A by @ACuriousMind about representations of a symmetry group in quantum mechanics and then also chapter 3 and 4 of Schottenloher's "A mathematical introduction to conformal field theory" it was cited in the comments of the question but I'm still left with some doubts (I posted another one here).

Just to give some context and notation we know that in QM the space of states is the projective Hilbert space $P\mathcal{H}$, so a symmetry transformation must act on it in the first place. Thus, given $G$ the symmetry group of the system, we have a projective representation $T \colon G \rightarrow \textsf{Aut}(P\mathcal{H})$ which is a group homomorphism. The equivalence classes, or operator rays, $T(g) \in \textsf{Aut}(P\mathcal{H})$ are induced, by Wigner's theorem, by either unitary and linear or anti-unitary and anti-linear operators $U(g) \in \textsf{Aut}(\mathcal{H})$.
$U \colon G \rightarrow U(\mathcal{H})$ (restricting the discussion only to unitary operators) is a group homomorphism just up to a phase $\omega$ $$U(g)U(h)=\omega(g,h)U(g,h)$$that in some cases can be made equal to 1, thus obtaining an ordinary representation. This depends on the second cohomology group of $G$ with coefficients in the group of phases $U(1)$: only if there are trivial cocycles (phases that can be written in a certain form) this is possible.

• Schottenloher states in page 48 that, given G the classical symmetry group

a lifting [of a projective representation of G to an ordinary one] exists with respect to the central extension of the universal covering group of the classical symmetry group.

that is also made clearer by the following example 3.8 about $SO(3)$ and $SU(2)$. At first this didn't coincide with what I learned (i.e. that a proj. rep. of $SO(3)$ is isomorphic to an ord. rep. of $SU(2)$ and not of its central extension) but then in chapter 4 in the proof of Bargmann's theorem he proves that for a simply connected group, as an universal cover like $SU(2)$ naturally is, the exact sequence $$1 \rightarrow U(1) \rightarrow E \rightarrow SU(2) \rightarrow 1$$ splits and so we can avoid the passage to the central extension and have directly and ordinary rep. of $SU(2)$.

Now, to do so he uses theorem 3.10: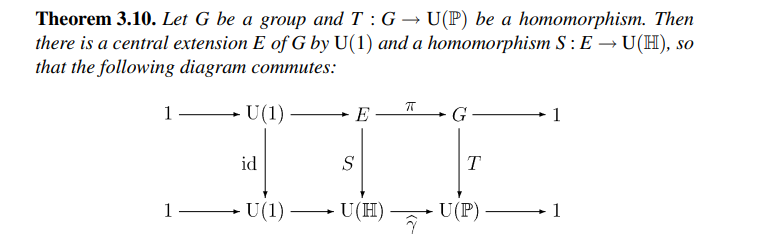

I don't get why this couldn't apply directly to $S0(3)$ as the group $G$ instead of doing this: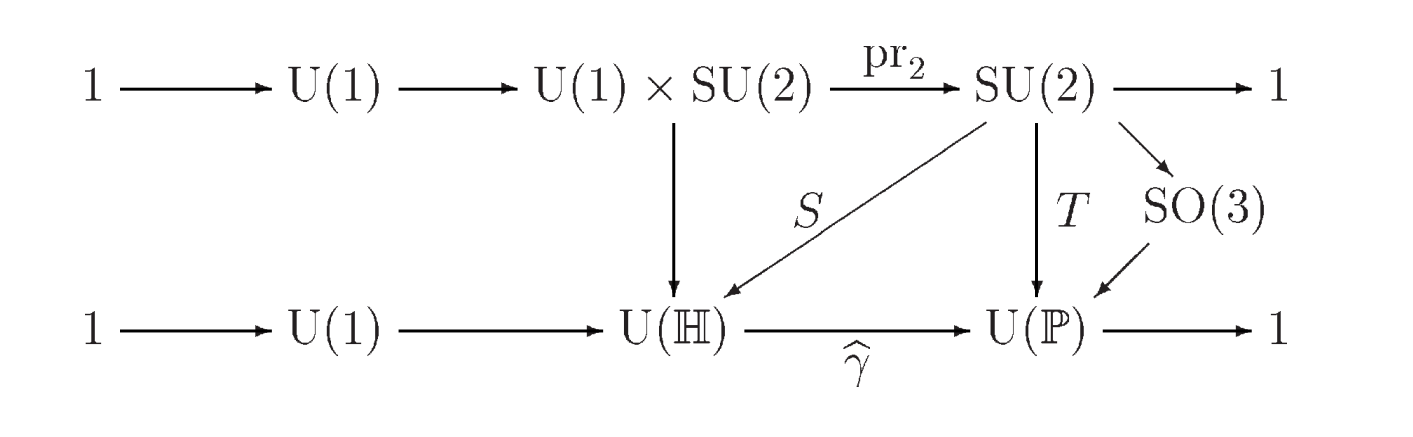
I will try to explain myself better: if we can take $SO(3)$ as the group $G$ why don't we (or at least the author) just refer to the ordinary representation $S \colon E \rightarrow U(\mathcal{H})$ of its central extension? Is it because that central extension $E$ of $SO(3)$ turns out to be exactly $U(1) \times SU(2)$? If that is the case what is the way to prove it and is it, as I suppose, general for all non simply connected groups?

Answer 1

Schottenloher's chapter 3 and 4 are somewhat "non-linear" in that a lot of chapter 3 only makes sense after you've seen what chapter 4 does with it. In this particular case, sure, theorem 3.10 as written would apply to $G=\mathrm{SO}(3)$, and you would get some $E$ as a central extension. But this is a useless observation, because we don't have any machinery that would tell us something about this $E$! What you really want is that $E$ splits as $E\cong G\times \mathrm{U}(1)$ so that the lifting $S:E\to\mathrm{U}(\mathbb{H})$ reduces to a proper linear representation $S_2 : G\to\mathrm{U}(\mathbb{H})$.

Chapter 4 presents Bargmann's theorem as the main tool for this - the sequence splits for a $G$ that is simply-connected and has $H^2(\mathfrak{g},\mathbb{R}) = 0$. As the universal cover of $\mathrm{SO}(3)$, $\mathrm{SU}(2)$ is simply-connected and so Bargmann's theorem will apply to it, which is why Schottenloher is using it in this example.

You never need to worry about applying theorem 3.10 to groups that aren't simply connected: Every projective representation of a group lifts to a projective representation of its universal cover and in fact all projective representations of the cover are also projective representations of $G$ because the universal cover is just a central extension by the fundamental group $\pi_1(G)$, so we can always apply this theorem to the universal cover to have a chance of applying Bargmann's theorem afterwards.

If "the universal cover is just a central extension by the fundamental group" doesn't convince you because we're actually talking about central extensions by $\mathrm{U}(1)$, not discrete fundamental groups, the rest of this answer is for you.

Explicitly, let $\tilde{G}$ be the universal cover, then we have the projection $$ \hat{\pi} : \mathrm{U}(1)\times \tilde{G} \to G$$ and when $G$ is compact and not the product of smaller Lie groups, $\pi_1(G)\cong \mathbb{Z}_m$ for some prime $m$. There is an embedding $\iota : \mathbb{Z}_m\to \mathrm{U}(1)$ mapping the elements of $\mathbb{Z}_m$ to the $m$-th roots of unity, and so we have a "diagonal" inclusion $$ \mathbb{Z}_m\to \mathrm{U}(1)\times \tilde{G}, \gamma\mapsto (\iota(\gamma), \gamma)$$ Let's call the image of this inclusion $A$. Then clearly $A$ lies in the kernel of the projection $$ \hat{\pi} :\mathrm{U}(1)\times \tilde{G}\to G, (x,g) \mapsto \pi(g)$$ where $\pi$ is the normal projection from the universal cover, and so we can turn the central extension

$$ 1\to \mathrm{U}(1)\times \pi_1(G) \to \mathrm{U}(1)\times \tilde{G} \overset{\hat{\pi}}{\to} G \to 1$$

into

$$ 1\to (\mathrm{U}(1)\times \pi_1(G))/A \cong \mathrm{U}(1) \to (\mathrm{U}(1)\times \tilde{G})/A \to G\to 1$$

where the thing in the middle is a "non-trivial" central extension of $G$ but only in the same way that $\tilde{G}$ is a non-trivial central extension of $G$, i.e. this doesn't have anything to do with the Lie algebra or its $H^2(\mathfrak{g},\mathbb{R})$ - on the level of the algebras, this extension is trivial.