Orbital motions

Question

Suppose two satellites are moving in circular orbits around the earth and then crash into one another.

My Question is this, how do we know that the bodies move in an elliptical orbit after the crash? Why not just remain in a circular orbit with a smaller radius (r)? Why not instead go into a hyperbolic or another orbit?

Answer 1

The conditions of the problem are not entirely clear from the diagram alone, so I am going to make some assumptions.

If you have two objects with masses $4m$ and $m$ colliding head on with velocities $v$ and $-v$ in a perfectly inelastic collision, then immediately after the collision you have a single object of mass $5m$ with momentum $3mv$. It's velocity is therefore $\frac 3 5 v$, which is less than $v$. Assuming $v$ is the sped required for a circular orbit at this height, then $\frac 3 5 v$ is insufficient to maintain a circular orbit (and certainly insufficient for a parabolic or hyperbolic orbit), so the object's orbit will become an ellipse.

Answer 2

Both the old orbit and the new orbit have to contain the point where the collision occurred. Therefore, they can't both be circles, because two circular orbits with different radii don't have any points in common.

It's possible for one or both orbits to become hyperbolic. That would be very difficult to achieve, though, because the only way for that to happen is for the satellites to collide in such a way that one or both of them somehow gain speed from the collision. One way that this could happen is if one satellite is a baseball, the other one is a rapidly spinning baseball bat, and, through an astonishing coincidence, the bat strikes the ball hard enough to knock it out of the park. Needless to say, that's not particularly likely.

Answer 3

I would be giving direct results and not proofs as they are too lengthy and mathematical.

Let us take a celestial body like the earth of mass $m$ and orbit radius is $r$. If velocity and radius vector has an angle of $\pi/2$ between them then:

$$\text{Ellipse for:}\,\,\,v\in\left(0,\,\,\sqrt{\frac{2Gm}{r}}\right)$$ $$\text{Circle for:}\,\,\,v=\left(\sqrt{\frac{Gm}{r}}\right)$$ $$\text{Parabola(open orbit) for:}\,\,\, v=\left(\sqrt{\frac{2Gm}{r}}\right)$$ $$\text{Hyperbola(open orbit) for:}\,\,\, v>\left(\sqrt{\frac{2Gm}{r}}\right)$$

If the angle between velocity and radius vector is not $\pi/2$ then also results are the same, except no circular orbit is possible in such case for any velocity.

Answer 4

bevor the collision both masses have the velocity $~\omega\,r~$. the velocity $~v'~$ after the collision is the center of mass velocity

$$v'=\frac{m_1\,v_1+m_2\,v_2}{m_1+m_2}=\omega\,r\frac{m_1-m_2}{m_1+m_2}$$

with your data $~v'=\frac 35\omega\,r$

using the Vis-Viva equation

ellipse

$$v^2=G\,M\left(\frac 2r-\frac 1a\right)\tag 1$$

Circle

$$v^2=G\,M\frac 2r\tag 2$$

Hyperbola

$$v^2=G\,M\left(\frac 2r+\frac 1a\right)\tag 3$$

with $~v=v'~$

• $v'^2\ne G\,M\frac 2r$

with $~M=1~,G=1,~\omega=1,r=1~$

• $~a > 0 ~$ equation (1)
• $~a < 0 ~$ equation (3)

thus the only possibility is ellipse

Answer 5

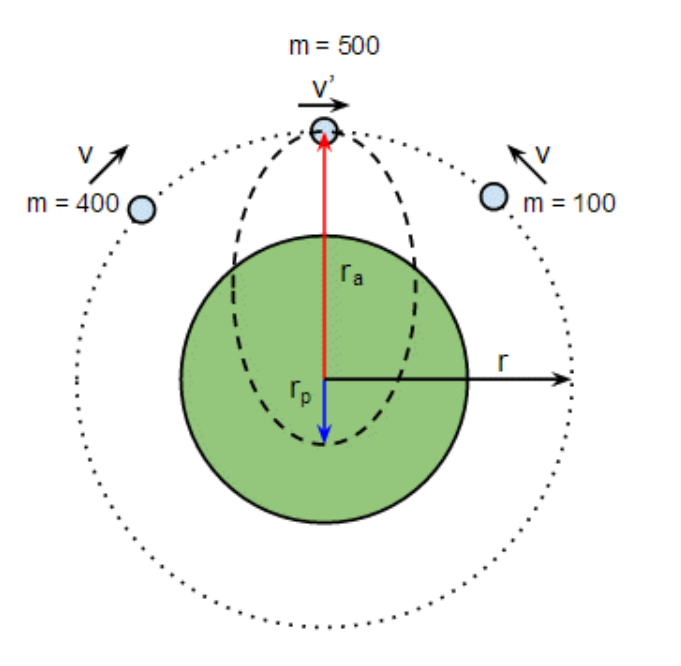
As an intuitive answer to "why can't it result in a smaller circular orbit":

The collision occurs at radius $r$. Therefore the resulting trajectory must pass through a point $r$ distance from the central body.

A hypothetical smaller circular orbit would have some radius $r'$. But a circular orbit by definition has constant radius. A body moving in that orbit is always at $r'$ distance, never at $r$. So for an instantaneous collision to produce a radius $r'$ circular orbit, the body would be required to teleport from distance $r$ to distance $r'$. That's clearly impossible.

So that means that if the body is to end up in a circular orbit at $r'$, there must be some intermediate non-circular trajectory taking the body from radius $r$ to radius $r'$, before it can take up its final circular orbit. But if the impact puts it on such a trajectory, why does it later change trajectory again (from a non-circular orbit into a circular one) when it reaches radius $r'$?

For this to happen would require another force to cause the change in trajectory; another impact, or an engine firing, etc. But that would mean the lower circular orbit at radius $r'$ isn't the result of the initial collision, but the result of both events (taking place at different times and places). If the impact is the only event we're analysing, then the "intermediate" trajectory is the final one.

Basically: an object can never transition from a circular orbit at one radius to a different circular orbit at another radius (whether lower or higher) as the result of a single event. And the reason is quite straightforward: the object is simply not located on the second circle, so it can't possibly be in a circular orbit on that circle!