0
$\begingroup$

When an electron drops to a lower orbit, energy is released as a photon. Gluons expand and contract in-between quarks, described like springs or rubber bands that have tremendous force when extended but only minor force when contracted. Where does that energy go when a gluon contracts? Is it released as some other virtual particle?

Improve this question
$\endgroup$
1
  • $\begingroup$ Like all other “elementary” particles in the Standard Model, gluons — the quanta of the gluon field — are point particles and can’t expand or contract. The gluon field forms, in some sense, a color flux tube and this flux tube can expand or contract. Since the gluon field only interacts with itself and quarks, any energy transfer has to be with those fields. $\endgroup$
    – Ghoster
    Commented Nov 6, 2022 at 21:15

1 Answer 1

Reset to default
2
$\begingroup$

In quantum mechanics, a proton is a stationary state, like the ground state of a hydrogen atom: all protons are indistinguishable at all times, forever. The only time evolution is an unobservable phase factor.

Any language that contradicts that (other than GUTs and proton decay) is metaphorical: don't take it too seriously.

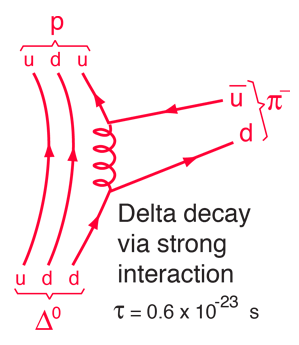
An analogy of atomic transitions is the decay of the Delta baryon:

enter image description here

While gluons are working underneath, this can be viewed in effective field theory as an energy level transition via pion emission.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I have read many times that electrons are indistinguishable but I have never heard the same for protons. Given that protons contain "a sea of quarks and gluons popping in and out of existence" I can't see how they can be indistinguishable from each other or even individually given that they are constantly changing. Can you give me more information on this aspect of your answer? $\endgroup$
    – foolishmuse
    Commented Nov 7, 2022 at 0:44
  • $\begingroup$ @foolishmuse They aren't constantly changing. That language is not precise. Atoms in BECs are certainly indistinguishable, yet the lamb shift tells us $e^+e^-$ pairs are "popping in and out of existence" from the virtual photon that binds the electron and nucleus. It's just an expression left over from old fashion perturbation theory. $\endgroup$
    – JEB
    Commented Nov 8, 2022 at 14:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.