The wave equation
$$\frac{\partial^2 u}{\partial x^2} - \frac{1}{v^2}\frac{\partial^2 u}{\partial t^2} = 0$$
is to be solved for the transverse displacement $u(x,t)$ for the fixed-driven boundary conditions of a string
\begin{align*}
u(0,t)= 0\quad \text{and}\quad u(L,t) = u_0e^{j\omega_0 t},
\end{align*}
where $u_0$ is the drive amplitude.
Employing separation of variables leads to the general solution
\begin{align}\label{general}\tag{1}
u(x,t) = (Ae^{-jkx} + Be^{jkx})(Ce^{j\omega t} + De^{-j\omega t})\,,
\end{align}
where $k = \omega/v$. Application of the fixed boundary condition $u(0,t)= 0$ necessitates $B= -A$, so equation (\ref{general}) becomes
\begin{align}\label{general2}\tag{2}
u(x,t) = (e^{-jkx} - e^{jkx})(C_1e^{j\omega t} + C_2e^{-j\omega t})
\end{align}
where $A$ has now been factored into the constants $C$ and $D$ in equation (\ref{general}), giving two new constants $C_1$ and $C_2$ in equation (\ref{general2}). Meanwhile, application of the driven boundary condition $u(L,t) = u_0e^{j\omega_0 t}$ to equation (\ref{general2}) gives
\begin{align}
u(L,t) = u_0e^{j\omega_0t}&= (e^{-jkL} - e^{jkL})(C_1e^{j\omega t} + C_2e^{-j\omega t})\notag\\
&=\frac{j}{2}\sin(kL)(C_1e^{j\omega t} + C_2e^{-j\omega t})\tag{3}\label{particulars}
\end{align}
To satisfy equation (\ref{particulars}), the phase on both sides of the equation must match, implying that $C_2 = 0$ and $\omega = \omega_0$ (and thus $k = k_0 =\omega_0/v$):
\begin{align*}
u_0e^{j\omega_0t} =\frac{j}{2}\sin(k_0L)C_1e^{j\omega_0 t} \quad \implies \quad C_1 =\frac{2u_0}{j\sin(k_0L)}\,,
\end{align*}
so the general solution, equation (\ref{general2}), becomes $u(x,t) = \frac{2u_0}{j\sin(k_0L)}(e^{-jk_0x}-e^{jk_0x})e^{j\omega_0 t}$, or
\begin{equation}\bbox[5px,border:2px solid purple]
{u(x,t) = u_0 \frac{\sin(k_0x)}{\sin(k_0L)}e^{j\omega_0 t} }\label{solutioners}\tag{4}
\end{equation}
Note that equation (\ref{solutioners}) matches the form of the solution to the 1D acoustic wave equation for a source at one end of a tube that is closed at the other end.
To visualize this solution to the wave equation, equation (\ref{solutioners}) is rewritten in terms of three dimensionless parameters, the reciprocal of the number of wavelengths in the string $\lambda_0/L$, distance normalized by the length of the string $x/L$, and dimensionless time $\omega_0t$:
\begin{align}\label{rewrite it}\tag{5}
\frac{u(x,t)}{u_0} = \frac{\sin[(x/L)(2\pi L/\lambda_0)]}{\sin(2\pi L/\lambda_0)}e^{j\omega_0 t}\,.
\end{align}
Below are animations of equation (\ref{rewrite it}) for various values of $\lambda_0/L$. Note that the string has an infinite response for $\lambda_0/L = 1/n$, where $n = 1,2,\dots$, because at these frequencies correspond to the eigenfrequencies of the fixed-fixed string.
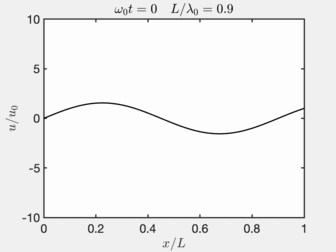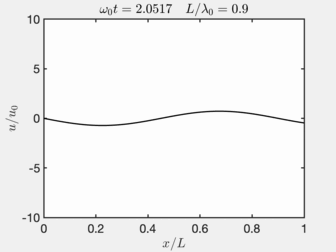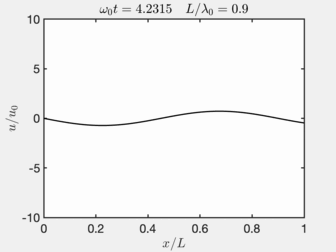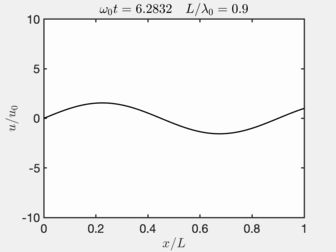

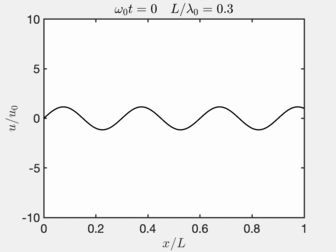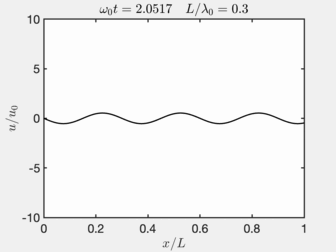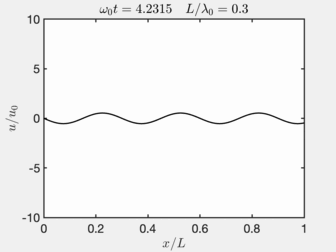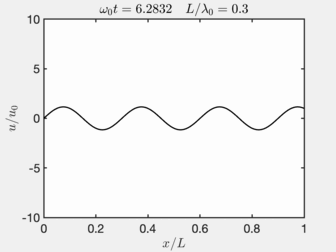
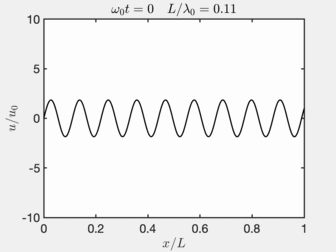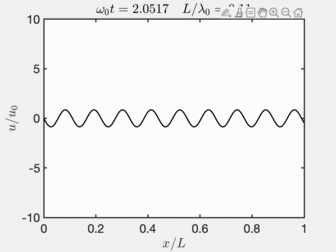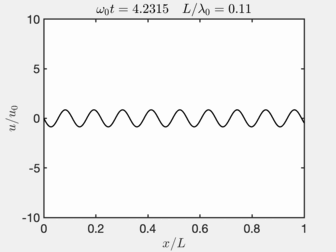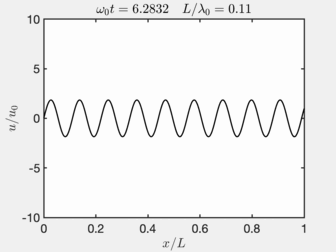
Finally, note that the d'Alembert solution solves the initial-value problem and is not of use to the boundary-value problem at hand.




