2
$\begingroup$

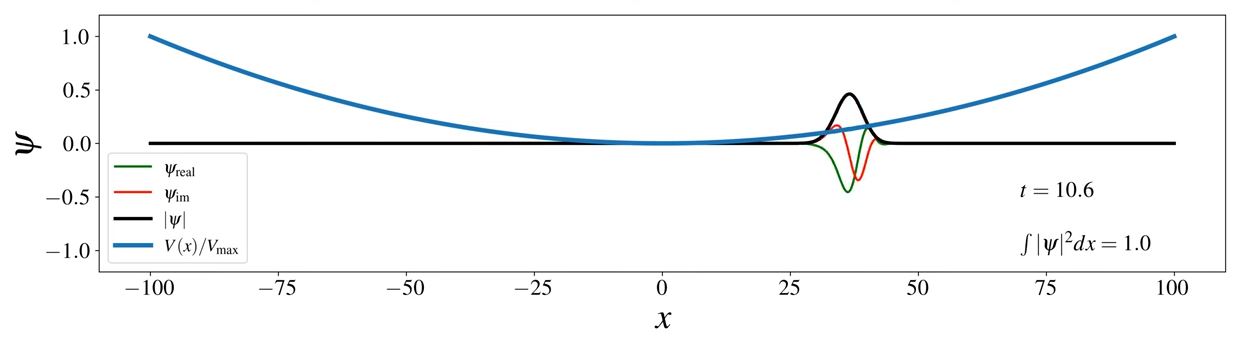
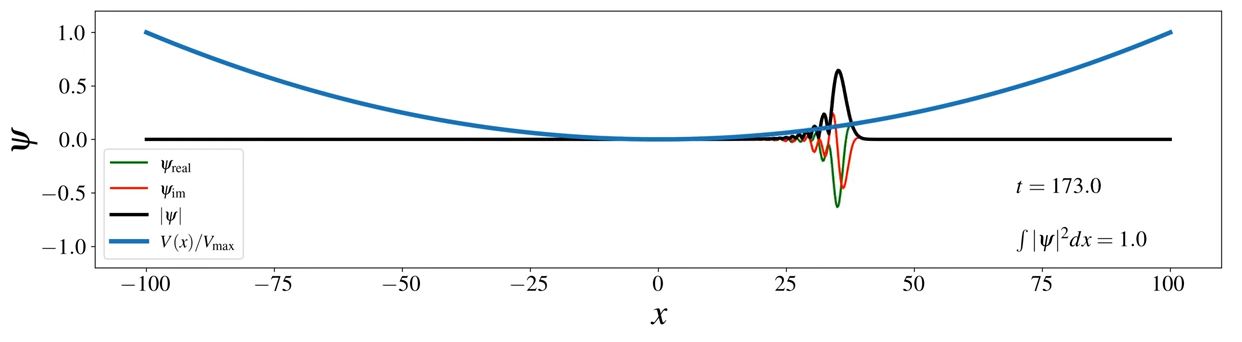
I have come across this video on Youtube where someone simulated the wave function of a moving particle in an unspecified harmonic potential well. (Link: https://www.youtube.com/watch?v=hHAxLE181sk , by PhysicsProgrammers)

What I noticed is how at first, the wave packet gets thinner when being reflected. But as time goes on, interference patterns can be seen forming when the particle changes direction. The wave function also gets wider at $x = 0$. These effects appear to get stronger with time.

Screenshots of the wave being reflected at $t=10.6$ and $t=173.0$:

First reflection of the wave Tenth reflection of the wave

The video is only 2 minutes long, but I tried to imagine how this simulation would develop further.

From my intuition, if time approaches infinity, I expect the wave to end up in a form that resembles a stationary solution for the quantum mechanical harmonic oscillator with the energy $$E_n = (n+1/2)\hbar\omega.$$ The idea is that, as time goes on, the particle wave will widen thanks to the initial momentum and position uncertainty. It will widen until it might become impossible to see any movement (the wave reaches maximal 'flatness'). It will be impossible to know where the particle is (like losing track of a moving ball in a box after a large amount of time), but the Energy should stay the same. The interference patterns will get stronger and stronger until they resemble the picture of a stationary particle with energy $E_n$. I don't see how this effect would not also be true with any other potential well.

Unfortunately, I have no way to test this idea. I do not even know for certain if the interference patterns are an artefact of the simulation, but I have seen simulations of particles being reflected at infinite potential walls and forming similar patterns.

Does my idea come close to how it would play out, or does the wave develop entirely differently? Will it become chaotic? Apologies if my question is not specific enough. It just came to mind after watching the video, and my knowledge of Quantum Mechanics is very basic at best.

Improve this question
$\endgroup$
1
  • $\begingroup$ The problem with numerical simulations of the SE is that they tend to give us a more or less random superposition because the initial conditions are usually unphysical (like perfect localization of the particle in one position) or random choices like a Gaussian wave packet in a potential that does not have Gaussian eigenfunctions. Since the mathematical background is identical to that of the Fourier transformation, we are also bound to run into effects similar to the Gibbs phenomenon. While they are real, they don't tell us much about the actual physical system. $\endgroup$
    – FlatterMann
    Commented Oct 21, 2022 at 18:42

1 Answer 1

Reset to default
4
$\begingroup$

The answer to the headline question is "no." Suppose we start with a state $|\psi(0)\rangle$ and evolve it under the time-evolution operator: $$ |\psi(t) \rangle = e^{-i H t/\hbar} |\psi(0) \rangle $$ Then the probability $P_n(t)$ of measuring the system to be in an energy eigenstate $|\phi_n\rangle$ with energy $E_n$ is constant with respect to time, since \begin{align*} P_n(t) &= |\langle \phi_n| \psi(t)\rangle|^2 \\ &= |\langle \phi_n| e^{-i H t/\hbar}|\psi(0)\rangle|^2 \\ &= \left| (e^{i E_n t} \langle \phi_n|) |\psi(0)\rangle \right|^2 \\ &= |e^{i E_n t}|^2 |\langle \phi_n| \psi(0)\rangle|^2 \\ &= |\langle \phi_n| \psi(0)\rangle|^2 = P_n(0). \end{align*} So a "non-stationary state", which is a state that is a superposition of energy eigenstates, can never evolve to a "stationary state" (a single energy eigenstate), because if there is a non-zero amplitude of more than one energy eigenstate initially then this will continue to be the case for all time.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.