Understanding coordinate system for looping pendulum equations of motion

Question

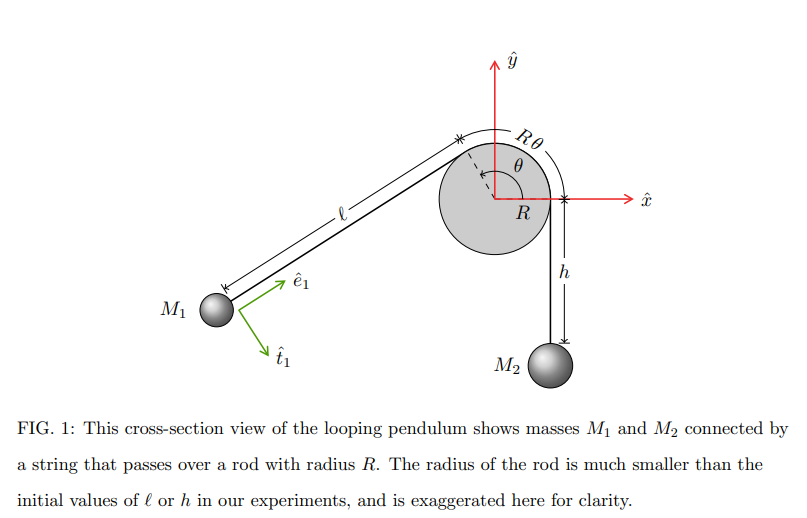
I'm interested in the phenomenon of the looping pendulum, which is a system consisting of one large and one small mass connected by a string passing over a rod. In particular, I've been looking at this paper: https://arxiv.org/abs/2103.14752

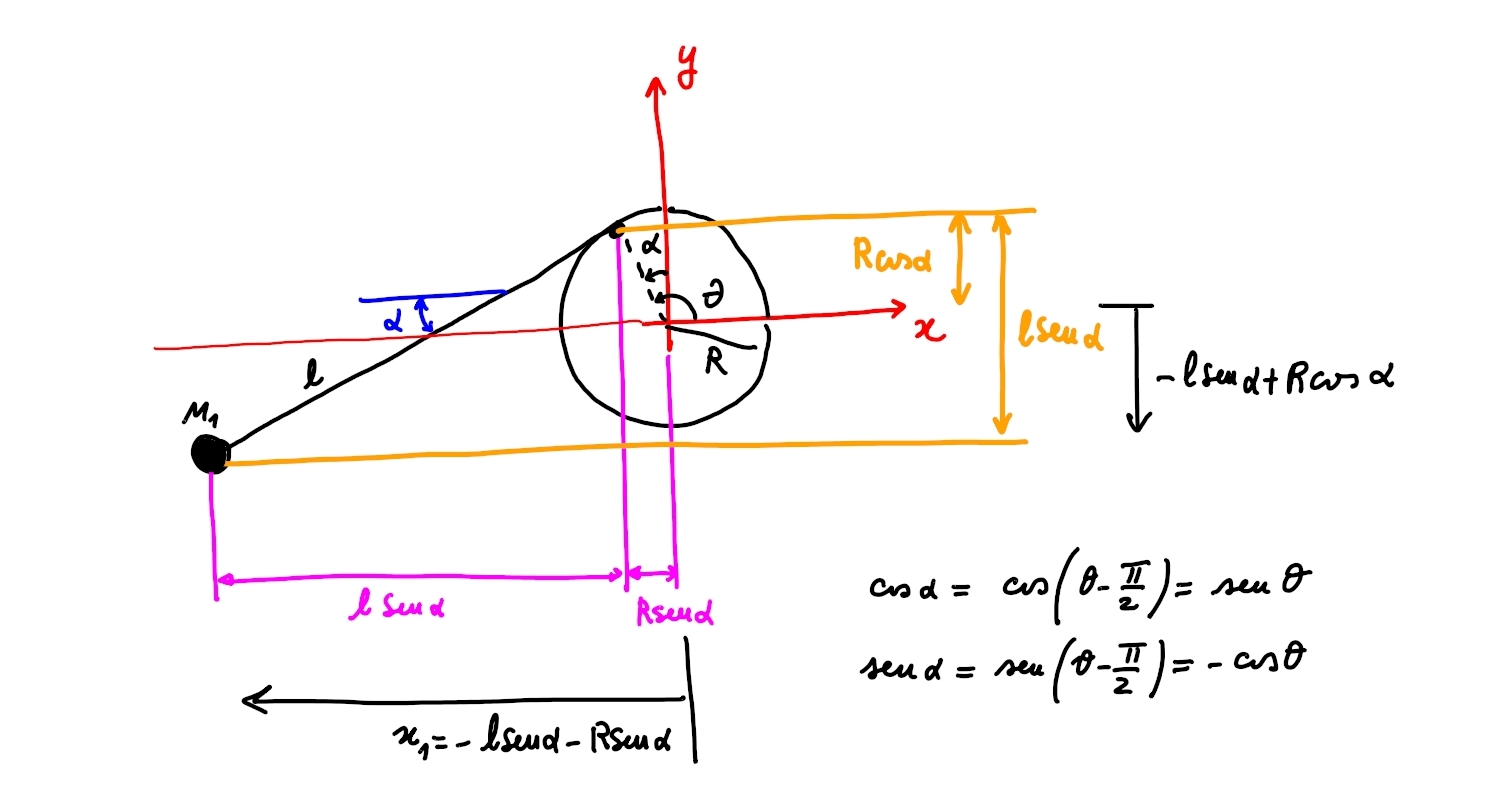
What I've been struggling to understand is how the position vector of the mass $M_1$ was expressed as

$$\vec{r}_1=(R \cos \theta-\ell \sin \theta) \hat{x}+(R \sin \theta+\ell \cos \theta) \hat{y}$$

on page 3 of the paper. I would greatly appreciate if someone could go through the process by which the position was expressed like this.

Answer 1

You only need to project the dashed radius and the plain line of length $\ell$ on horizontal (for $x$-component) and vertical directions (for $y$-component),

Let's call $\alpha = \theta - \dfrac{\pi}{2}$ the angle between the dashed radius and the vertical axis, that is the same angle as the angle between the wire of length $\ell$ and the horizontal axis.

Thus $x$- and $y$- coordinates of the mass $M_1$ reads

$x_1 = - \ell \cos \alpha - R \sin \alpha = -\ell \sin \theta + R \cos \theta$
$y_1 = -\ell \sin \alpha + R \cos \alpha = \ell \cos \theta + R \sin \theta$