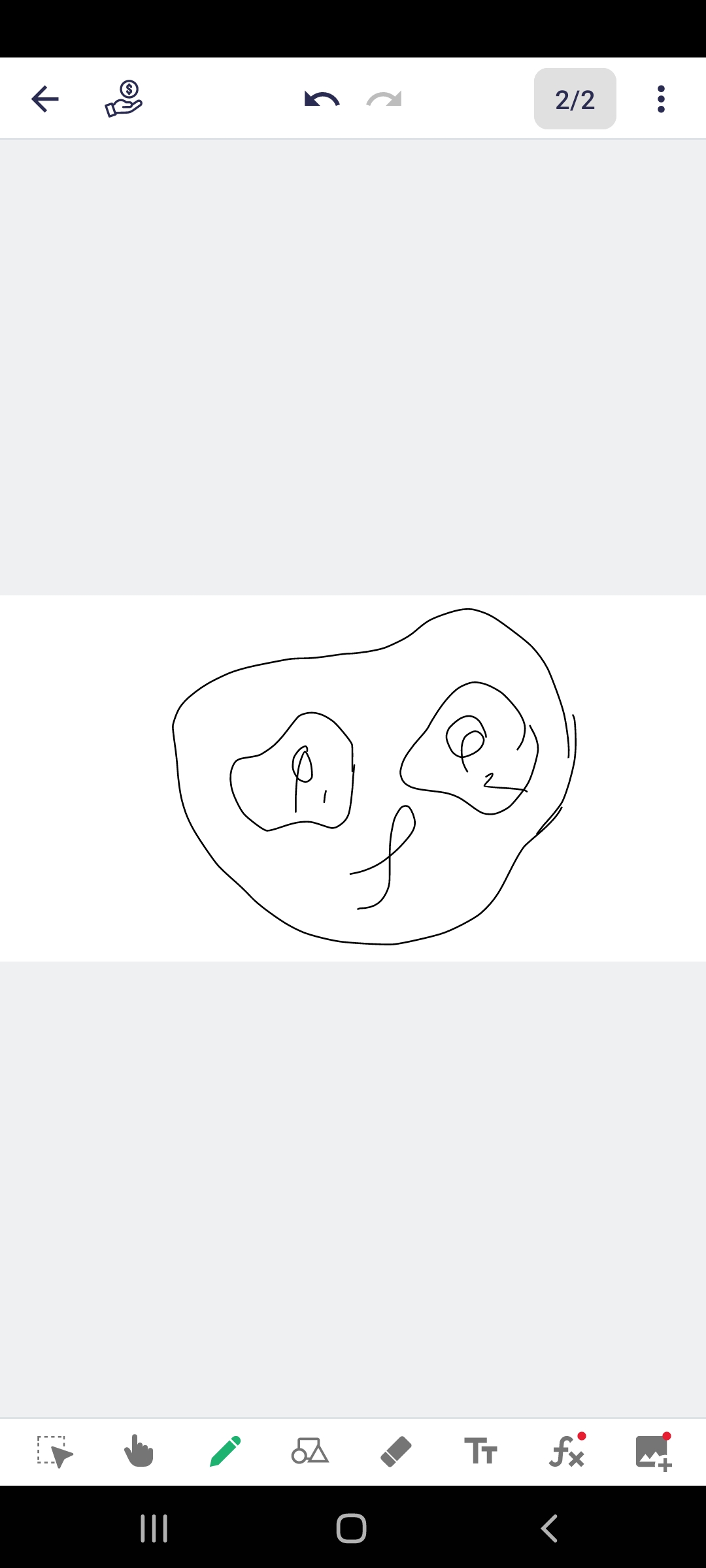
What I don't understand is that we have a big Region with volume $V$ and there are two cavities inside the volume $V$ ,the cavities ,each of them hold $Q_1$ & $Q_2$ charges in them.The density of the charges in between the regions of the conductor is ρ .
Now Second Uniqueness Theorem postulates that the Electric Field of this setup is unique .
My question is if $E_1$ & $E_2$ are two Electric Fields, then due to Gauss Law. $$\nabla.E_1=\dfrac{ρ}{ε_0}$$.
Similarly $$\nabla.E_2=\dfrac{ρ}{ε_0}.$$
This equation states that Electric field due to the charge density at any point in between the cavities are $E_1$ & $E_2$.
Integral form states that the Electric Field due to charges on the surface on Cavities (ie) $Q_a$ & $Q_b$ are $$\oint\vec{E_1}.\vec{dA}=\dfrac{Q_a}{ε_0}$$ etc (The same for $E_2$).
Now
Is the electric fields $E_1$ & $E_2$ ,is it because of the charges on the surface of the cavities or is it because of the charge density in between the cavities?
Are the charges $Q_a$ & $Q_b$ taken into account when estimating the charge density $ρ$?