Are we measuring static, dynamic, or total pressure?

Question

Our lab is using a gas driven shock tube and are using piezoelectric sensors to measure the pressure that the shock wave produces. There are three sensors placed near the end of the shock tube, perpendicular to the direction of gas flow, and one sensor placed a few centimeters away from the exit of the tube facing the shock tube and parallel to the direction of flow. There is a short millisecond scale shock wave as the pressure spikes and rapidly decays.

We are trying to figure out if the sensors are measuring static, dynamic, or total pressure. We are a neuroscience lab and not an engineering lab so don't have the expertise to come to a solid conclusion.

The link to the sensors we are using: https://www.pcb.com/products?m=102a05 Thank you in advance for any help!

Answer 1

Preliminaries

Bernoulli theorem for compressible isentropic flows. Assuming that Bernoulli theorem holds in a region (steady state, no rotational effect, isentropic flow - i.e. negligible viscosity), the total enthalpy is uniform, $h^t = e^t + \frac{P}{\rho} = e + \frac{P}{\rho} + \frac{1}{2} V^2$, i.e. considering two points of this regions,

$h^t_1 = h^t_2 \qquad \rightarrow \qquad e_1 + \dfrac{P_1}{\rho_1} + \dfrac{1}{2} V_1^2 = e_2 + \dfrac{P_2}{\rho_2} + \dfrac{1}{2} V_2^2 $.

Bernoulli theorem for compressible non-isentropic flows and jump conditions. Shocks introduce entropy in the flow, so you can use Bernoulli theorem on both sides of the shock independently, but you can't directly connect two points across a shock using Bernoulli equation above. When you cross a shock, thermodynamic variables on its sides are related by the jump conditions, see as an example https://en.wikipedia.org/wiki/Normal_shock_tables.

Static, dynamic and total pressure in isentropic flows. Static, dynamic and total pressure are definitions of different terms of energy balance equation (in the form of the Bernoulli theorem), usually introduced by experimental guys:

• static pressure is thermodynamic pressure itself;

• dynamic pressure $q$ is defined as $q = \frac{1}{2}\rho V^2$;

• total pressure $P_0$ is defined as the pressure you get if you isentropically (and thus you can use Bernoulli theorem) decelerate the flow until it is at rest, i.e. $V_0 = 0$,
  $e_1 + \dfrac{P_1}{\rho_1} + \dfrac{1}{2} V_1^2 = e_0 + \dfrac{P_0}{\rho_0}$ and it is a measure of the total enthalpy of the flow.

  Using ideal gas law $P = \rho R T$, the expression for the internal energy $e = c_v T$, and isentropic relation $P/\rho^\gamma = const.$ we can further manipulate this expression, to get the total pressure as a function of the pressure (what the experimental guys call static pressure) and the local Mach number, $M$, of the flow,

  $P_0 = P \left(1 + \dfrac{\gamma-1}{2} M^2 \right)^{\frac{\gamma}{\gamma-1}}$.

Experiment

What do the sensors measure? The piezoelectric sensors you're using always give you a measurement of the pressure acting on the sensitive surface.

Interpretation of the measures using the "experimental language". Now, let's give an interpretation of the measurements you're taking.

• A sensor whose surface normal is perpendicular to the direction of gas flow, approximately is not intrusive at all, it doesn't stop the flow and, assuming a uniform flow inside the tube, it measures the pressure (static pressure) in that section of the tube;
• A sensor whose surface normal is parallel to the direction of the flow is locally very intrusive, since it locally stops the flow and, thus it measures the total pressure, $P_0$ in the flow in the region where it is placed.
  *(You need to pay attention that if this sensor produces a shock before it in the flow, you need to use the jump conditions, to correct for the intrusivity of the sensor in the flow: the sensor generates a shock that would not be there if the sensor is removed)

Answer 2

I'm not an expert, because I'm not working in a technical branch.

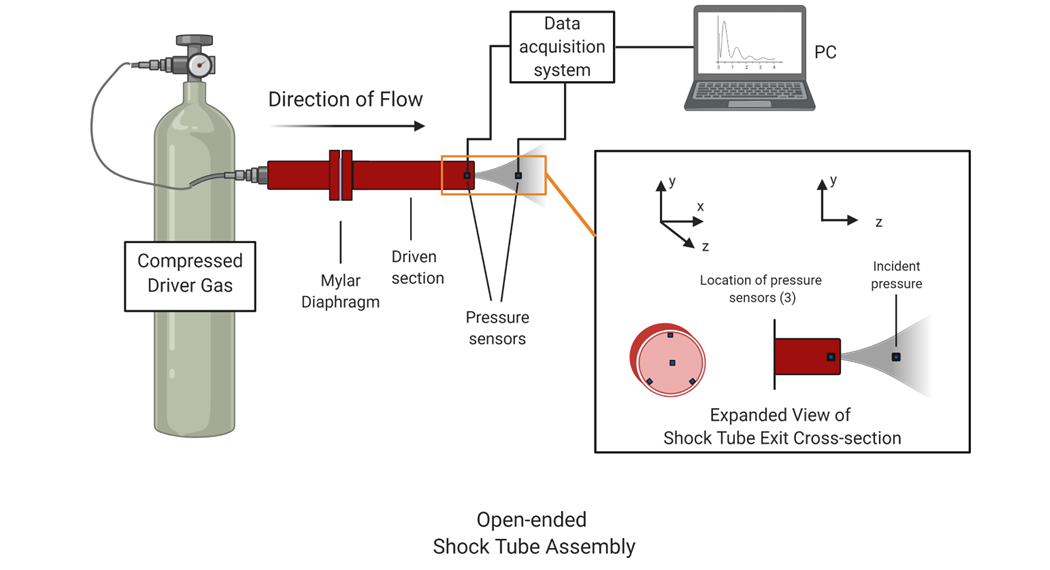
But I have a certain idea about the Piezoelectric effect in crystals. I think the sensors are measuring the variations of the static pressure. Because the sensors are sensible at mechanic compression, as far I know. Indirectly, these are also measuring the variations of dynamic pressure. At an increasing of the dynamic pressure, the static pressure decreases. In reality, I think the total pressure also has a variation. This is because the gas it is compressible, it is not an ideal gas. And the total pressure it is not conserved.

Dynamic pressure is expressd in International Units [ N/m^2 (Pa) ] as:

Pd = f * v^2 / 2 

Where f is the density of the gas, v is the velocity of the molecules.

Static pressure Ps, is the value of a distributted force per area unit:

Ps = F / S = n * R * T / (S * v * t) 

where (S * v * t) is the volume, or debit.

Speed multiplied by time gives a displacement vector for the flowing.

Can be also expressed in function of Temperature and molecular density n. R is the constant of Rydberg.

Between the total pressure Pt, the static pressure and dynamic pressure, could be a relation of equality:

Pt = Pd + Ps 

Apparently, this equality is not satisfied for a limited region of space. Or for a smaller amount of time. But if we make an average for all space, and for a longer time, the average pressure shoud verify this equality. Can be the case of a storm of gas, where it is a dispersion of the speeds. Or any turbulence, can break this balance for a limited period of time.

For describing better this behavior, the Brnoully equation can be used. According to this equation, the next formula could be applied:

v^2 / 2 + g * z + Ps / f = K

where K is a constant g, gravitational acceleration and z, the altitude. As it has been explained before, these could be also average values.

But this formula it can be valid only for liquid fluids. For gases, it is another new term for variation of a flowing potential:

dF / dt + v^2 / 2 + g * z + Ps / f = K 

These callculations require an integration of this equations. You can find more details in Wikipedia about Bernoulli equation:

https://en.wikipedia.org/wiki/Bernoulli%27s_principle

Hope it helps, Adrian.

Answer 3

The behaviour of pressure waves is described by the wave equation, which is based on the perturbation Ansatz, so that one assumes:

$p=p_{static}+p_{dynamic}$

Which part is measured depends on the sensor used. Classical acoustic measurement microphones are built in such a way that they only measure the dynamic component through static pressure compensation (basically theres a hole in the microphone behind the membrane). If your sensors measure the total pressure, the static pressure component is a DC offset in the signal, so you can simply separate those. If there is no DC offset you are measuring the dynamic pressure.