What is the meaning of "local" band structure?

Question

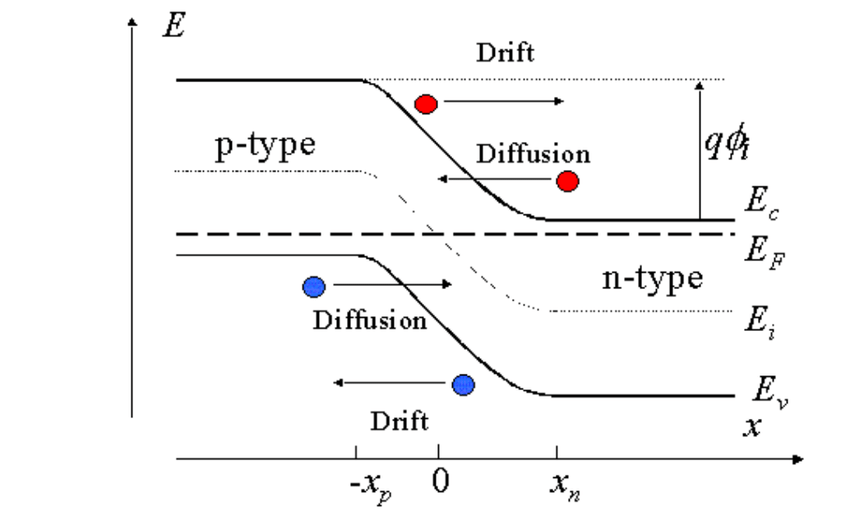
In solid state physics, especially when discussing physics of junctions and interfaces, very often diagrams of band edges with respect to position are drawn, such as the following pn junction diagram.

Since band structure is defined using an infinite lattice, how can it depend on position (in principle - I don't like answers like "the atom does not see the potential landscape "far away", because in band structure model, the carriers are completely delocalised)? In particular, what Hamiltonian involving $x$ as a parameter would produce such an energy spectrum?

I have been thinking about this a lot recently and came to the conclusion that the band structure can be localized by supposing it is a property of the unit cell. All information about it is contained in the unit cell and periodic boundary conditions. However, the unit cells may or may not be deformed into each other continuously as a function of $x$, in which case the diagram cannot be drawn.

I also do not understand how can it be guaranteed that

1. a particle can be effectively localised by taking many energy levels to produce a Wannier orbital, because that would (in principle) require to solve the Schrodinger equation for both sides of the interface as a single quantum system, removing the periodicity and therefore band model is no longer appropriate,
2. a particle localised in a certain region (let's say, far to the left in diagram 1) in an energy eigenstate at the bottom of the conduction band with $k_x > 0$, as it "travels" to the left, follows the conduction band edge "adiabatically".

Answer 1

These diagrams are to be understood as semiclassical approximations in which both positiona and momentum are rereasonably well defined and one can use Hamilton's equations with $$ H(p,x)\to E(p,x) $$ where $E(p,x)$ is the energy as a function of $p$ in the neghbourhood of point $x$. The semiclassical approximation is valid if the band structure changes slowly on the scale of the unit cell

The idea is basically the same as deriving geometric (ray) optics from the wave equation. Alternatively you can think of it as a WKB-like approximation which is valid when the Fermi wavelength is much smaller than size of the junction.