When I was studying solid state physics, I found that the Cv remains constant at high temperatures for solid material but it changes at low temperatures.
My question is what happens in the solid material which causes its specific heat to be constant at high temperatures regardless of temperature change.
How the energy is distributed among the molecules, atoms or electrons.
And why not the law of equipartition of energy not applicable at low temperatures?
As you are asking for the high temperature limit I hope that you will agree that classical mechanics is enough. In this framework, you can approximate a solid to a bunch of ions connected through spring-like bonds (Boltzmann's solid), and you can use the equipartition theorem or your favorite statistical ensemble to show that the heat capacity is a constant. At high temperature the nature of the bonds or the geometry does not matter much as long as it is crystal like and the energy spectrum is continuous.
At low temperatures, you need a quantum picture, if not the calculation fails. If you extrapolate the ion-spring model to its quantum version (Einstein's solid) you will indeed obtain a heat capacity that depends on temperature. See figure:
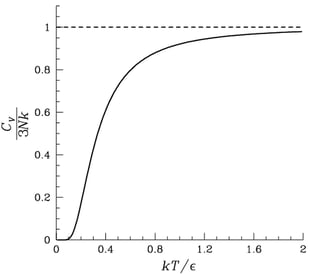
Heat capacity $C_V$ as function of the temperature $T$
The main difference with the high temperature case, it is that for low temperatures, the energy is quantized and you only activate a discrete number of these modes which gets lower and lower with temperature.
As you get closer to zero kelvins you have to use more sophisticated models that take into account the geometry of the lattice (phonons) and you start to need to add the electronic contribution as it starts to become relevant (at least in some solids like metals).
There are different always to approach looking at the heat capacity of solids and one challenge early last century was to match the experimental observations with theory.
The link has a nice write up with equations, but the short answer is that lower temperature you are strongly dependent on the density of states. You can think of this a there being an increasing number of modes being filled as the temperature is rising.
For each material there is a characteristic temperature, the Einstein temperature, or Debye temperature. At high temperature the ratio of that characteristic temperature with the temperature of the material goes to 0. Essentially the system is saturating as all the available modes are used and there can only be a finite number of modes.
You can also consider the contribution due to electrons, but that is typically much smaller than lattice vibrations at room temperature. At low temperatures it is more important. In that each electron will add a small amount to the heat capacity proportional to temperature.
