0
$\begingroup$

Can two Cartesian inertial frames, in general relative motion, be drawn parallel to each other? A picture in Wikipedia suggests this, but some answers on this site appear to differ.

Consider observers 1 and 2 are in two inertial frames $\Sigma_1$ and $\Sigma_2$ with relative velocity of 2 wrt 1 as $V=(v_x,v_y,v_z)$, $v_x v_y v_z≠0$, sharing origin, $x$-axis, and $xy$-plane at time $0$. Would the $y$-axis of $\Sigma_2$ be perpendicular to $x$-axis of $\Sigma_1$ as reported by observer 1?

In Wikipedia's picture:

Wikipedia's picture

the coordinates are drawn as if various axes of 1 and 2 form a perfect box, as in Galilean relativity. A comment on the picture's caption

comment below picture

seems to challenge that statement. I tend to think that observer 1's will report the coordinate frames of 2 and 1 won't form a perfect box, various angles won't be 90 degrees. References to texts with a clarification of the issue is appreciated.

Improve this question
$\endgroup$
3
  • $\begingroup$ Any linearly independent set of coordinate axes $(x,y,z)$ and $(x',y',z')$ could serve as coordinate systems for both, $F$ and $F'$. Nothing forbids us to choose both Cartesian (or as you call them perfect box(es)) to make things as simple as possible. If we did not there is just some gratuitous extra work when comparing what $F$ observes vs. $F'$. That's all. It seems like you are mixing this up with Minkowski diagrams, where typically the $t'$ axis is not at 90 degrees with the $x'$ axis to make visualization of time dilation and length contraction easier. $\endgroup$
    – Kurt G.
    Commented May 12, 2022 at 14:07
  • 1
    $\begingroup$ Related : Parallel axes between inertial frames in Special Relativity. $\endgroup$
    – Voulkos
    Commented May 12, 2022 at 14:16
  • $\begingroup$ The paper referred to by Fobenius can be found here fr.art1lib.org/book/11401699/65a583 $\endgroup$
    – Maesumi
    Commented May 12, 2022 at 15:37

1 Answer 1

Reset to default
2
$\begingroup$

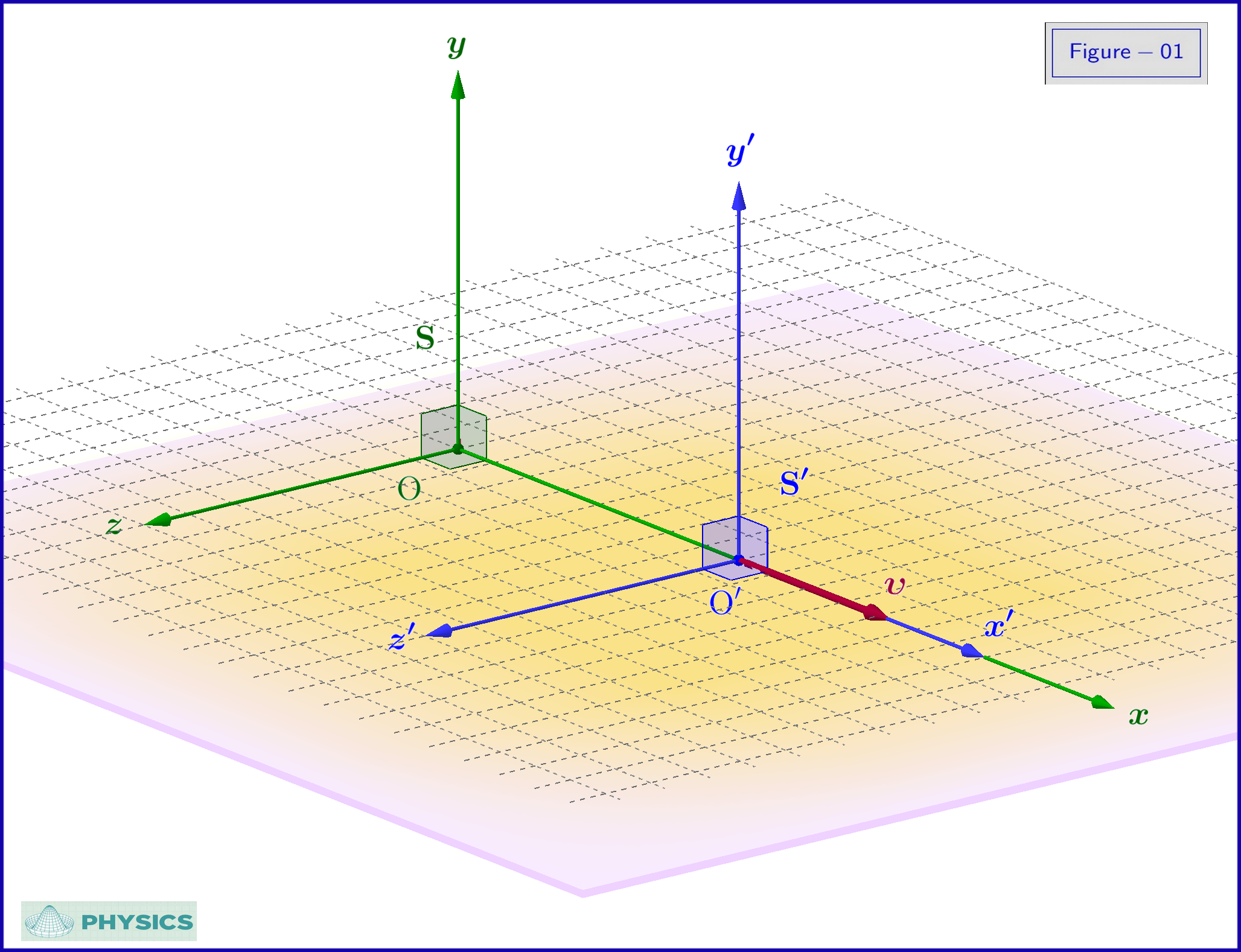
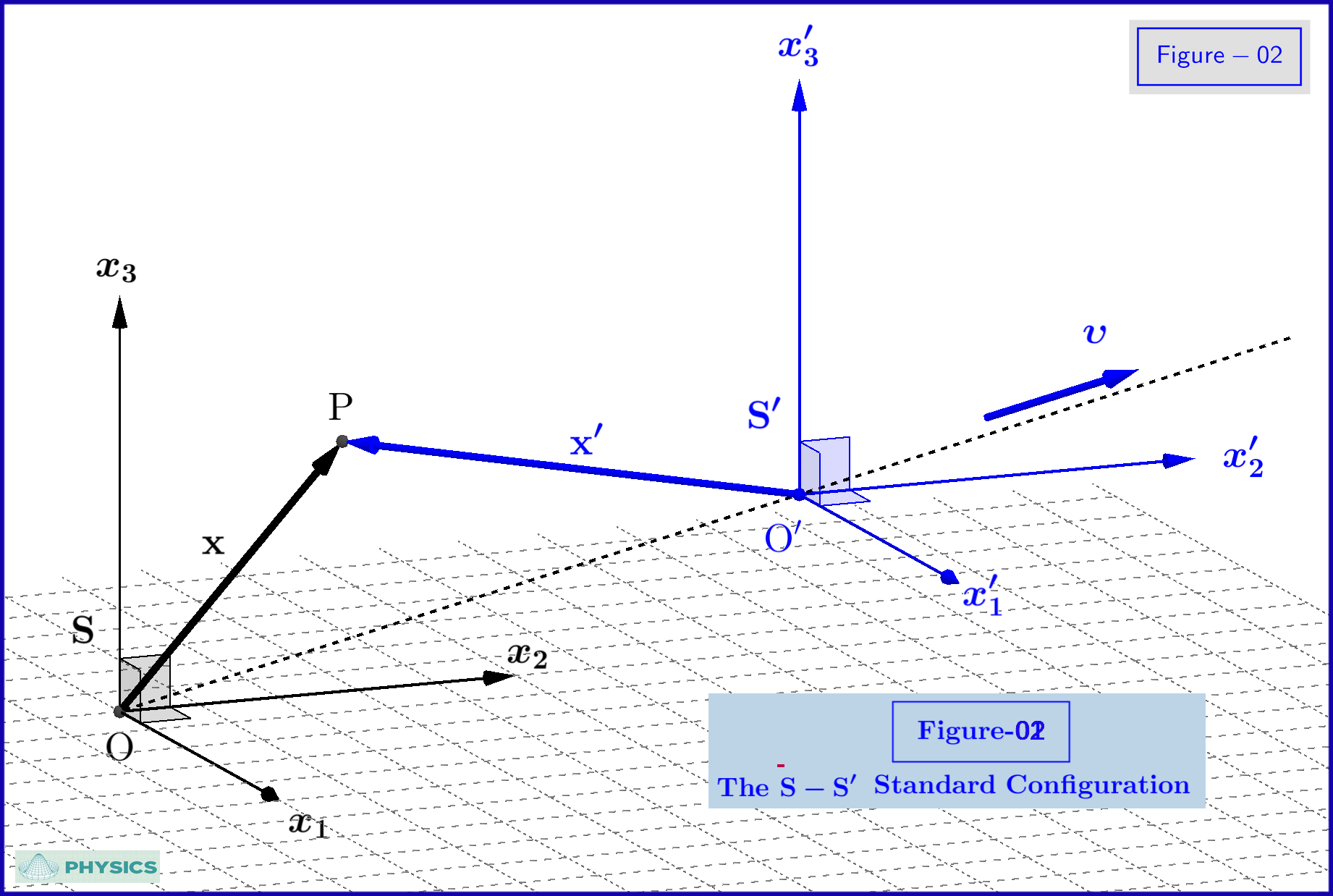
In Special Relativity we couldn't say in general that the axes of two inertial frames $\:\rm S\:$ and $\:\rm S'\:$ in relative translational motion (boost) are parallel, see Figure-02, except of special cases, see Figure-01.

Suppose that a frame $\:\rm S'\:$ is moving uniformly with velocity $\:\boldsymbol\upsilon\:$ with respect to an inertial frame $\:\rm S\:$ and with common $\: x',x-$axes along this velocity as in Figure-01. Planes $\:\mathrm O'x'y'\:$ and $\:\mathrm Oxy\:$ are parallel. We could make the $\: y',y-$axes parallel and the same for the $\:z',z-$axes. This is a special case we could talk about parallel axes between the two inertial frames.

To the contrary, in case of a general boost with velocity $\:\boldsymbol\upsilon$, see Figure-02, we could not talk about parallel axes. For example, the points of the $\:x'_2-$axis in frame $\:\rm S'\:$ at a given moment $\:t'\:$ are simultaneous events in Minkowski space, so the $\:x'_2-$axis is a well-defined straight line in $\:\rm S'$. But these events are not simultaneous in $\:\rm S\:$ so there doesn't exist such a thing or curve or whatever else in $\:\rm S\:$ to be parallel to the $\:x_2-$axis.

enter image description here

enter image description here

ADDENDUM (20-08-2022)

The $\:Ox_1\:$ axis of frame $\:\mathbf S\:$ in Figure-02 is a straight line in frame $\:\mathbf S'\:$ but not parallel to $\:O'x'_1\:$. To derive the details, extract from my answer here Is it a typo in David Tong's derivation of spin-orbit interaction? equations (03) for the Lorentz boost of Figure-02.

Consider that the points of a straight segment (vector) $\:\Delta\mathbf x\:$ are seen simultaneously by observer $\:\mathbf S\:$, that is $\:\Delta t=0\:$, to derive that in $\:\mathbf S'$ \begin{align} \Delta\mathbf{x'} & = \Delta\mathbf{x}\boldsymbol + \dfrac{\gamma^2}{c^2 \left(\gamma\boldsymbol +1\right)}\left(\boldsymbol{\upsilon}\boldsymbol{\cdot} \Delta\mathbf{x}\right)\boldsymbol{\upsilon} \tag{03a}\label{03a}\\ \Delta t' & = \boldsymbol-\,\gamma\dfrac{\boldsymbol{\upsilon}\boldsymbol{\cdot} \Delta\mathbf{x}}{c^2} \tag{03b}\label{03b} \end{align}

Depending on the sign of $\:\Delta t'\:$ return backward or forward in time $\:t'\:$ the points of $\:\Delta\mathbf{x'}\:$ in order to be seen simultaneously by observer $\:\mathbf S'$. The result will be a straight segment (vector) but not parallel to $\:\Delta\mathbf x$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Maybe I have misunderstood your answer: Is it correct that a coordinate axis/ a straight line is not necessarely viewed as a straight line from another observer or is this only about the angles between straight lines? $\endgroup$
    – Filippo
    Commented Aug 19, 2022 at 20:38
  • $\begingroup$ @Filippo : The $\:Ox_1\:$ axis of frame $\:\mathbf S\:$ in Figure-02 is a straight line in frame $\:\mathbf S'\:$ but not parallel to $\:O'x'_1\:$. To derive the details, extract from my answer here Is it a typo in David Tong's derivation of spin-orbit interaction? equations (03) for the Lorentz boost of Figure-02. $\endgroup$
    – Voulkos
    Commented Aug 19, 2022 at 21:42
  • $\begingroup$ @Filippo : See the ADDENDUM in my answer. $\endgroup$
    – Voulkos
    Commented Aug 19, 2022 at 22:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.