2
$\begingroup$

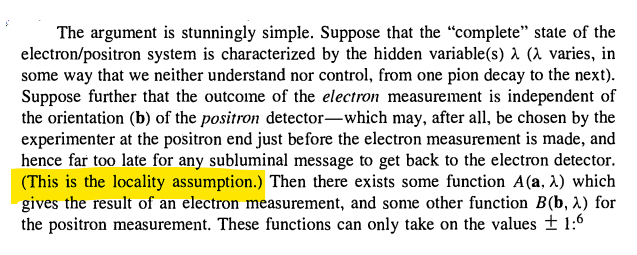
A pion decays to a singlet electron/positron state. We will measure the component of the electron's spin in the $\vec a$ direction and positron's spin in the $\vec b$ direction. If there exists a local hidden variable(s) $\lambda$, then outcomes of the measurements must be given by functions $A(\vec a,\lambda)$ and $B(\vec b,\lambda)$ respectively. these functions can only output the values $\pm1$ (in units of $\hbar/2$.) Due to entanglement and the case of $\vec a=\vec b$, we may write $B(\vec b,\lambda)=-A(\vec b,\lambda)$. We also assume a non-negative probability density $\rho(\lambda)$ which integrates to unity over all of space. Then Bell's inequality follows in the usual way.

This is my question: What is it in the above definitions for $\lambda$ that makes it "local"?

Griffiths writes the following:

enter image description here

Is the locality condition only that $A$ is strictly not a function of $\vec b$ (and $B$ not of $\vec a$), which could not be ruled out for FTL signals?

Improve this question
$\endgroup$
1
  • $\begingroup$ A great resource for understanding Bell's theorem and the notion of locality it assumes is Travis Noreen's articles on the subject (you can search for them on arxiv); this one in particular is relevant: arxiv.org/abs/0707.0401 $\endgroup$
    – Matt Dickau
    Commented Jul 1, 2022 at 13:54

2 Answers 2

Reset to default
2
$\begingroup$

In addition to $A$ strictly not being a function of $b$ (and vice versa), the locality condition is ensures that $\vec{a}$, $\vec{b}$, and $\lambda$ are completely independent of each other. In particular, $\vec{a}$, $\vec{b}$ are not functions of $\lambda$ (or correlated with $\lambda$ in any way). Any such correlation would spoil the proof of Bell's theorem.

Improve this answer
$\endgroup$
0
0
$\begingroup$

What makes the functions $A(a,\lambda)$ and $B(b,\lambda)$ local are two factors

  1. As you said before the fact that $A(a,\lambda)$ does not depend on b.
  2. The $\lambda$ variables are fixed at the source at the moment the particles are created. So their behavior is "locally" explained or predetermined by these variables.

Notice that "predetermined" in a general sense does not imply determinism because the model may also be stochastic.

Improve this answer
$\endgroup$
2
  • $\begingroup$ For a given direction in $A(a,\lambda)$ we must put $a=\theta$ and for the opposite direction $a=\theta+\pi$. So the function is well defined. $\endgroup$
    – facenian
    Commented Jul 20, 2022 at 20:05
  • $\begingroup$ Suppose we consider variable 1 in a and 2 in b, if we look at thd setup in a spacetime diagram, then after the meadurement 1 can "know" the direction a. So looking at it through time the lambda1 can depend on a after ghe measurement and be transmitted to the averager, like in $cov(a,b,l1,l2)=1/(l1*l2)\int_0^{l1}Cos(A-S)ds\int_0^{l2}cos(b-t)dt$ $\endgroup$
    – QuantumPotatoïd
    Commented Aug 7, 2023 at 10:36

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.