Can there be thermal equilibrium of a gas at non-uniform distribution of its particles?

Question

Sometimes thermal equilibrium of a thermodynamic system is defined as a state when the system’s temperature is uniform and constant at a given moment of time.

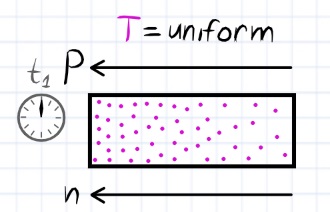
Now, look at the picture below and consider the distribution of a gas’s particles for moment $t_1$.

where $T$, $P$ and $n$ is temperature, pressure, and concentration of the gas, respectively.

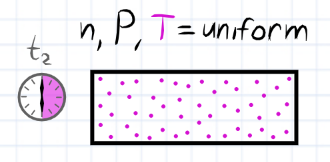
This is certainly not an equilibrium (in general sense) state of the gas. However, we understand that at some moment $t_2$, the system turns to total (or thermodynamic) equilibrium, and particularly to the thermal equilibrium.

But let’s go back to moment $t_1$. What can we say about thermal equilibrium here? I think one can argue that this is thermal equilibrium because temperature is uniform and constant.

By “uniform temperature” I mean that thermal energy is equally shared among all particles, but it is obviously not uniform in volume for the moment $t_1$.

So my question: is thermal equilibrium for a thermodynamic system about thermal energy equally shared among all particles or about thermal energy distributed uniformly in volume?

Thanks everyone who gives their answers or comments in advance.

Answer 1

Yes, you can have equilibrium even if the density is not uniform.

An example of a system you can solve analytically which is in equilibrium while having a non-uniform distribution of particles is the isothermal atmosphere. Because of gravity, it is energetically favorable for particles to fall to the surface of the Earth. However, thermal fluctuations will cause some particles to move upwards through random motion. The balance between these effects leads to a density $\rho$ that changes with height $z$ as \begin{equation} \rho(z) = \rho(0) e^{-z/H} \end{equation} where $H=RT/kM$ ($R$ is the gas constant, $T$ is the temperature, $k$ is Boltzmann's constant, and $M$ is the mass of the particles).

You can view the change in density as being due to a gradient in the chemical potential, caused by gravity.