-2
$\begingroup$

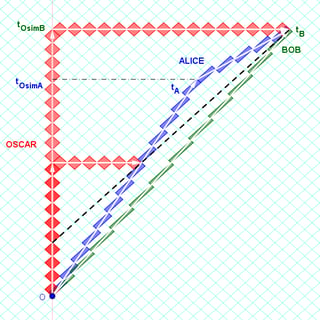
If the laws of physics are the same in all inertial frames why can't we accelerate a particle stationary in its own frame to 0.99c?

Consider a particle that has been accelerated to 0.99c in a particle accelerator in direction d. In the stationary frame of the particle the laws of physics should allow the particle to be accelerated in direction d to 0.99c resulting in a relative speed of 1.98c in direction d from the stationary frame of the particle accelerator.

Improve this question
$\endgroup$
5
  • 6
    $\begingroup$ this is exactly the thought that leads to special relativity! $\endgroup$
    – ACarter
    Commented Jan 16, 2022 at 22:25
  • $\begingroup$ @ACarter well no this appears to cast doubt on the second postulate that the speed of light is a definite value. An observer moving with the particle at 0.99c can claim to be stationary and thus should expect to be able to accelerate the particle from its apparently stationary state to 0.99c relative to his frame. $\endgroup$
    – jamesfairclear
    Commented Jan 16, 2022 at 22:49
  • 2
    $\begingroup$ Does this answer your question? (Almost) double light speed $\endgroup$
    – Bill N
    Commented Jan 17, 2022 at 1:05
  • $\begingroup$ I discuss how velocity in SR is a spacetime angle in this answer: physics.stackexchange.com/a/598415/123208 where I also give a handy way to calculate velocity composition. Using units where $c=1$, the result of combining speeds $\frac{a-1}{a+1}$ and $\frac{b-1}{b+1}$ is $\frac{ab-1}{ab+1}$ $\endgroup$
    – PM 2Ring
    Commented Jan 17, 2022 at 1:18
  • 1
    $\begingroup$ You misunderstand relativity. If you accelerate to 0.99c relative to the Earth, you are now at rest in a new frame, A, say. You can accelerate again to 0.99c relative to frame A, in which case you are now at rest in Frame B. You can then accelerate again to 0.99c in frame B so you are at rest in frame C, and so on ad infinitum. The point is that having accelerated to 0.99c in several separate frame in succession, you will still not have exceeded c in the first frame. Owing to the relativistic law of the addition of velocities, the sum of n speeds of 0.99c is always less than c. $\endgroup$
    – Professor Sushing
    Commented Sep 4 at 12:27

4 Answers 4

Reset to default
6
$\begingroup$

As others have said, the so called "addition of velocities" (better: "composition of velocities") is not additive, as it is in PHY 101.

  • Instead $$V_{CA}=\frac{V_{CB}+V_{BA}}{1+V_{CB}V_{BA}}$$
    https://www.wolframalpha.com/input/?i=B%3D0.99%3B+A%3D0.99%3B+%28B%2BA%29%2F%281%2BB*A%29
    yields 0.999949 for your velocities.

    (This is akin to the fact that slopes of lines don't add. You need a different formula.)

  • However, what does add are "angles" (which are called "rapidities" in relativity) where $V_{CA}=\tanh\theta_{CA}$
    ... so, $$\theta_{CA}=\theta_{CB}+\theta_{BA}$$ and $$\tanh(\theta_{CA})\equiv \tanh( \theta_{CB}+\theta_{BA})\equiv \frac{\tanh\theta_{CB}+\tanh\theta_{BA}}{1+\tanh\theta_{CB}\tanh\theta_{BA}},$$ which is equivalent to the above... but possibly geometrically-simpler to interpret (but may need some practice to accept physically).

    https://www.wolframalpha.com/input/?i=B%3D0.99%3B+A%3D0.99%3B+tanh%28arctanh%28B%29%2Barctanh%28A%29%29
    yields 0.999949 (the same).

  • For small velocities, the result is (approximately) additive... since the denominator is approximately equal to 1 in that case.

UPDATE

  • As @JerrySchirmer points out in an answer, it might puzzling how this formula relates to time and space measurements in a reference frame. So, I'll direct you to an old post Relativistic velocity addition from time dilation which features a "spacetime diagram" (a valuable tool for understanding special relativity).
    robphy-RRGP-velocity

While this situation (counter to everyday experience) may cast doubt on the correctness Special Relativity, there are many experimental tests of Special Relativity and its implications... and it's done quite well over the range of its applicability.

As starting points,
https://math.ucr.edu/home/baez/physics/Relativity/SR/experiments.html
https://en.wikipedia.org/wiki/Tests_of_special_relativity

UPDATE

Prompted by @PM2Ring 's nested doll sequence comment in another answer,
this visualization of mine might be useful for see what happens when one does a nested sequence of equal boosts (equal increments in rapidity, in a regular time interval in the instantaneous frame).
One approaches (but never reaches) the speed of light,
and, in the original (lab) frame, it appears that each increment (naively) appears less effective toward that goal of trying to reach the speed of light.

https://www.desmos.com/calculator/tjngj63cat

robphy-AcceleratedWorldline-desmos-tjngj63cat

Improve this answer
$\endgroup$
3
$\begingroup$

People have answered about how the velocities don't add. As to the why they don't add.

Assume that you have frame A, frame B moving at speed v relative to frame A, and object C moving at speed w relative to frame B.

What is the speed of C in frame A? well, in frame B, the object is moving at a speed w as measured with respect to B's time and space. So, in addition to adding the speed w to to the speed v of frame B, there is also a conversion of B's time and space into A's time and space, which is necessary before you can simply add the two velocities together. Thinking about this carefully using the rules of frame transformations ends up at the rules discussed in the other answers.

Improve this answer
$\endgroup$
10
  • 1
    $\begingroup$ good point. I've updated my answer to point to an old answer featuring a spacetime diagram physics.stackexchange.com/questions/392515/… $\endgroup$
    – robphy
    Commented Jan 17, 2022 at 0:07
  • 1
    $\begingroup$ @jamesfairclear: your intuition here is wrong, because the velocity addition formula is as given in the other answers. Say your observer accelerates the object to $.5c$ in their frame, then, in the earth frame, the objects velocity is: $(0.5c + .99c)/(1 + .5*.99) = 0.996655518395c < c$, precisely because you would be transforming to a frame that is moving so fast relative to earth, and then translating that very warped space and time back into "earth space and time", which is captured in the denominator of the forumla $\endgroup$
    – Zo the Relativist
    Commented Jan 17, 2022 at 21:33
  • 1
    $\begingroup$ @james It's a real speed of 0.99c relative to his frame, which is already moving at 0.99c, relative to the Earth frame. And the amount of energy required for him to accelerate (eg) a proton to 0.99c in his frame is identical to the energy required to accelerate a proton to 0.99c in the Earth frame. $\endgroup$
    – PM 2Ring
    Commented Jan 17, 2022 at 22:26
  • 2
    $\begingroup$ @jamesfairclear: you seem to want to use the earth frame as an absolute frame of reference. That's not how the theory works. There is no absolute speed. All speeds are relative to some frame. Each ship inside the russian doll has the same right to talk about the speed of the object as the Earth does. $\endgroup$
    – Zo the Relativist
    Commented Jan 18, 2022 at 17:11
  • 2
    $\begingroup$ And I'm saying this not as an accusation, but more of a, you seem to keep on coming back to "how fast is it moving, really?" sort of question, and as far as special relativity is concerned, that is a meaningless question. $\endgroup$
    – Zo the Relativist
    Commented Jan 18, 2022 at 17:37
2
$\begingroup$

See https://en.wikipedia.org/wiki/Velocity-addition_formula You're correct up to the point of assuming "resulting in a relative speed of" $.99+.99=1.98$ The original guy still sees the particle moving at $v\lt1.0c$ in his frame of reference, even though the second guy sees it moving at $v=.99c$ in his frame.

Improve this answer
$\endgroup$
6
  • $\begingroup$ but if the speed of the particle does not increase at all in the second guy's particle accelerator then the laws of physics appear to be different in his frame. $\endgroup$
    – jamesfairclear
    Commented Jan 16, 2022 at 22:59
  • 1
    $\begingroup$ The laws of physics do not appear to be different if you consider special relativity to be the governing law. What you are describing is the whole conundrum that special relativity was invented to solve. The older laws of Galileo and Newton are really good approximations of reality when the speeds are much less than c, but they give wrong answers when the speeds are close to c. $\endgroup$
    – Solomon Slow
    Commented Jan 16, 2022 at 23:18
  • $\begingroup$ @jamesfairclear The 2nd guy sees the 1st guy moving (let's call it) "backwards" at $.99c$ and also sees the particle moving "forwards" at $.99c$. And the 1st guy sees the 2nd guy moving "forwards" at $.99c$, and also sees the particle moving "forwards", but at a velocity $.99c\lt v \lt 1.0c$ The particle does gain some additional speed from the point of view of the 1st guy, but never enough so that its velocity is $\ge1.0c$. See the first formula at en.wikipedia.org/wiki/…, whereby the 1st guy sees $v=.999949497c$ for the particle. $\endgroup$
    – eigengrau
    Commented Jan 16, 2022 at 23:28
  • $\begingroup$ @eigengrau 'The 2nd guy sees the 1st guy moving (let's call it) "backwards" at .99c and also sees the particle moving "forwards" at .99c.'. If we assume that the 2nd guy has no reference other than a stationary particle in his frame then would we not expect that when he tries to accelerate it in direction d the particle will barely move relative to him as it is already in motion at 0.99c in direction d? $\endgroup$
    – jamesfairclear
    Commented Jan 17, 2022 at 21:11
  • $\begingroup$ @jamesfairclear You said "a stationary particle in his frame" and then referring to that same particle "is already in motion at 0.99c". It's at rest in his frame, period!!! That's what counts to him, and affects what he sees. The additional fact that the particle's already in motion relative to somebody else's rest frame only affects what that other person sees. James... you haven't been reading/studying/and-or/understanding the answers (mine as well as others) and comments to your question. Everybody's been consistently telling you exactly the same thing. $\endgroup$
    – eigengrau
    Commented Jan 18, 2022 at 5:18
-2
$\begingroup$

You defined the stationary frame to be the frame moving at .99c to the lab frame. If you also define the lab frame to be stationary you have built a logical contradiction into your premise and no conclusion can be valid.

For how to actually work with comparing relative velocities between frames, research "relativistic velocity addition".

Improve this answer
$\endgroup$
2
  • $\begingroup$ @g s I don't see any contradiction. An observer moving with the particle at 0.99c can claim to be stationary and thus should expect to be able to accelerate the particle from its apparently stationary state to 0.99c relative to his frame. $\endgroup$
    – jamesfairclear
    Commented Jan 16, 2022 at 22:44
  • $\begingroup$ $\vec v_1=0$ and $\vec v_2=0$ and $|\vec v_1 - \vec v_2| = 0.99c$ $\endgroup$
    – g s
    Commented Jan 17, 2022 at 0:39

Not the answer you're looking for? Browse other questions tagged or ask your own question.