0
$\begingroup$

I know there are some similar questions but I want to know why a specific idea doesn't work. I'm considering a ball or a cylinder rolling without sliding on a plane. The plane applies a frictional force to make it spin. 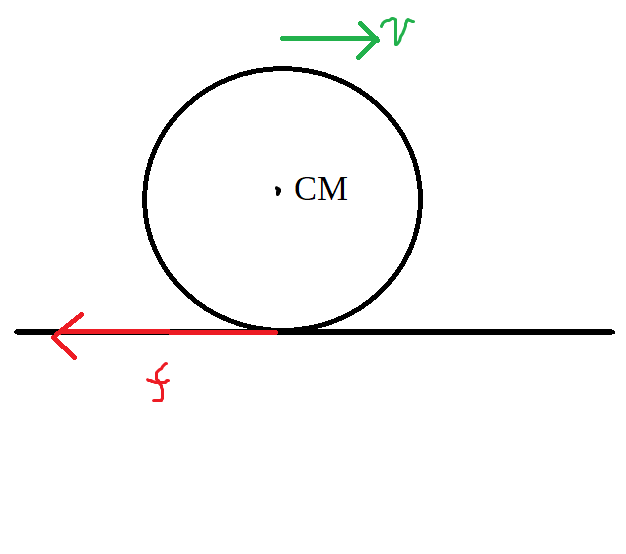enter image description here

Now, the question is, to analyze it we do the sum of forces = Ma, so $-\vec{f}=m\vec{a}_{CM}$ and from angular momentum formula with pole in the CM: $I_{CM}\vec{\alpha} = \vec{R}x\vec{f}$. From both this equations we get that there should be a deceleration.

Analyzing all this from an energetic point of view we obviously get that the work done by friction is 0, because the contact point is "still", thus no deceleration should occur. Not only this but if we put the pole in the point of contact we don't have any torque, thus also concluding that no deceleration should be happening.

Where is the first approach wrong? What assumption am I making that breaks everything?

Improve this question
$\endgroup$
4
  • $\begingroup$ It seems to me that the acceleration of the contact point on the cylinder (due to its rotation) is radial, whereas your force vector 'f' is tangential; so the vector product is zero - as indeed is the magnitude of 'f'. $\endgroup$
    – Martin CR
    Commented Jan 9, 2022 at 19:45
  • $\begingroup$ Or were you considering the initial state in which the cylinder has an initial linear velocity but no rotation? $\endgroup$
    – Martin CR
    Commented Jan 9, 2022 at 19:47
  • $\begingroup$ I'm assuming a cylinder rolling without slipping. Btw the vector product should be Rf since they're perpendicular, and f can't be 0 since otherwise the motion would be a cylinder sliding with no rolling. $\endgroup$
    – sonod4
    Commented Jan 9, 2022 at 19:52
  • $\begingroup$ is the cylinder rotating at a constant rate? If so, unless I've misunderstood, I would say that that the tangential component of 'f' would be zero $\endgroup$
    – Martin CR
    Commented Jan 9, 2022 at 20:01

1 Answer 1

Reset to default
1
$\begingroup$

The assumption you are making that breaks everything is that static friction is required for the ball or cylinder to freely roll at constant velocity on a horizontal surface without sliding. It is not.

The friction force you show would only be required to start the ball or cylinder rolling backwards without sliding from rest, for example in response to a counter clockwise torque initially applied about the COM to start it rolling backwards, or to decelerate the ball or cylinder that is rolling forwards, as you found out in your analysis. Once it starts rolling, no static friction is required to keep it rolling without sliding, assuming there are no externally applied forces.

Hope this helps.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Yes, thank you so much, I did so many exercises where it was required that I couldn't picture it without friction, but it actually makes a lot of sense thanks. $\endgroup$
    – sonod4
    Commented Jan 9, 2022 at 20:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.