Why do we hear louder sound when the source is in a resonator?

Question

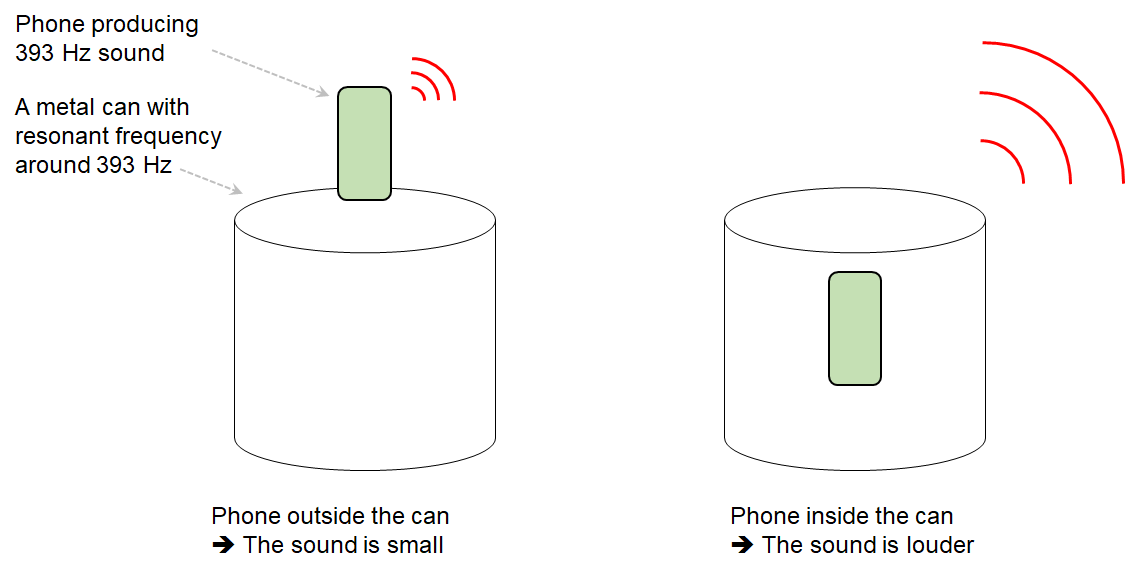
I did a simple experiment as illustrated below

Basically, I used an app to let my phone produce a 393Hz sine wave sound. This is roughly the resonant frequency of a metal can. When the phone is outside the can, the sound is not large. While when I put the phone into the can, the sound becomes noticeably louder. I used a second phone to measure the spectrum of the sound, and the recording indicates a 15dB increase in magnitude. At the link below you may find a video recording of the experiment (though the quality of the video is not satisfactory).

https://drive.google.com/file/d/1Ku4a-kpM1ef6Ke1ivNwOzsGZCA7q8BNL/view?usp=sharing

Here is the question: the phone has a constant power, which means the sound source are producing a fixed amount of energy per unit time. Then, why do we hear louder sound when the phone is inside the can? From the conservation of energy, shouldn't the total sound power remain constant? Obviously the can cannot produce energy by itself, so where does the extra power come from?

Also, the louder sound can be heard from almost any direction, from top or from side, which means that the metal can did not attempt to focus the sound toward specific directions.

Thanks for any comment.

Answer 1

The phone and the speaker may have constant power, but it cannot deliver all of the power into the air. Some of the power just stays in the phone and is lost as vibrational heat.

Wave energy is not able to efficiently move from one medium to another when the propagation properties are very dissimilar (Impedance Matching). Instead the energy reflects at the interface. In this example, the energy reflects back into the phone instead of being transmitted into the air.

I don't know how you did your experiment, but if the phone and the can were both sitting on a table, that solid surface might have been able to send much more of the energy into the can, and the can was able to couple more efficiently to the air to make a nice speaker.

Even without a solid contact between the phone and the can, the resonant cavity might have been able to couple to the phone more efficiently than the open room did.