3
$\begingroup$

I've just finished studying for an exam on the Standard Model (so electroweak theory and symmetry breaking) and I can't figure out how this question never crossed my mind. I'm now studying the QCD chiral symmetry breaking, but I think my question applies to any (physical) SSB.

I know what SSB is (symmetry of the Lagrangian but not of the states) and I also know how one implements it in a theory (scalar sector with a mexican-hat potential) and it's clear to me the implications of the two different phases, the broken one and the restored one (vev for the nonbroken scalars, Goldstone bosons eventually "eaten" by the gauge bosons).
What I don't understand is, how does the phase transition work? How did the universe go from one phase to the other?
Does the potential just "switch on"? Is it always on but at high energies the quantum/thermal fluctuations don't "see" its structure?

Improve this question
$\endgroup$
10
  • 3
    $\begingroup$ Suppose the only SSB you knew about, rather than Higgs, was an upright pen falling in a random direction when you take your finger off it. This has a $U(1)$ symmetry, like the Higgs found in scalar electrodynamics. We can't predict or explain that direction, but it's obvious that the SSB is due to reducing potential, like so much dynamics. $\endgroup$
    – J.G.
    Commented Dec 21, 2021 at 14:27
  • 2
    $\begingroup$ Yes, there is an entire field of thermal QFT... $\endgroup$
    – Cosmas Zachos
    Commented Dec 21, 2021 at 15:24
  • 2
    $\begingroup$ This is a mother paper, but perhaps too rich for an intro... Try this. $\endgroup$
    – Cosmas Zachos
    Commented Dec 21, 2021 at 15:31
  • 2
    $\begingroup$ @Lunaron That looks like the beginning of an answer, not a comment - please consider writing an answer. $\endgroup$
    – ACuriousMind ♦
    Commented Dec 21, 2021 at 15:37
  • 2
    $\begingroup$ @CosmasZachos Likewise - please consider writing an answer. $\endgroup$
    – ACuriousMind ♦
    Commented Dec 21, 2021 at 15:37

2 Answers 2

Reset to default
1
$\begingroup$

I'm just sketching the trail map of where your question might wish to go... it is a subject of limitless complexity.

The universe cools down, and thermal QFT dictates mutation of the Higgs potential with temperature, section 3.

This mutation of the effective Higgs potential from the form favoring the symmetric phase to one favoring SSB at lower temperature describes when the phase transition is likely to switch on, at $T_c$.

enter image description here

Improve this answer
$\endgroup$
7
  • 2
    $\begingroup$ I'm baffled by the fact that you often seek "where the question might wish to go". Your answer is a perfectly good answer for a question that doesn't really want to go anywhere: I didn't know that the temperature changed the form of the potential, now I do. Thanks! $\endgroup$
    – Mauro Giliberti
    Commented Dec 21, 2021 at 16:03
  • 1
    $\begingroup$ In the early universe, which is what the OP suggests, the transition was thermal. This may be not quite an academic question…. $\endgroup$
    – Cosmas Zachos
    Commented Dec 22, 2021 at 0:06
  • $\begingroup$ Original posting. It is the accepted theory of the evolution of the universe. Mainstream physics need not be argued for ab initio. $\endgroup$
    – Cosmas Zachos
    Commented Dec 22, 2021 at 13:02
  • $\begingroup$ I am not a cosmologist, but the accepted mainstream scenario certainly monitors thermal evolution, from the Wigner to the Nambu mode realization. Coleman - Weinberg has lost out for decades, and its demise merits a separate question. $\endgroup$
    – Cosmas Zachos
    Commented Dec 31, 2021 at 2:32
  • $\begingroup$ The phenomenology of the mainstream thermal picture works out pretty well. $\endgroup$
    – Cosmas Zachos
    Commented Dec 31, 2021 at 3:13
1
$\begingroup$

Good question.

Well in any QFT, the origin of SSB can be traced back to the loop Quantum corrections as was pointed out beautifully by Sidney Coleman and Eric Weinberg for the first time.

The analogy with solid state physics is helpful but not precisely and (necessarily) what takes place in QFT.

There are several variants of SSB, Global, Local, Chiral, Dynamical etc. But in all these cases SSB is due to quantum fluctuations.(At least at the level of Peskin Schroeder which is all written at zero temperature)

The effective action formalism(used for the first time by Jona Lasinio in the context of QFT) can shed enormous light on the physics of SSB in QFT as makes the solid state analogy even closer to real while one should keep in mind, SSB in QFT happens at zero temperature(though one can extend it to finite temperature QFT in a more advanced level) so the anology with solid state shouldn't be taken literally, and the role of temperature in QFT is basically played by a controlling parameter like vacuum expectation value of a Quantum Field(say scalar).

The following paper might help:

EDIT:

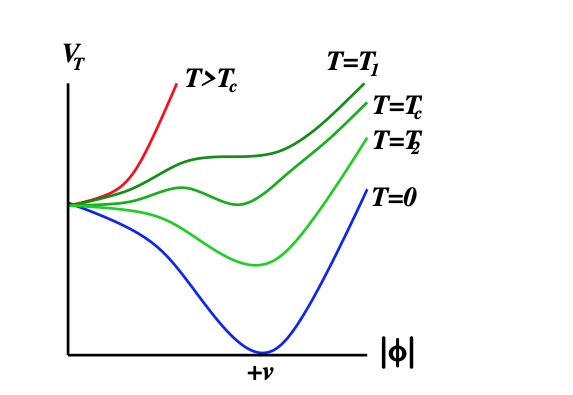
In fact "why the initial state is at the tip of the Mexican hat" can be answered this way in my opinion:

I'd say perhaps the question can be answered from a more diplomatic point of view. One can assume the initial temperature is so high that quantum fluctuations are dominated by thermal fluctuations and one has a prabolic potential, and this preseves the vacuum at the bottom of the well, and as the temperature decreases then at a certain point the quantum fluctuations can dominate the statistical ones, where the old vacuum tunnels into the new one.[In fact it can be even more complicated, the potential might not be necessarily parabolic but that "suffices" to make my point]

The first regime is where $kT>ℏω$ and the second one is where $kT<ℏω$. And all this is to avoid nonsensical negative mass terms needed for thermal phase transition! That's the root cause of my objection to thermal phase transition as the ultimate proposal for SSB in particle physics!

Improve this answer
$\endgroup$
12
  • 2
    $\begingroup$ Suppose you've got a ball at the minimum of a potential that fulfills the original symmetry of the theory. This is quite classical. What happens is that if you add loop corrections to the classical potential(a perturbative series in Planck constant), then the shape of the potential changes to that of a mexican hat! Then, the ball that is steady at the local maximum of the new potential undergoes Quantum Tunneling and moves to the absolute minimum of the Quantum potential! This is how Quantum theory leads to phase transition. It's called Quantum phase transition at zero temperature. $\endgroup$
    – Bastam Tajik
    Commented Dec 21, 2021 at 19:12
  • 1
    $\begingroup$ In solid state physics the controlling parameter is the temperature. But in QFT the controlling parameter(order parameter) is the vacuum expectation value of the field that can be even space-time dependent in general and even break the translational invariance. $\endgroup$
    – Bastam Tajik
    Commented Dec 21, 2021 at 19:14
  • 1
    $\begingroup$ that's a lot more clear, the fact that quantum loop corrections reshape the potential. One last thing though: a quantum correction doesn't happen, it isn't an event, it's a correction of something that already there. But the SSB happens, meaning that the early universe underwent a reshaping of the scalar potential. What happened there? The currently accepted answer seems to say that "a drop in temperature happened" and I found that satisfying. What's the "quantum fluctuations" version of this? $\endgroup$
    – Mauro Giliberti
    Commented Dec 21, 2021 at 19:24
  • 2
    $\begingroup$ but why was the ball at the local maximum? Why is there a "breaking" and not just a broken case to start with? $\endgroup$
    – Mauro Giliberti
    Commented Dec 22, 2021 at 12:48
  • 1
    $\begingroup$ Thanks. As far as I remember(I studied particle physics 5 years ago!), the vacuum field configuration can be anything in the potential well theoretically speaking. One must bear in mind, that the experimental evidence says that light is massless, so Weinberg chose a particular direction for the Higgs field to maintain this, though one can rotate that vacuum arbitrarily and make massive photons, as negligible as one might will! But I'm not sure if unitarity sets any upper bound on the photon masses or not(just like how it sets an upper bound on the Higgs mass). @James $\endgroup$
    – Bastam Tajik
    Commented Oct 23 at 20:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.