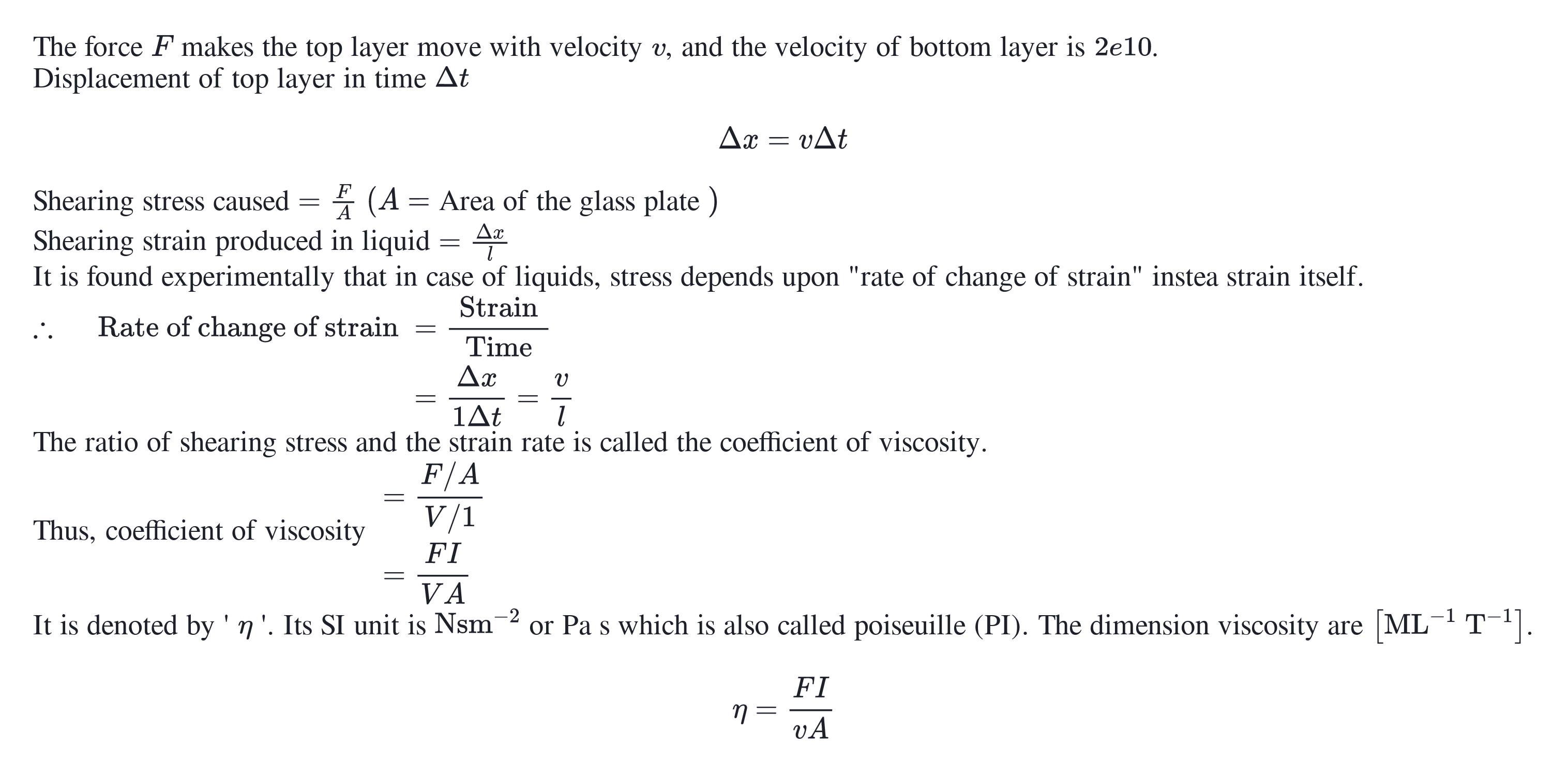Coefficient of viscosity $\eta=\frac{F l}{v A}$

Question

The coefficient of viscosity is described in my textbook as the ratio of shearing stress to strain rate. However, I am unable to understand how the coefficient of viscosity is derived here based on the aforementioned definition. I wanted to check with Stack Exchange to see if both the definition and the derivation is valid and if it follows the strokes law.

The ratio of shearing stress and the strain rate is called the coefficient of viscosity. Thus, coefficient of viscosity $$\eta=\frac{F/A}{v/l}$$ $$\eta=\frac{F l}{v A}$$

The actual derivation stated in my textbook has me stumped. In the case of liquids, the textbook says that stress is determined by "the rate of change of strain" rather than the strain itself.

P.S. $\eta$ = coefficient of viscosity, $F$ = Viscous force, /= Separation between two lamina, $A$ = Area of each lamina, $v$ = Relative velocity of two lamina

Answer 1

(a) Imagine that you are applying a shearing stress to a cuboid of foam rubber resting on a table, using the force applied to the bottom of the cuboid by the table and the force applied to the top of the cuboid by the flat of your hand. [Aside: The situation is more complicated than just a pair of equal and opposite horizontal forces, as these would cause the cuboid to rotate. In fact you would (unconsciously perhaps) also apply vertical forces to make an opposing couple, which would stop the rotation, but still leave the shearing stress. We don't need to go further into this, as your worry seems to be about strain, not stress.] The result of applying the stress to the cuboid is that it deforms, reaching a final or equilibrium deformation. This is measured by the shearing strain. The deformation is elastic, disappearing when we remove the stress.

Now imagine that instead of the cuboid, you have a pile of paper – several hundred sheets, perhaps. If you apply enough shearing stress, pieces of paper will start to slip over each other, and we get a continually increasing non-elastic shearing strain.

What happens in a liquid subject to a shearing stress is a little like what happens in the pile of paper. Layers of liquid start to slide over each other. This is because the bonds between liquid molecules are such that a molecule continually changes its neighbours in adjacent layers. Applied stress favours neighbour swaps that relieve the stress. The rate at which this neighbour-exchange happens in such a way as to cause sliding of layers is roughly proportional to the shearing stress applied. So strain $=x/l$ is no use for a liquid because $x$ keeps changing even when the stress is applied is constant. Instead we use rate of (change of) strain $=v/l$, because $v/l$ is constant when a constant stress is applied.

(b) Is "the strokes law" George Gabriel Stokes's law about the resistive force on a sphere moving through a fluid under streamline conditions? If so, the law is indeed derived using the definition of viscosity, $\eta$, that you have given. The derivation is not elementary.