Intensity in function of distance of a flashlight

Question

any of you may be familiar with the inverse square law for a spherical wave. The thing is that I did the experimental measurements and I was shocked to find out that the data doesn't behave that way. Actually, it fits exceptionally well to a decreasing exponential. I was thinking that the reason for this is that a flashlight isn't a punctual light source, then the light doesn't behave as a spherical wave. Also, the flashlight gathers light inside a cone, where we can spect some interference.

I searched online for a solution for this problem and dint find why it is that behaves exponentially. I think it could maybe be derived from an inhomogeneous wave equation but haven't been successful in finding that exponential behavior.

Does someone know how to derive these, or why this happens? 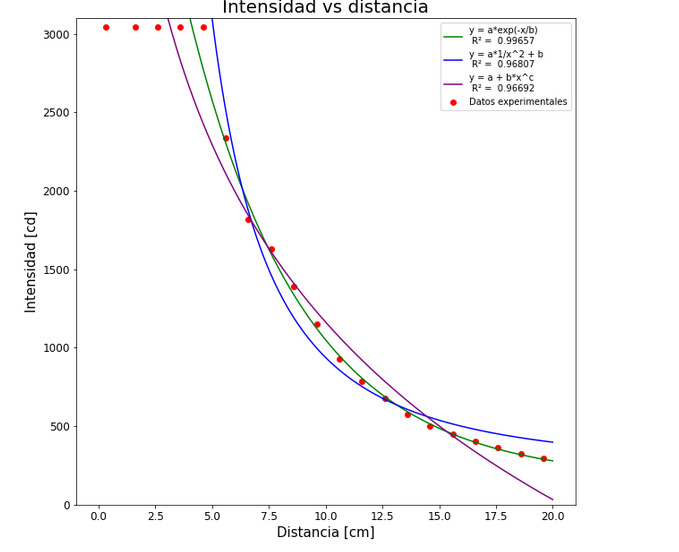Intensity vs distance plot of experimental data with some different fits. The first 5 points can be ignored since the sensor was saturated.

EDIT

Thanks to all of the responses I managed to solve the mystery. It turns out that indeed the sensor was saturated. I did the measurements for a range of 20 - 90 cm and it fitted perfectly 1/r^2. Then, I did the measurements in a range of 8 - 20 cm with a linear polarizer on the front to reduce light intensity in half and also fitted very well. Then, it Was the sensor's fault. I made sure that light wasn't polarized at the start. And yes, the flashlight was shining through air.

Answer 1

Consider the case of a flashlight consisting of a point source of light located in front of a spherical or parabolic reflector.

We then have effectively two light sources to consider

The first involves the light emitted by the point source going forward from the flashlight towards the detector. The contribution of this light to the overall intensity at the detector would have an inverse square variation with distance, with the distance measured from the actual light source.

The second contribution comes from the light emitted toward the reflector. This light will form a real or virtual image of the light source at a location depending on the position of the real light source and the focal length of the spherical/parabolic reflector. It is the distance from this location that should be used in the inverse-square formula to find the drop off in intensity.

For example, if the light source is located at the focal point of the reflector, the result would be a virtual image of the light source at infinity. with effectively no drop off in intensity between the source and the detector.

Or consider if the flashlight is focused, by moving the light source relative to the reflector, to form a real image of the light source in front of the flashlight. Trying to measure the intensity at that point could lead to a fried detector!

Answer 2

First of all, if you just look at the intensities at 10 cm and 20 cm, the latter appears to be pretty close to 1/4 of the former, so what you would expect from a $1/r^2$ law when doubling the distance. So there is certainly nothing wrong with physics here.

Now a $1/r^2$ law would only exactly apply for a point source, but you indicated yourself that the flashlight has some extension. This would obviously lead to a different behaviour in the near zone. If, for the sake of the argument, you model the flashlight by a disk of radius R rather a point you will an get r-dependence of the intensity like that of the field of a charged disk. So you should try to fit the data to the following equation

$$y=b+a\cdot (1-\frac{x}{\sqrt{c^2+x^2}})$$

where c characterizes the effective size of the light source.

For $c<<x$ (point source) this formula reduces to a $1/r^2$ dependence.

Anyway, why don't you put a filter over the flashlight to reduce the intensity? This would prevent the detector getting saturated in the near zone and thus expand your usable range.