A "Bohr orbit" is related to a classical orbit via the correspondence principle, but not all sets of quantum numbers for hydrogen-like atoms correspond to Bohr orbits. Quantum mechanics is richer than Bohr initially imagined.
Let's consider a hydrogen-like atom with quantum numbers $(n,\ell,m)$. The radial part of the wavefunction is
$$
R_{n\ell}(r) = \sqrt{\text{stuff}}\times e^{-u/2} u^\ell L_{n-\ell-1}^{2\ell+1}(u)
\quad\quad
\text{where } u = \frac{2Zr}{n a}
$$
where $r$ is the radial coordinate, $Z$ the nuclear charge, and $a$ the Bohr radius.
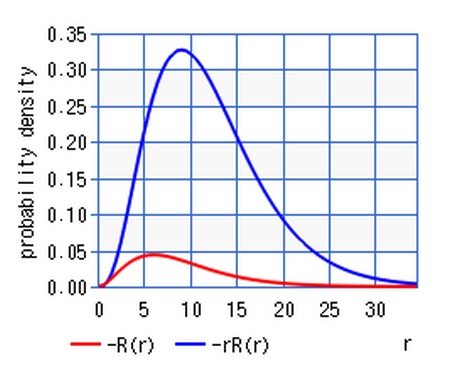
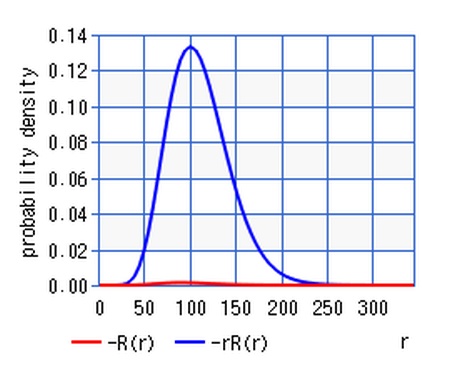
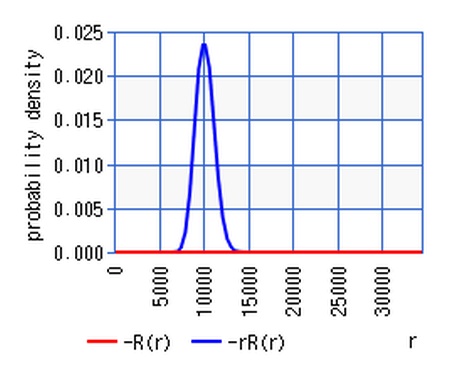
The $L_\alpha^\beta$ are the associated Laguerre polynomials, which are polynomials of order $\alpha$. So for an orbital with maximal angular momentum $l=n-1$, the Laguerre stuff is just a constant, and the radial wavefunction $R\sim e^{-r} r^\ell$ has a zero at the origin (for nonzero $\ell$) and a single maximum at some finite $r$. For large $n,\ell$ this single peak is narrow and it makes sense to think of the electron as being "radially localized," like a particle in a circular orbit.
Similarly, the spherical harmonics are given by an associated Legendre polynomial in the variable $\cos\theta$, multiplied by an azimuthal phase $e^{im\phi}$. The extremal Legendre polynomials all have the form
\begin{align}
P_\ell^\ell (x) &= (\text{constant}) (1-x^2)^{\ell/2}
\\
P_\ell^\ell (\cos\theta) &= (\text{constant}) \sin^{\ell}\theta
\end{align}
So for a hydrogen electron where the angular momentum projection is maximized, $|m|=\ell$, the angular probability distribution is strongly peaked at the equator; that peak gets narrower for larger $\ell$. The complex phase increases linearly as you go around in $\phi$.
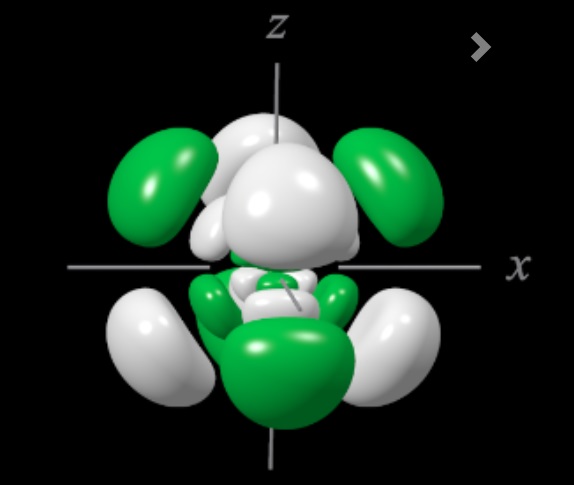
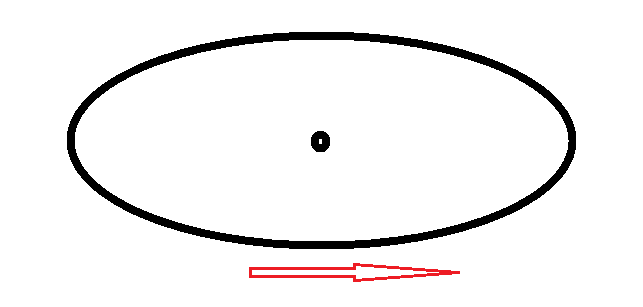
Taken together, an electron in a hydrogen-like orbital with maximum angular momentum and maximum angular momentum projection onto the $z$-axis, with quantum numbers $(n,\ell,m) = (\ell+1,\ell,\pm\ell)$, has its probability concentrated in a narrow ring around the equator of the coordinate system, with the phase changing around the ring.
If you include the time-dependent part of the wavefunction, multiplying by $e^{-i\omega t}$, you have a phase change with time which you can use to find a "probability current." This is the Schrodinger version of a Bohr orbit.
If $\ell$ is large enough that the Bohr orbit is a good description, then the atom can in fact radiate —— not continuously, but by emitting photons and going to orbits with smaller $n$, and eventually to the ground state.
Beware of visualizations like the orbitron, linked in your post. For the case of the $p$-orbitals ($\ell=1$), chemists like to refer to the spatially-oriented $p_x$ and $p_y$, which are rotated versions of $p_z$. However, $p_z$ has definite $m=0$; the $m=\pm1$ states are linear combinations of $p_x \pm i p_y$. For the higher $\ell$, there are more choices to make. I think the chemists' approach is to combine wavefunctions with equal-magnitude $m$, so that the combined wavefunctions are real.