0
$\begingroup$

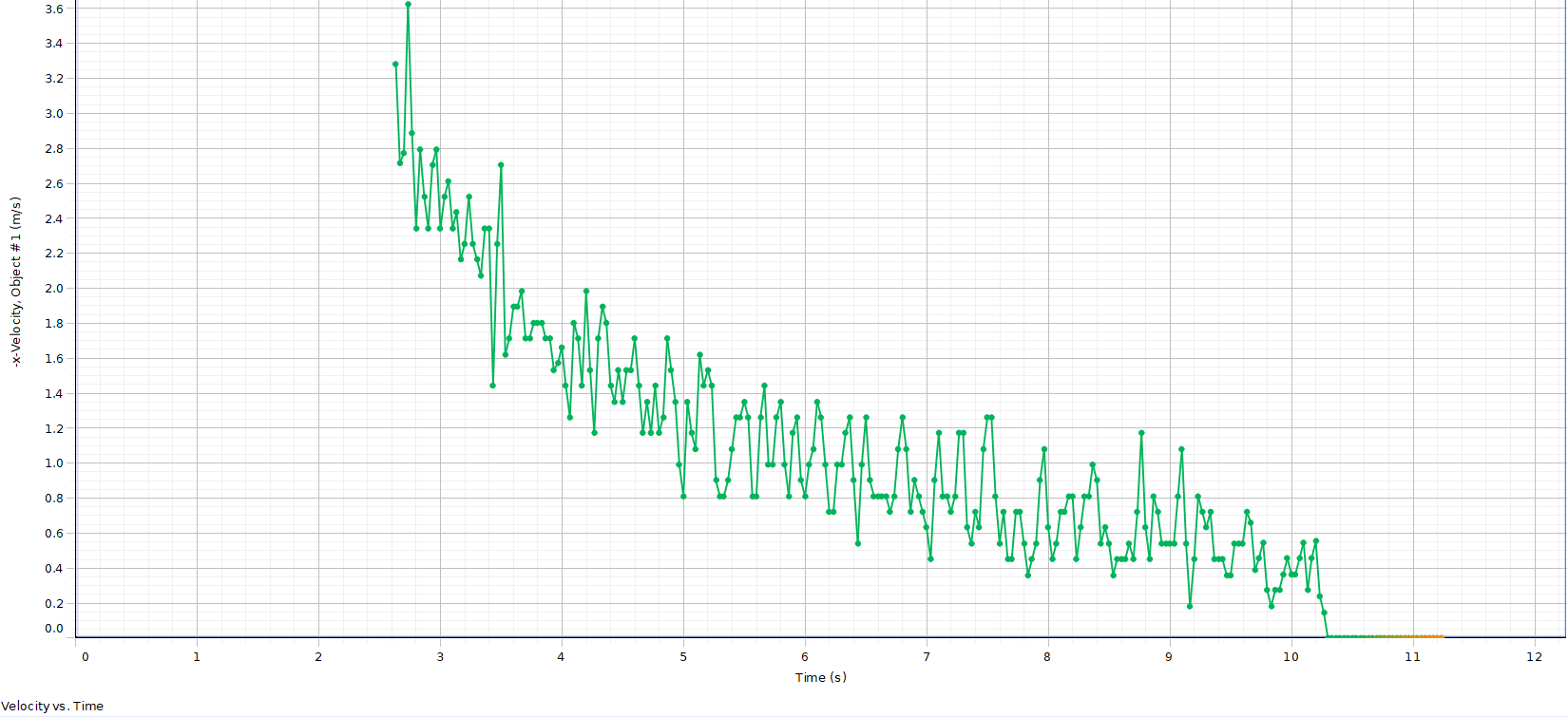
I took a video of a swimmer pushing off of the wall and gliding through the water until they come to a stop. Using some video analysis software, I was able to plot data points for their velocity over time. enter image description here

I would like to fit this data to a curve, but am not sure what type of relationship it should be. I know that the only acceleration that the swimmer experiences is from drag, which is modeled by the equation $$F=\frac{1}{2}\rho v^{2}C_dA$$ Where $\rho$=density of the water, v=velocity of the swimmer relative to the water, $C_d$=drag coefficient, and A=reference area. This suggests that acceleration (since F=ma) is proportional to $v^2$. However, according to the SUVAT equations, $$v=u+at$$ Where u=initial velocity. I'm not too sure how to continue deriving the relationship between v and t. I'm thinking it could be exponential, inverse, or inverse squared, but I don't really have any reasoning behind those guesses.

Improve this question
$\endgroup$
1
  • $\begingroup$ The drag formula can get a lot worse if you consider planing. I doubt a human body planes to any measurable amount, but certainly something like, say, a windsurf board planes at high speed and then enters into the standard drag formula as it slows and sinks. $\endgroup$
    – Carl Witthoft
    Commented Nov 23, 2021 at 17:55

1 Answer 1

Reset to default
1
$\begingroup$

The equation $v=u+at$ is only valid for constant acceleration. To go ahead $$\begin{aligned} F&=ma \\\implies\frac{1}2\rho v^2C_dA &=m\dot v \end{aligned}$$ The above equation can be solved for the velocity as a function of time.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.