Consider a loop (or a hoop) rotating perpendicularly to it's symmetry axis. The loop flattens. It is a model of Earth flattening.
https://www.youtube.com/watch?v=Tctr8CIMOZA&ab_channel=UMDemoLab
The usual explanation is that there is a centrifugal force acting. This is however, a description of in the rotating system. What would a description in the inertial frame? There is a centripetal force, which is the result of which forces? With many thanks.
You can think about what a little "chunk" of the Earth is doing as it's rotating around the axis. Instantaneously this "chunk" has some momentum upwards, and it would like to keep that momentum upwards. However, a centripetal force (which is "real") is pulling this chunk radially inwards, with the net effect of it rotating. But it will pull back on whatever is causing the centripetal force because of its initial momentum. In other words the chunk will try to instantaneously pull away from the rest of the Earth, hence the flattening.
It is usually easier to work in the rotating frame, but you have to be careful because now our "chunk" has no initial momentum. In order to reproduce the above "pulling" effect, we introduce a "fictitious" centrifugal force acting radially outwards. This force doesn't exist in the inertial frame, rather it is just a way for us to account for conservation of momentum when working in non-inertial frames.
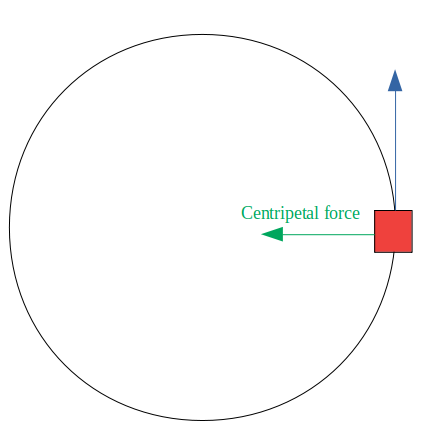
If something rotates in a circle there has to be a force acting on it. If it weren't it would fly away in a straight line. The metal strip is rotating so which force keeps it from flying away? In this case the tension from the metal strip provides the necessary centripetal force.
The force needed to rotate at a certain radius (and speed) is given by $$F_{\text{centripetal}}=\frac{m v^2}{r}$$ So how does this relate to your question? The parts of the metal strip want to move in a straight line so when the strip is rotating the parts want to spread out. Take a look at this picture:
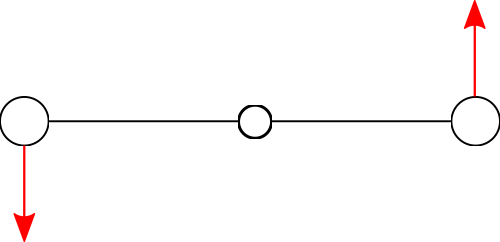
If the balls were to follow their velocity vector (red arrow) they would fly away from the center. Again, to prevent this you need centripetal force. The further out you the more centripetal force you need to stay on your circle. The parts on the metal strip that are furthest out need the largest force but the tension of the strip can only give so much. Another way to see this would be consider a couple ideal springs each having force $F=-k(r-r_0)$ attached to a rotating axis like this:
Here $v=\omega r$ and using that it is easy to show that in equilibrium the length is proportional to the resting length of the spring i.e. $r\propto r_0$. The springs provide the necessary centripetal acceleration and the further away you are from the center the more force you need. In practice this often means you get flung out further.
