Application of Bernoulli equation to pipe with pump

Question

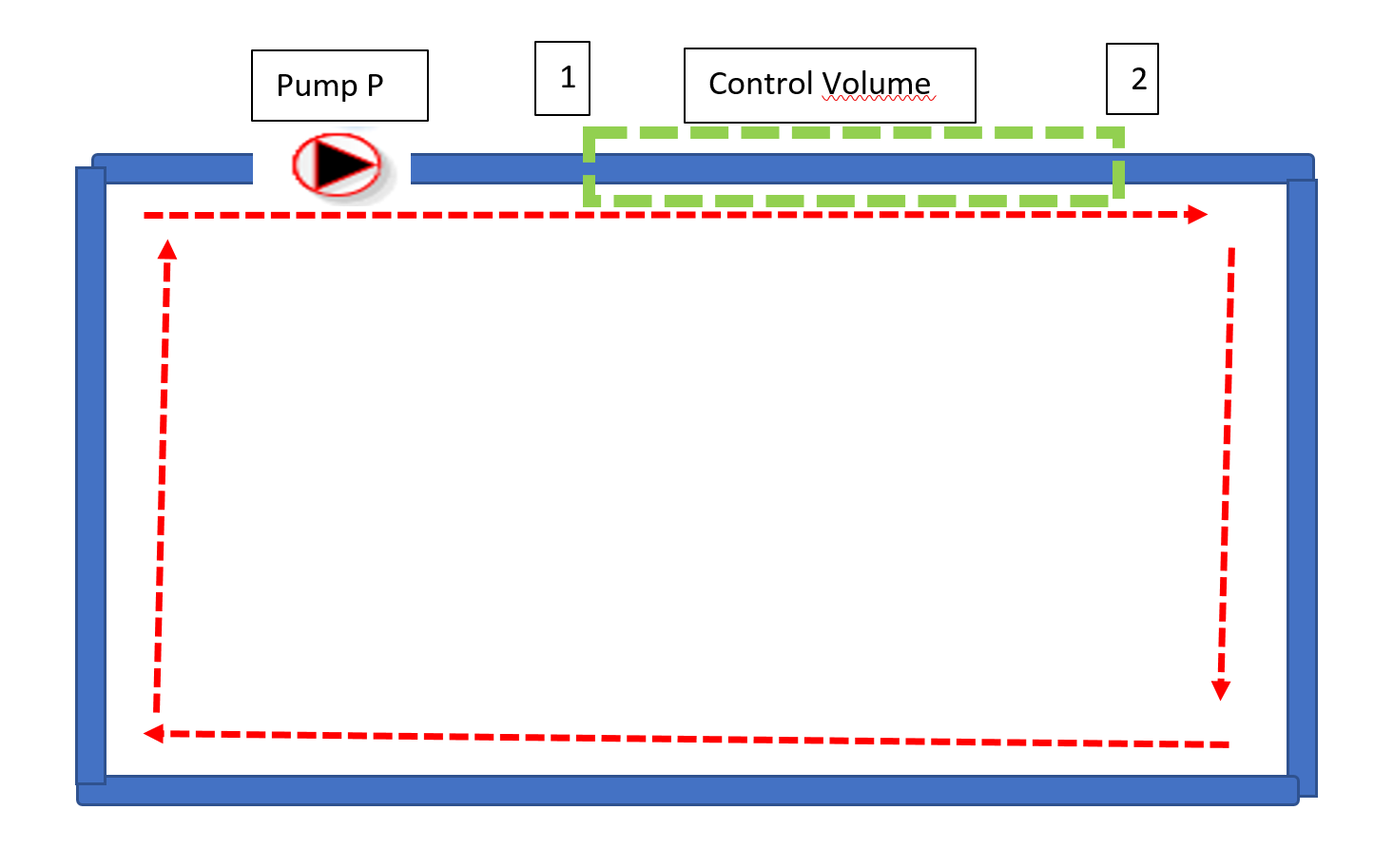
I am currently trying hard to understand the application of Bernoulli's equation to describe flow generated in a closed circuit driven by a centrifugal pump

Below is closed circuit circulating water. The circulation is maintained by a centrifugal pump. I am assuming the flow to be incompressible. Then I take a random control volume marked by green boundary, somewhere downstream of the pump

Now I try to apply the bernoulli's equation to explain the flow across the control volume. Since, the gravitation acceleration, elevation, density and velocity in the streamline is same at the entry and exit of the control volume,

$$\frac{v_1^2}{2} + gz_1 + \frac{p_1}{\rho} = \frac{v_2^2}{2} + gz_2 + \frac{p_2}{\rho} $$

$v_1 = v_2, z_1 = z_2$

When solving the above equation, we get $ p_1 = p_2 $

If the pressures are equal then how is the flow happening through the control volume. I know I am thinking in a totally wrong way, and would be very glad if I get some explanations regarding the above phenomenon

Please bear with me as I have lost touch with high school physics a long time ago, so it could be nice if the explanations are bit detailed

Answer 1

You are missing a head loss term from the friction in the pipe. The downstream pressure will be slightly less than the upstream pressure because of this.

Answer 2

Bernoulli assumes not only incompressible but also inviscid fluids.

But real fluids do show viscosity and thus there's pressure loss in the pipes and the sudden bends, due to viscous losses.

Ignoring the latter leads to $p_1=p_2$, which can be considered a crude approximation.

Answer 3

You are looking at the system at steady-state after the flow has established. In the absence of measurable loss in this section, then pressure differences create accelerations, not flow.

When the pump starts up, $p_1 > p_2$, so the fluid in that section increases speed. After the system reaches steady-state, then the pressure difference is only needed to overcome loss in that section. In the limit, if there is minimal loss, the pressure at each end may not be measurably different.

But that is okay. That just means the velocity of the flow doesn't change in that section. It enters with velocity $v_1$ and exits with velocity $v_1$. No pressure difference, no acceleration.